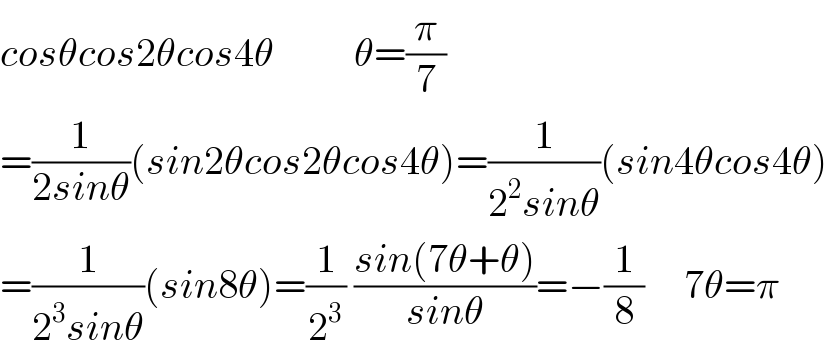please-help-cos-pi-7-cos-2pi-7-cos-4pi-7-

Question Number 117552 by sandy_delta last updated on 12/Oct/20

please help

\cos \frac{π}{7} . \cos \frac{2 π}{7} . \cos \frac{4 π}{7} = ?

Commented by TANMAY PANACEA last updated on 12/Oct/20

f i n d c o s \frac{π}{7} + c o s \frac{2 π}{7} + c o s \frac{4 π}{7} p l s

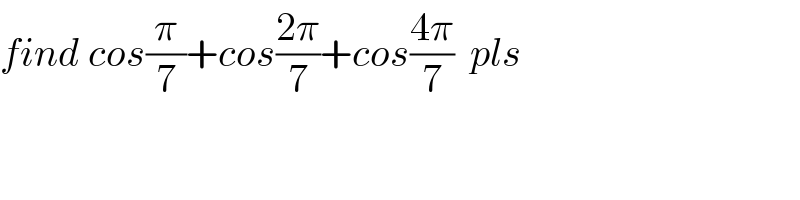
Answered by AbduraufKodiriy last updated on 12/Oct/20

\frac{s i n \frac{π}{7} c o s \frac{π}{7} c o s \frac{2 π}{7} c o s \frac{4 π}{7}}{s i n \frac{π}{7}} = \frac{s i n \frac{2 π}{7} c o s \frac{2 π}{7} c o s \frac{4 π}{7}}{2 s i n \frac{π}{7}} =

= \frac{s i n \frac{4 π}{7} c o s \frac{4 π}{7}}{4 s i n \frac{π}{7}} = \frac{s i n \frac{8 π}{7}}{8 s i n \frac{π}{7}} = - \frac{s i n \frac{π}{7}}{8 s i n \frac{π}{7}} = - \frac{1}{8}

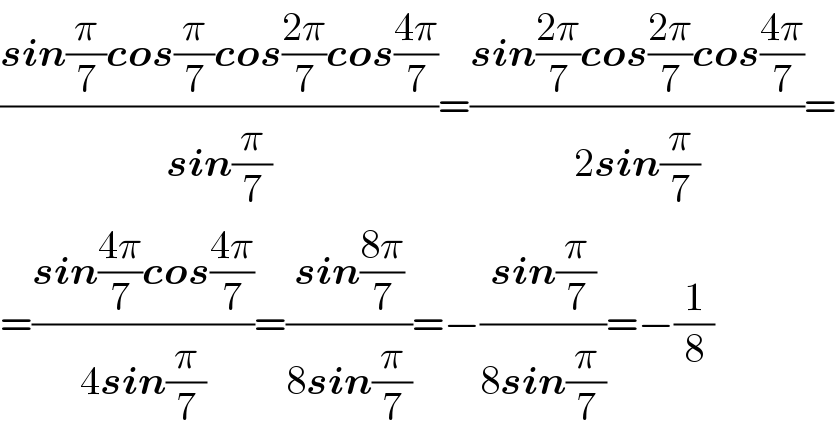
Commented by sandy_delta last updated on 12/Oct/20

thank you sir

Answered by Dwaipayan Shikari last updated on 12/Oct/20

c o s θ c o s 2 θ c o s 4 θ θ = \frac{π}{7}

= \frac{1}{2 s i n θ} (s i n 2 θ c o s 2 θ c o s 4 θ) = \frac{1}{2^{2} s i n θ} (s i n 4 θ c o s 4 θ)

= \frac{1}{2^{3} s i n θ} (s i n 8 θ) = \frac{1}{2^{3}} \frac{s i n (7 θ + θ)}{s i n θ} = - \frac{1}{8} 7 θ = π