Question Number 101921 by Farruxjano last updated on 05/Jul/20
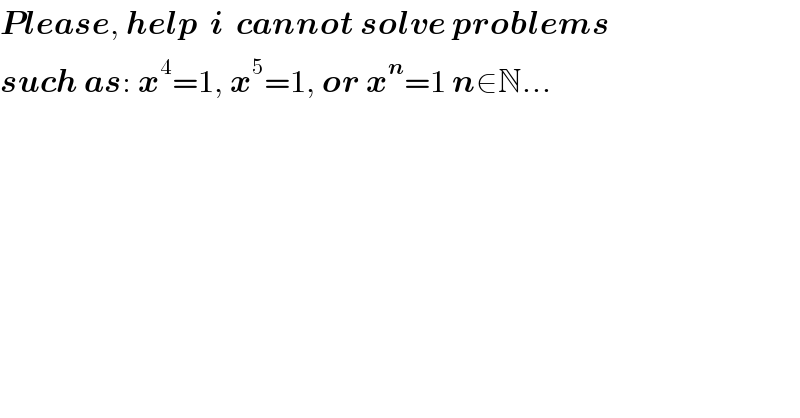
$$\boldsymbol{{Please}},\:\boldsymbol{{help}}\:\:\boldsymbol{{i}}\:\:\boldsymbol{{cannot}}\:\boldsymbol{{solve}}\:\boldsymbol{{problems}} \\ $$$$\boldsymbol{{such}}\:\boldsymbol{{as}}:\:\boldsymbol{{x}}^{\mathrm{4}} =\mathrm{1},\:\boldsymbol{{x}}^{\mathrm{5}} =\mathrm{1},\:\boldsymbol{{or}}\:\boldsymbol{{x}}^{\boldsymbol{{n}}} =\mathrm{1}\:\boldsymbol{{n}}\in\mathbb{N}… \\ $$
Commented by prakash jain last updated on 05/Jul/20

$$\mathrm{Search}\:\mathrm{internet}\:\mathrm{for}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{unity}. \\ $$$$\mathrm{You}\:\mathrm{will}\:\mathrm{find}\:\mathrm{lot}\:\mathrm{of}\:\mathrm{information}. \\ $$$$\mathrm{to}\:\mathrm{start}\:\mathrm{with}\:{i}=\sqrt{−\mathrm{1}} \\ $$$${e}^{{i}\theta} =\mathrm{cos}\:\theta+{i}\mathrm{sin}\:\theta \\ $$$$\mathrm{1}=\mathrm{cos}\:\mathrm{2}\pi{k}+{i}\mathrm{sin}\:\mathrm{2}\pi{k} \\ $$$$\mathrm{1}^{\mathrm{1}/{n}} ={e}^{\frac{\mathrm{2}\pi{j}}{{n}}} \:\:\left({j}=\mathrm{0},….{n}−\mathrm{1}\right) \\ $$$$\mathrm{as}\:\mathrm{you}\:\mathrm{can}\:\mathrm{see}\:\left({e}^{\frac{\mathrm{2}\pi{j}}{{n}}} \right)^{{n}} ={e}^{\mathrm{2}{j}\pi} =\mathrm{1} \\ $$
Answered by 1549442205 last updated on 06/Jul/20

$$\mathrm{Applying}\:\mathrm{the}\:\mathrm{Mauvra}'\mathrm{s}\:\mathrm{formular}\: \\ $$$$\left(\mathrm{cos}\varphi+\mathrm{isin}\varphi\right)^{\mathrm{n}} =\mathrm{cosn}\varphi+\mathrm{isinn}\varphi\: \\ $$$$\mathrm{Since}\:\mathrm{1}=\mathrm{cos2k}\pi+\mathrm{isin2k}\pi\:,\mathrm{so} \\ $$$$\left.\mathrm{i}\right)\mathrm{For}\:\mathrm{the}\:\mathrm{eqs}.\mathrm{x}^{\mathrm{4}} =\mathrm{1}\:\mathrm{we}\:\mathrm{get}\:\mathrm{x}=\mathrm{1}^{\frac{\mathrm{1}}{\mathrm{4}}} \\ $$$$\Leftrightarrow\mathrm{x}=\left(\mathrm{cos2k}\pi+\mathrm{isin2k}\pi\right)^{\frac{\mathrm{1}}{\mathrm{4}}} =\mathrm{cos}\frac{\mathrm{2k}\pi}{\mathrm{4}}+\mathrm{isin}\frac{\mathrm{2k}\pi}{\mathrm{4}}\left(\mathrm{k}=\mathrm{0},\mathrm{1},\mathrm{2},\mathrm{3}\right) \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{four}\:\mathrm{different}\:\mathrm{roots}\: \\ $$$$\left.\mathrm{ii}\right)\mathrm{For}\:\mathrm{the}\:\mathrm{eqs}.\mathrm{x}^{\mathrm{5}} =\mathrm{1}\Leftrightarrow\mathrm{x}=\mathrm{1}^{\frac{\mathrm{1}}{\mathrm{5}}} .\mathrm{We}\:\mathrm{get} \\ $$$$\mathrm{x}=\left(\mathrm{cos2k}\pi+\mathrm{isin2k}\pi\right)^{\frac{\mathrm{1}}{\mathrm{5}}} \Leftrightarrow\mathrm{x}=\mathrm{cos}\frac{\mathrm{2k}\pi}{\mathrm{5}}+\mathrm{isin}\frac{\mathrm{2k}\pi}{\mathrm{5}}\left(\mathrm{k}\in\left\{\mathrm{0},\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{4}\right\}\right) \\ $$$$\mathrm{weget}\:\mathrm{five}\:\mathrm{different}\:\:\mathrm{roots} \\ $$$$\left.\mathrm{iii}\right)\mathrm{For}\:\mathrm{eqs}.\mathrm{x}^{\mathrm{n}} =\mathrm{1}\Leftrightarrow\mathrm{x}=\mathrm{1}^{\frac{\mathrm{1}}{\mathrm{n}}} .\mathrm{We}\:\mathrm{get} \\ $$$$\mathrm{x}=\left(\mathrm{cos2k}\pi+\mathrm{isin2k}\pi\right)^{\frac{\mathrm{1}}{\mathrm{n}}} =\mathrm{cos}\frac{\mathrm{2k}\pi}{\mathrm{n}}+\mathrm{isin}\frac{\mathrm{2k}\pi}{\mathrm{n}}\left(\mathrm{n}\in\left\{\mathrm{0},\mathrm{1},\mathrm{2},…\mathrm{n}−\mathrm{1}\right\}\right) \\ $$$$\mathrm{weget}\:\mathrm{n}\:\mathrm{different}\:\mathrm{roots} \\ $$$$\mathrm{for}\:\mathrm{k}=\mathrm{n},\mathrm{n}+\mathrm{1},\mathrm{n}+\mathrm{2},…\:\mathrm{we}\:\mathrm{get}\:\mathrm{the}\:\mathrm{repeated}\:\mathrm{roots} \\ $$$$ \\ $$
