Question Number 100317 by pticantor last updated on 26/Jun/20
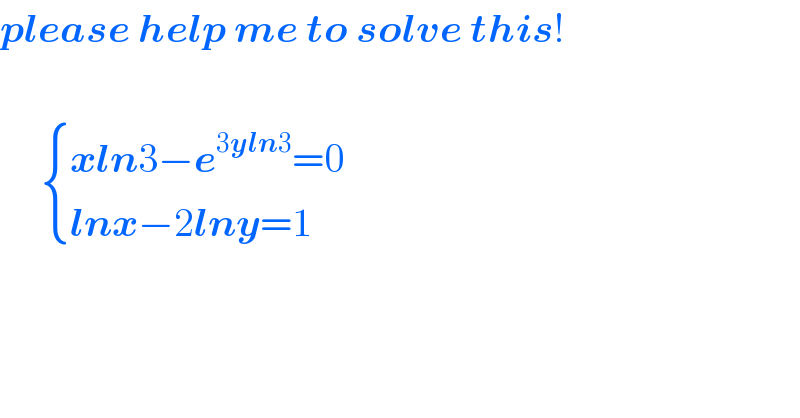
$$\boldsymbol{{please}}\:\boldsymbol{{help}}\:\boldsymbol{{me}}\:\boldsymbol{{to}}\:\boldsymbol{{solve}}\:\boldsymbol{{this}}! \\ $$$$ \\ $$$$\:\:\:\:\:\begin{cases}{\boldsymbol{{xln}}\mathrm{3}−\boldsymbol{{e}}^{\mathrm{3}\boldsymbol{{yln}}\mathrm{3}} =\mathrm{0}}\\{\boldsymbol{{lnx}}−\mathrm{2}\boldsymbol{{lny}}=\mathrm{1}}\end{cases} \\ $$$$ \\ $$
Answered by MJS last updated on 26/Jun/20
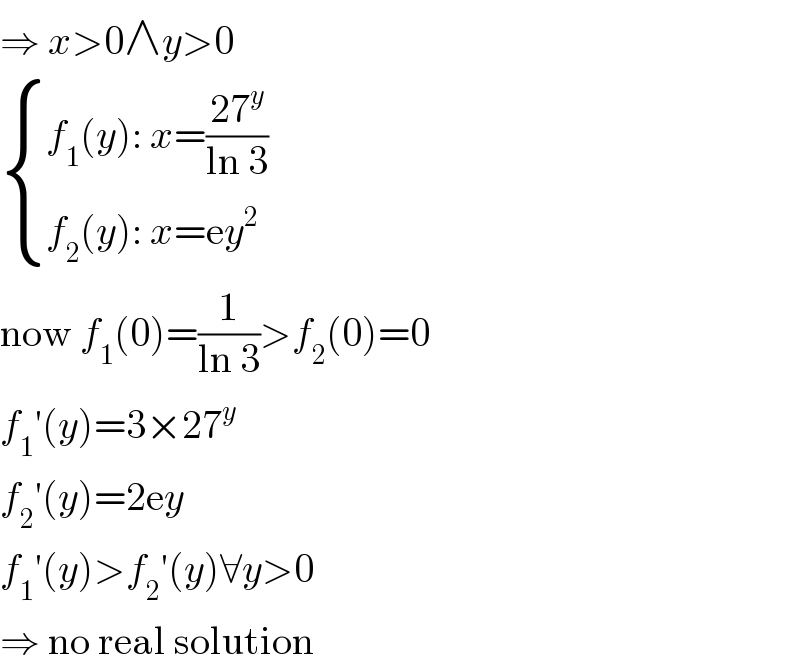
$$\Rightarrow\:{x}>\mathrm{0}\wedge{y}>\mathrm{0} \\ $$$$\begin{cases}{{f}_{\mathrm{1}} \left({y}\right):\:{x}=\frac{\mathrm{27}^{{y}} }{\mathrm{ln}\:\mathrm{3}}}\\{{f}_{\mathrm{2}} \left({y}\right):\:{x}=\mathrm{e}{y}^{\mathrm{2}} }\end{cases} \\ $$$$\mathrm{now}\:{f}_{\mathrm{1}} \left(\mathrm{0}\right)=\frac{\mathrm{1}}{\mathrm{ln}\:\mathrm{3}}>{f}_{\mathrm{2}} \left(\mathrm{0}\right)=\mathrm{0} \\ $$$${f}_{\mathrm{1}} '\left({y}\right)=\mathrm{3}×\mathrm{27}^{{y}} \\ $$$${f}_{\mathrm{2}} '\left({y}\right)=\mathrm{2e}{y} \\ $$$${f}_{\mathrm{1}} '\left({y}\right)>{f}_{\mathrm{2}} '\left({y}\right)\forall{y}>\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{no}\:\mathrm{real}\:\mathrm{solution} \\ $$
Answered by 4635 last updated on 26/Jun/20
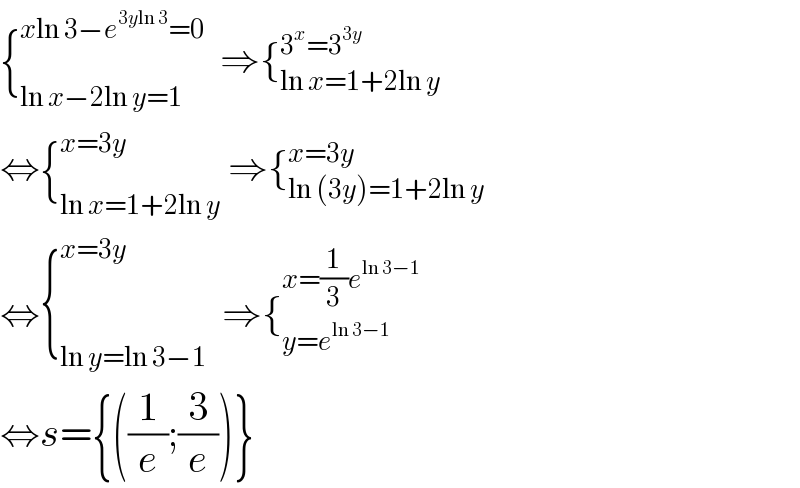
$$\left\{_{\mathrm{ln}\:{x}−\mathrm{2ln}\:{y}=\mathrm{1}} ^{{x}\mathrm{ln}\:\mathrm{3}−{e}^{\mathrm{3}{y}\mathrm{ln}\:\mathrm{3}} =\mathrm{0}} \:\:\Rightarrow\left\{_{\mathrm{ln}\:{x}=\mathrm{1}+\mathrm{2ln}\:{y}} ^{\mathrm{3}^{{x}} =\mathrm{3}^{\mathrm{3}{y}} } \right.\right. \\ $$$$\Leftrightarrow\left\{_{\mathrm{ln}\:{x}=\mathrm{1}+\mathrm{2ln}\:{y}} ^{{x}=\mathrm{3}{y}} \:\Rightarrow\left\{_{\mathrm{ln}\:\left(\mathrm{3}{y}\right)=\mathrm{1}+\mathrm{2ln}\:{y}\:} ^{{x}=\mathrm{3}{y}} \right.\right. \\ $$$$\Leftrightarrow\left\{_{\mathrm{ln}\:{y}=\mathrm{ln}\:\mathrm{3}−\mathrm{1}} ^{{x}=\mathrm{3}{y}} \:\:\Rightarrow\left\{_{{y}={e}^{\mathrm{ln}\:\mathrm{3}−\mathrm{1}} \:} ^{{x}=\frac{\mathrm{1}}{\mathrm{3}}{e}^{\mathrm{ln}\:\mathrm{3}−\mathrm{1}} } \right.\right. \\ $$$$\Leftrightarrow{s}=\left\{\left(\frac{\mathrm{1}}{{e}};\frac{\mathrm{3}}{{e}}\right)\right\} \\ $$
Commented by MJS last updated on 26/Jun/20
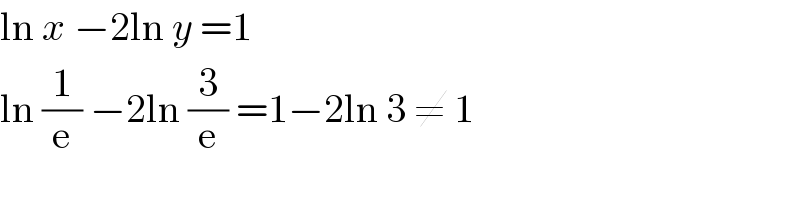
$$\mathrm{ln}\:{x}\:−\mathrm{2ln}\:{y}\:=\mathrm{1} \\ $$$$\mathrm{ln}\:\frac{\mathrm{1}}{\mathrm{e}}\:−\mathrm{2ln}\:\frac{\mathrm{3}}{\mathrm{e}}\:=\mathrm{1}−\mathrm{2ln}\:\mathrm{3}\:\neq\:\mathrm{1} \\ $$
Commented by MJS last updated on 26/Jun/20

$${x}\mathrm{ln}\:\mathrm{3}\:\neq\mathrm{3}^{{x}} \\ $$$$\Rightarrow\:\mathrm{your}\:\mathrm{1}^{\mathrm{st}} \:\mathrm{line}\:\mathrm{is}\:\mathrm{wrong} \\ $$
Commented by bemath last updated on 26/Jun/20

$$\mathrm{xln}\left(\mathrm{3}\right)=\:\mathrm{ln}\left(\mathrm{3}^{\mathrm{x}} \right)\: \\ $$
Commented by 4635 last updated on 26/Jun/20

$${please}\:{sir}\:,\:{I}\:{don}'{t}\:{understand}\:{how} \\ $$$${x}\mathrm{ln}\:\mathrm{3}#\mathrm{3}^{{x}\:} \\ $$
Commented by 4635 last updated on 26/Jun/20

$${ist}\:{good}\:.{but}\:{why}\:{the}\:{solution}\:{that}\:{you}\:\: \\ $$$${see}\:{never}\:{this}\:{equation} \\ $$
Commented by MJS last updated on 26/Jun/20
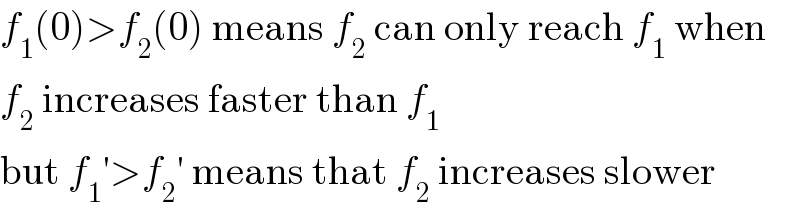
$${f}_{\mathrm{1}} \left(\mathrm{0}\right)>{f}_{\mathrm{2}} \left(\mathrm{0}\right)\:\mathrm{means}\:{f}_{\mathrm{2}} \:\mathrm{can}\:\mathrm{only}\:\mathrm{reach}\:{f}_{\mathrm{1}} \:\mathrm{when} \\ $$$${f}_{\mathrm{2}} \:\mathrm{increases}\:\mathrm{faster}\:\mathrm{than}\:{f}_{\mathrm{1}} \\ $$$$\mathrm{but}\:{f}_{\mathrm{1}} '>{f}_{\mathrm{2}} '\:\mathrm{means}\:\mathrm{that}\:{f}_{\mathrm{2}} \:\mathrm{increases}\:\mathrm{slower} \\ $$
