please-I-need-help-e-x-1-e-2x-1-dx-

Question Number 130226 by stelor last updated on 23/Jan/21

please I need help \dots

\int (\frac{e^{x} + 1}{e^{2 x} + 1}) d x

Answered by Lordose last updated on 23/Jan/21

Ω \overset{u = e^{x}}{=} \int \frac{u + 1}{u (u^{2} + 1)} du = \int \frac{1}{u^{2} + 1} du + \int \frac{1}{u (u^{2} + 1)} du

Ω = \tan^{- 1} (u) + \int (\frac{a}{u} + \frac{bu + c}{u^{2} + 1}) du

1 = a (u^{2} + 1) + u (bu + c)

a = 1

1 = (a + b) u^{2} + a + cu

b = - 1, c = 0

Ω = \tan^{- 1} (u) + \int \frac{1}{u} du - \int \frac{u}{u^{2} + 1} du

Ω = \tan^{- 1} (u) + \ln (u) - \frac{1}{2} \ln (u^{2} + 1) + C

Ω = \tan^{- 1} (e^{x}) + x - \frac{1}{2} \ln (e^{2 x} + 1) + C

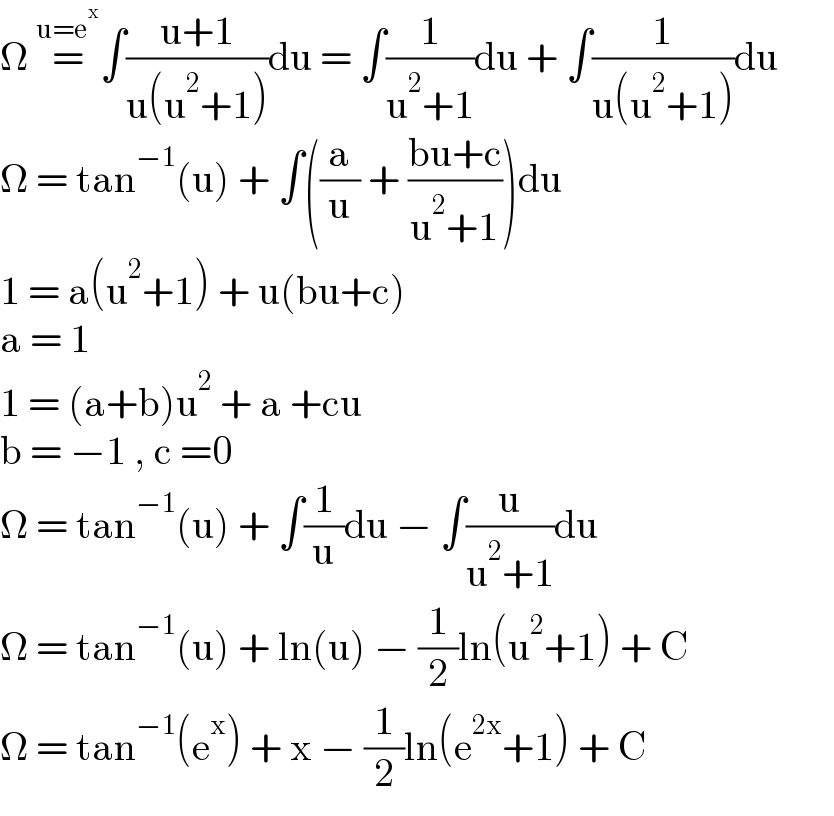
Commented by stelor last updated on 23/Jan/21

good \dots \dots

Answered by EDWIN88 last updated on 23/Jan/21

let e^{x} = \tan r \land dx = \frac{\sec^{2} r}{\tan r} dr \Rightarrow I = \int \frac{(\tan r + 1)}{\sec^{2} r} (\frac{\sec^{2} r}{\tan r}) dr

I = \int \frac{\tan r + 1}{\tan r} dr = r + \int \frac{\cos r}{\sin r} dr

I = r + \int \frac{d (\sin r)}{\sin r} = r + \ln (\sin r) + c

I = \tan^{- 1} (e^{x}) + \ln (\frac{e^{x}}{\sqrt{e^{2 x} + 1}}) + c

I = \tan^{- 1} (e^{x}) + x - \frac{1}{2} \ln (e^{2 x} + 1) + c

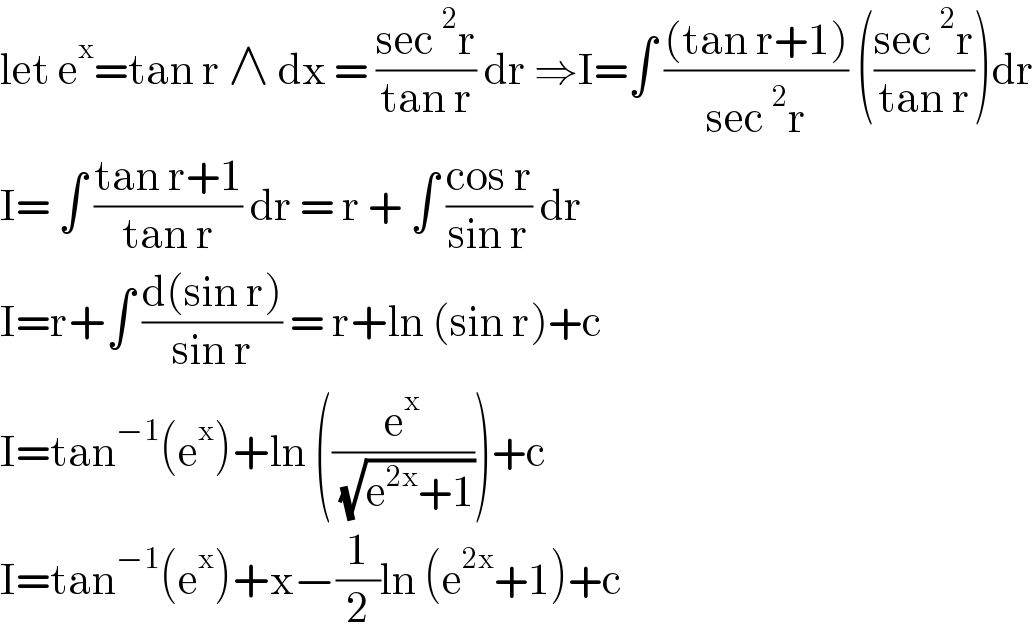
Commented by bramlexs22 last updated on 23/Jan/21

waw \dots elegant

Commented by stelor last updated on 23/Jan/21

cool \dots

Facebook Tweet Pin