Please-solve-

Question Number 174804 by Kallu last updated on 11/Aug/22

P l e a s e s o l v e .

Commented by Kallu last updated on 11/Aug/22

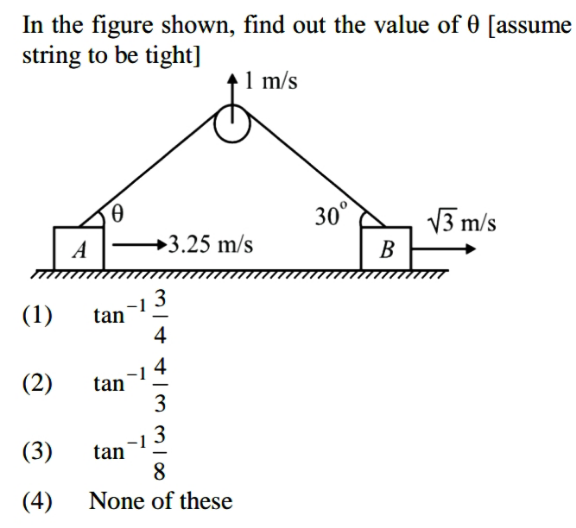
Commented by ajfour last updated on 12/Aug/22

3 . 25 \cos θ - \sin θ = 2 (= \sqrt{{(\sqrt{3})}^{2} + 1^{2}})

\Rightarrow 13 \cos θ - 4 \sin θ = 8

\Rightarrow 13 - 4 \tan θ = 8 \sqrt{1 + \tan^{2} θ}

s a y t = \tan θ

169 + 16 t^{2} - 104 t = 64 + 64 t^{2}

48 t^{2} + 104 t - 105 = 0

t = \frac{52}{48} \pm \frac{\sqrt{52^{2} + 105 \times 48}}{48} = \frac{52 \pm 88}{48}

f o r t > 0, t = \tan θ = \frac{35}{12}

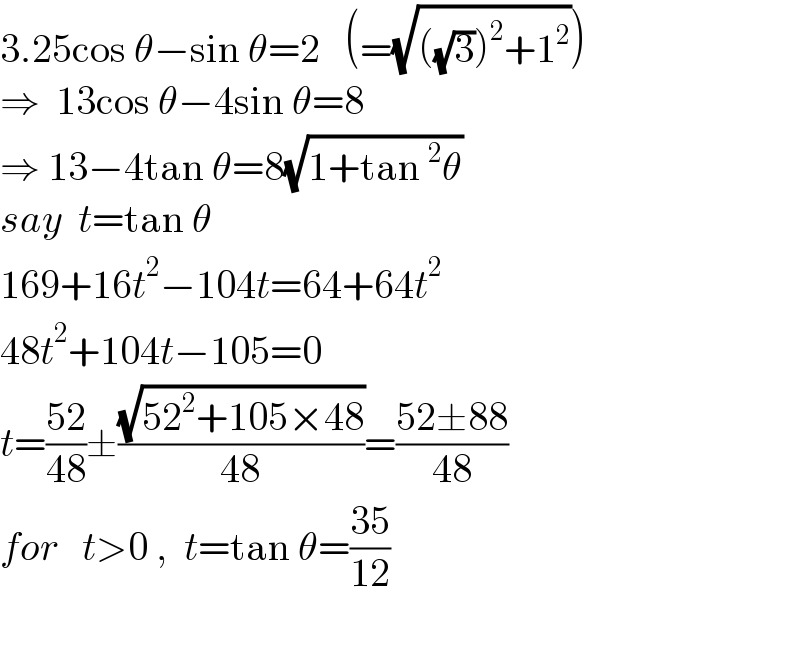
Commented by Tawa11 last updated on 12/Aug/22

Great sir

Commented by Kallu last updated on 12/Aug/22

T h e c o r r e c t a n s w e r i s o p t i o n A

\tan^{- 1} \frac{3}{4}

Facebook Tweet Pin