Proof-for-the-following-n-2-3-n-2-

Question Number 111954 by Hassen_Timol last updated on 05/Sep/20

P roof for the following :

n! ⩾ 2 \times 3^{n - 2}

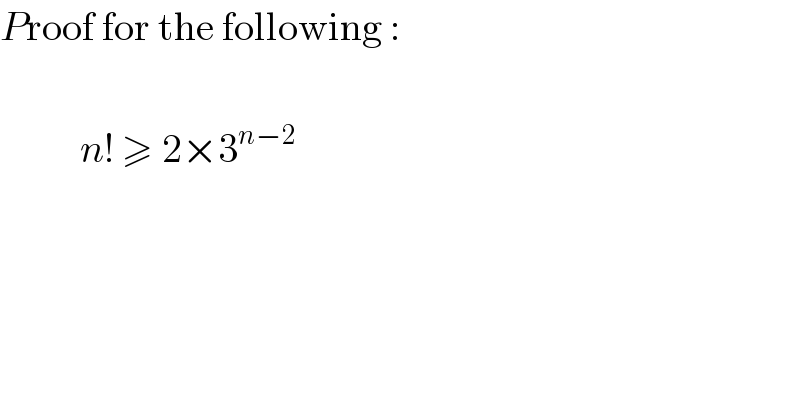
Answered by mathmax by abdo last updated on 05/Sep/20

by recurence n = 2 2! ⩾ 2 \times 1 (true) let suppose n! ⩾ 2 . 3^{n - 2}

(n + 1)! = (n + 1) n! ⩾ (n + 1) 2 . 3^{n - 2} we have

2 (n + 1) 3^{n - 2} - 2 . 3^{n + 1 - 2} = 2 (n + 1) 3^{n - 2} - 2 . 3^{n - 2 + 1}

= 2 . 3^{n - 2} {n + 1 - 3} = 2 . 3^{n - 2} (n - 2) ⩾ 0 due to n ⩾ 2 \Rightarrow

(n + 1)! ⩾ 2 . 3^{n + 1 - 2} the relation is true at term (n + 1)

Commented by aleks041103 last updated on 05/Sep/20

H e u s e d p r o o f b y i n d u c t i o n

Commented by Hassen_Timol last updated on 05/Sep/20

Sorry but I don't understand I think... ��

Commented by mathmax by abdo last updated on 05/Sep/20

I have used recurrence method \dots