Prove-by-principle-of-mathematical-induction-sin-x-sin-2x-sin-3x-sin-nx-cos-1-2-x-cos-n-1-2-x-2-sin-1-2-x-

Question Number 60156 by Tawa1 last updated on 18/May/19

Prove by principle of mathematical induction

\sin (x) + \sin (2 x) + \sin (3 x) + \dots + \sin (nx) = \frac{\cos (\frac{1}{2} x) - \cos (n + \frac{1}{2}) x}{2 \sin (\frac{1}{2} x)}

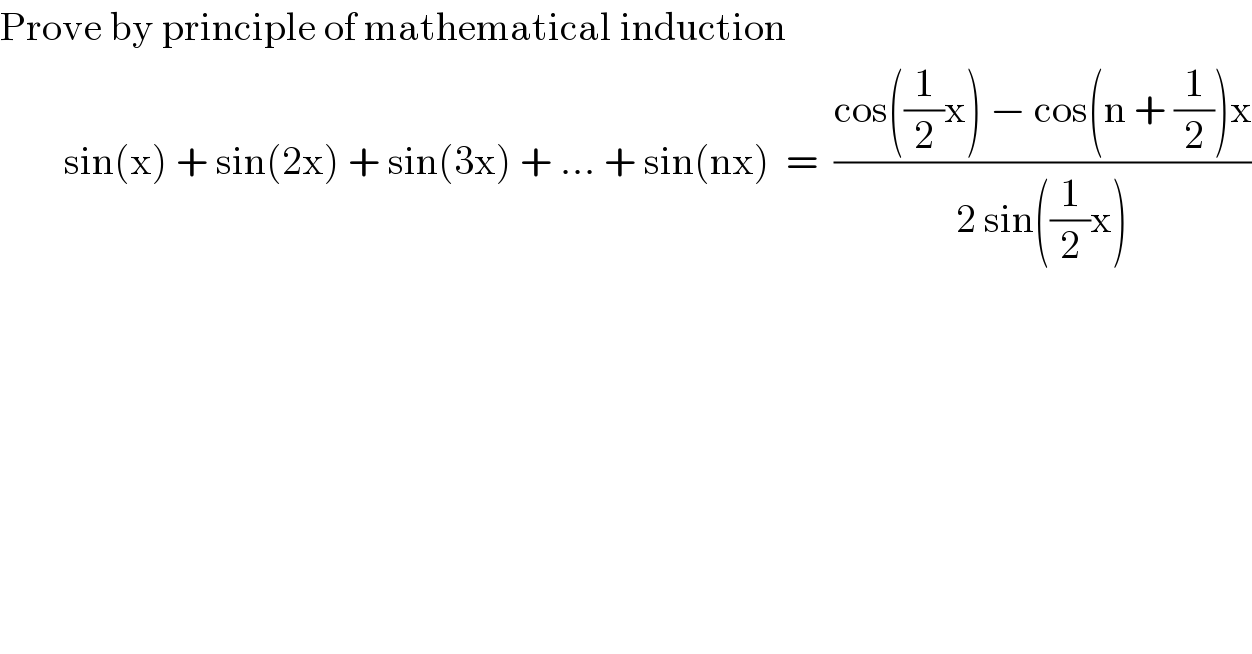
Commented by Smail last updated on 19/May/19

sinx+sin2x+...+sin(nx)=Σ_(k=0) ^n sin(kx) =Im(Σ_(k=0) ^n e^(ikx) ) Σ_(k=0) ^n e^(ikx) =Σ_(k=0) ^n (e^(ix) )^k =((1−(e^(ix) )^(n+1) )/(1−e^(ix) )) =((1−e^(i(n+1)x) )/(1−e^(ix) ))=((1−cos((n+1)x)−isin((n+1)x))/(1−cosx−isinx)) =((2sin^2 (((n+1)/2)x)−2isin(((n+1)/2)x)cos(((n+1)/2)x))/(2sin^2 (x/2)−2isin(x/2)cos(x/2))) =((−i×sin(((n+1)/2)x)(isin(((n+1)/2)x)+cos(((n+1)/2)x)))/(−isin(x/2)×(isin(x/2)+cos(x/2)))) =((sin(((n+1)/2)x)e^(i((n+1)/2)x) )/(sin(x/2)×e^(i(x/2)) ))=((sin(((n+1)/2)x))/(sin(x/2)))e^(i(n/2)x) Im(Σ_(k=0) ^n e^(ikx) )=((sin(((n+1)/2)x)sin((n/2)x))/(sin(x/2))) sinx+sin2x+...+sinnx=((sin(((n+1)/2)x)sin((n/2)x))/(sin(x/2))) =((cos(((n+1)/2)x−(n/2)x)−cos(((n+1)/2)x+(n/2)x))/(2sin(x/2))) =((cos((x/2))−cos(((2n+1)/2)x))/(2sin(x/2)))

s i n x + s i n 2 x + \dots + s i n (n x) = \underset{k = 0}{\sum^{n}} s i n (k x)

= I m (\underset{k = 0}{\sum^{n}} e^{i k x})

\underset{k = 0}{\sum^{n}} e^{i k x} = \underset{k = 0}{\sum^{n}} {(e^{i x})}^{k} = \frac{1 - {(e^{i x})}^{n + 1}}{1 - e^{i x}}

= \frac{1 - e^{i (n + 1) x}}{1 - e^{i x}} = \frac{1 - c o s ((n + 1) x) - i s i n ((n + 1) x)}{1 - c o s x - i s i n x}

= \frac{2 {s i n}^{2} (\frac{n + 1}{2} x) - 2 i s i n (\frac{n + 1}{2} x) c o s (\frac{n + 1}{2} x)}{2 {s i n}^{2} \frac{x}{2} - 2 i s i n \frac{x}{2} c o s \frac{x}{2}}

= \frac{- i \times s i n (\frac{n + 1}{2} x) (i s i n (\frac{n + 1}{2} x) + c o s (\frac{n + 1}{2} x))}{- i s i n \frac{x}{2} \times (i s i n \frac{x}{2} + c o s \frac{x}{2})}

= \frac{s i n (\frac{n + 1}{2} x) e^{i \frac{n + 1}{2} x}}{s i n \frac{x}{2} \times e^{i \frac{x}{2}}} = \frac{s i n (\frac{n + 1}{2} x)}{s i n \frac{x}{2}} e^{i \frac{n}{2} x}

I m (\underset{k = 0}{\sum^{n}} e^{i k x}) = \frac{s i n (\frac{n + 1}{2} x) s i n (\frac{n}{2} x)}{s i n \frac{x}{2}}

s i n x + s i n 2 x + \dots + s i n n x = \frac{s i n (\frac{n + 1}{2} x) s i n (\frac{n}{2} x)}{s i n \frac{x}{2}}

= \frac{c o s (\frac{n + 1}{2} x - \frac{n}{2} x) - c o s (\frac{n + 1}{2} x + \frac{n}{2} x)}{2 s i n \frac{x}{2}}

= \frac{c o s (\frac{x}{2}) - c o s (\frac{2 n + 1}{2} x)}{2 s i n \frac{x}{2}}

Commented by Tawa1 last updated on 19/May/19

God bless you sir

Answered by Kunal12588 last updated on 18/May/19

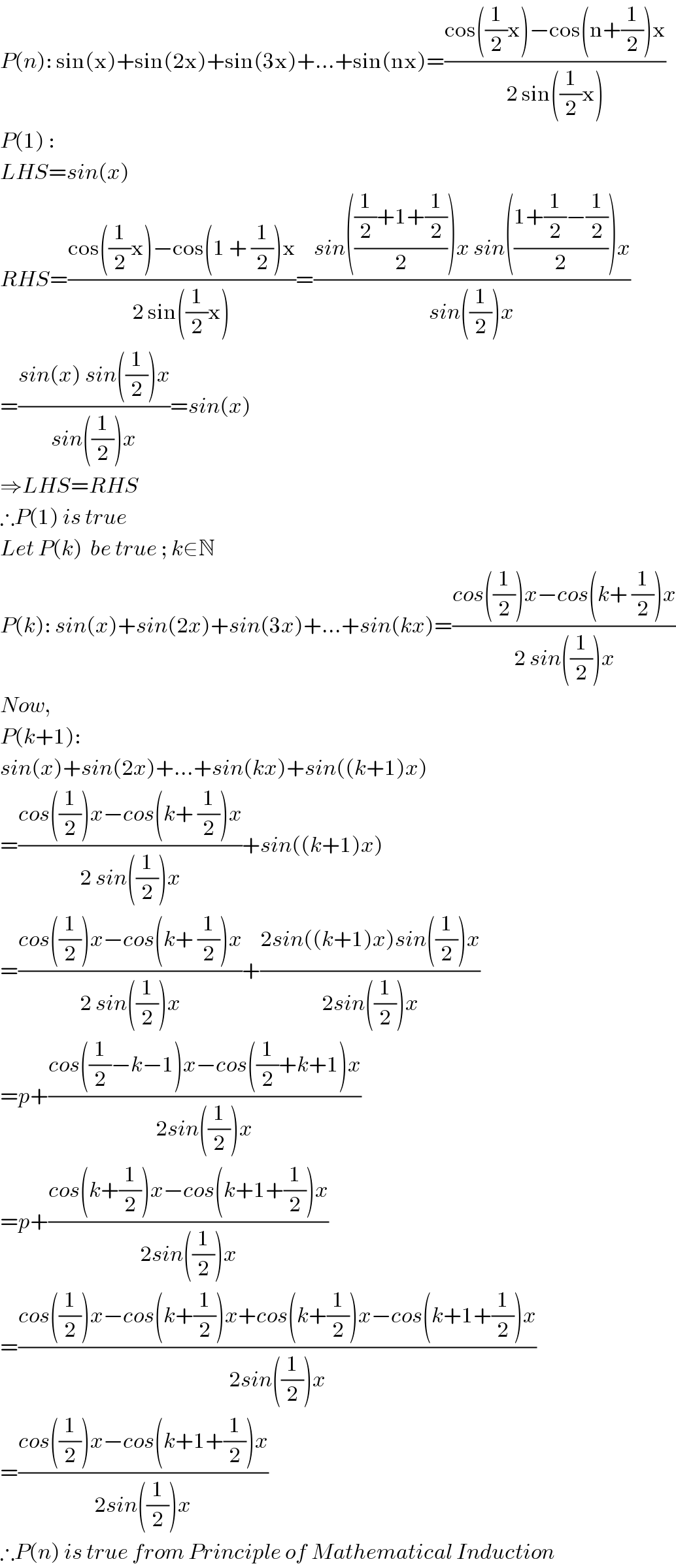
P(n): sin(x)+sin(2x)+sin(3x)+...+sin(nx)=((cos((1/2)x)−cos(n+(1/2))x)/(2 sin((1/2)x))) P(1) : LHS=sin(x) RHS=((cos((1/2)x)−cos(1 + (1/2))x)/(2 sin((1/2)x)))=((sin((((1/2)+1+(1/2))/2))x sin(((1+(1/2)−(1/2))/2))x)/(sin((1/2))x)) =((sin(x) sin((1/2))x)/(sin((1/2))x))=sin(x) ⇒LHS=RHS ∴P(1) is true Let P(k) be true ; k∈N P(k): sin(x)+sin(2x)+sin(3x)+...+sin(kx)=((cos((1/2))x−cos(k+ (1/2))x)/(2 sin((1/2))x)) Now, P(k+1): sin(x)+sin(2x)+...+sin(kx)+sin((k+1)x) =((cos((1/2))x−cos(k+ (1/2))x)/(2 sin((1/2))x))+sin((k+1)x) =((cos((1/2))x−cos(k+ (1/2))x)/(2 sin((1/2))x))+((2sin((k+1)x)sin((1/2))x)/(2sin((1/2))x)) =p+((cos((1/2)−k−1)x−cos((1/2)+k+1)x)/(2sin((1/2))x)) =p+((cos(k+(1/2))x−cos(k+1+(1/2))x)/(2sin((1/2))x)) =((cos((1/2))x−cos(k+(1/2))x+cos(k+(1/2))x−cos(k+1+(1/2))x)/(2sin((1/2))x)) =((cos((1/2))x−cos(k+1+(1/2))x)/(2sin((1/2))x)) ∴P(n) is true from Principle of Mathematical Induction

P (n) : \sin (x) + \sin (2 x) + \sin (3 x) + \dots + \sin (nx) = \frac{\cos (\frac{1}{2} x) - \cos (n + \frac{1}{2}) x}{2 \sin (\frac{1}{2} x)}

P (1) :

L H S = s i n (x)

R H S = \frac{\cos (\frac{1}{2} x) - \cos (1 + \frac{1}{2}) x}{2 \sin (\frac{1}{2} x)} = \frac{s i n (\frac{\frac{1}{2} + 1 + \frac{1}{2}}{2}) x s i n (\frac{1 + \frac{1}{2} - \frac{1}{2}}{2}) x}{s i n (\frac{1}{2}) x}

= \frac{s i n (x) s i n (\frac{1}{2}) x}{s i n (\frac{1}{2}) x} = s i n (x)

\Rightarrow L H S = R H S

∴ P (1) i s t r u e

L e t P (k) b e t r u e; k \in N

P (k) : s i n (x) + s i n (2 x) + s i n (3 x) + \dots + s i n (k x) = \frac{c o s (\frac{1}{2}) x - c o s (k + \frac{1}{2}) x}{2 s i n (\frac{1}{2}) x}

N o w,

P (k + 1) :

s i n (x) + s i n (2 x) + \dots + s i n (k x) + s i n ((k + 1) x)

= \frac{c o s (\frac{1}{2}) x - c o s (k + \frac{1}{2}) x}{2 s i n (\frac{1}{2}) x} + s i n ((k + 1) x)

= \frac{c o s (\frac{1}{2}) x - c o s (k + \frac{1}{2}) x}{2 s i n (\frac{1}{2}) x} + \frac{2 s i n ((k + 1) x) s i n (\frac{1}{2}) x}{2 s i n (\frac{1}{2}) x}

= p + \frac{c o s (\frac{1}{2} - k - 1) x - c o s (\frac{1}{2} + k + 1) x}{2 s i n (\frac{1}{2}) x}

= p + \frac{c o s (k + \frac{1}{2}) x - c o s (k + 1 + \frac{1}{2}) x}{2 s i n (\frac{1}{2}) x}

= \frac{c o s (\frac{1}{2}) x - c o s (k + \frac{1}{2}) x + c o s (k + \frac{1}{2}) x - c o s (k + 1 + \frac{1}{2}) x}{2 s i n (\frac{1}{2}) x}

= \frac{c o s (\frac{1}{2}) x - c o s (k + 1 + \frac{1}{2}) x}{2 s i n (\frac{1}{2}) x}

∴ P (n) i s t r u e f r o m P r i n c i p l e o f M a t h e m a t i c a l I n d u c t i o n

Commented by Tawa1 last updated on 18/May/19

God bless you sir

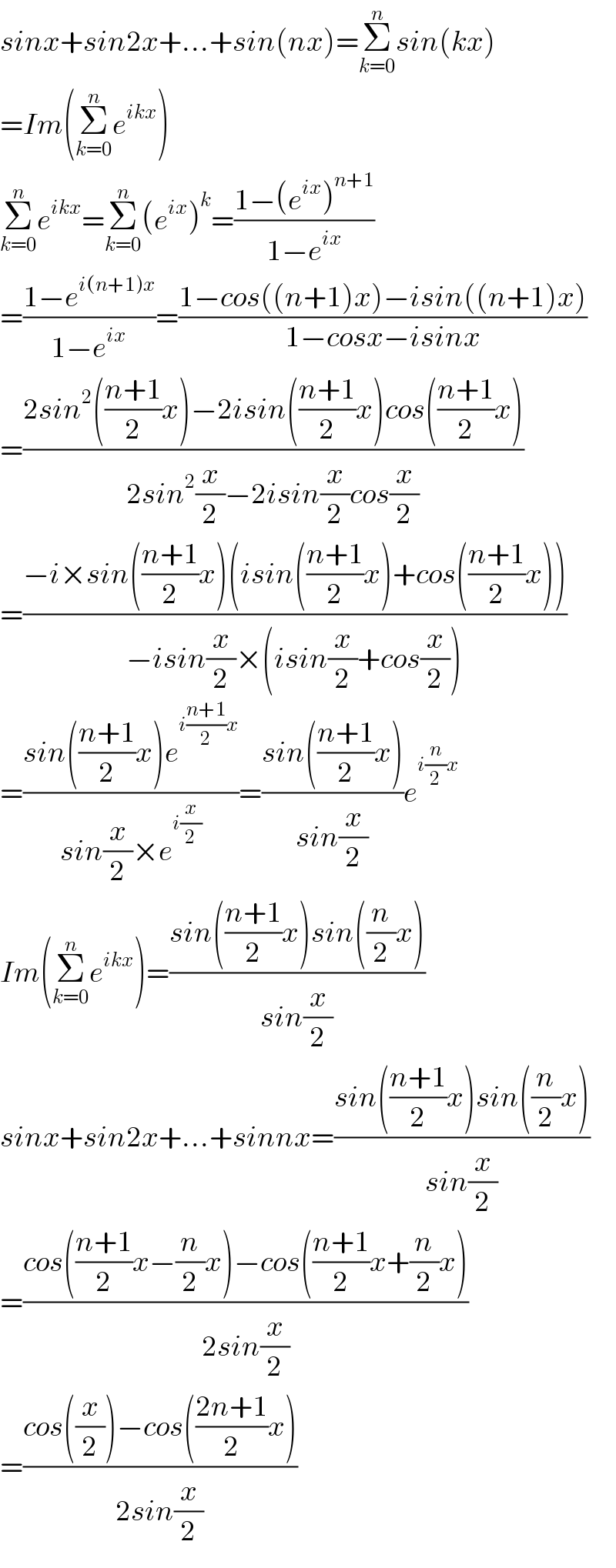
Answered by tanmay last updated on 18/May/19

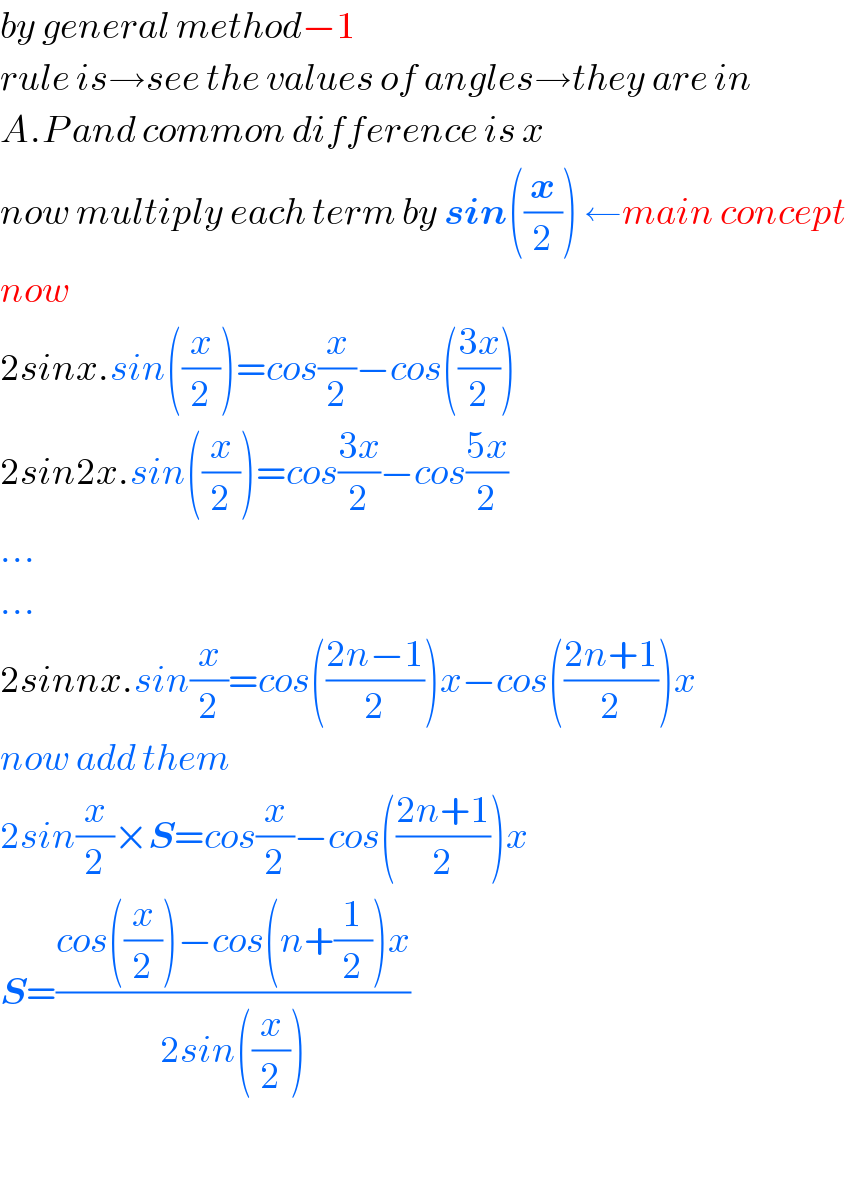
by general method−1 rule is→see the values of angles→they are in A.P and common difference is x now multiply each term by sin((x/2)) ←main concept now 2sinx.sin((x/2))=cos(x/2)−cos(((3x)/2)) 2sin2x.sin((x/2))=cos((3x)/2)−cos((5x)/2) ... ... 2sinnx.sin(x/2)=cos(((2n−1)/2))x−cos(((2n+1)/2))x now add them 2sin(x/2)×S=cos(x/2)−cos(((2n+1)/2))x S=((cos((x/2))−cos(n+(1/2))x)/(2sin((x/2))))

b y g e n e r a l m e t h o d - 1

r u l e i s \to s e e t h e v a l u e s o f a n g l e s \to t h e y a r e i n

A . P a n d c o m m o n d i f f e r e n c e i s x

n o w m u l t i p l y e a c h t e r m b y s i n (\frac{x}{2}) \leftarrow m a i n c o n c e p t

n o w

2 s i n x . s i n (\frac{x}{2}) = c o s \frac{x}{2} - c o s (\frac{3 x}{2})

2 s i n 2 x . s i n (\frac{x}{2}) = c o s \frac{3 x}{2} - c o s \frac{5 x}{2}

\dots

\dots

2 s i n n x . s i n \frac{x}{2} = c o s (\frac{2 n - 1}{2}) x - c o s (\frac{2 n + 1}{2}) x

n o w a d d t h e m

2 s i n \frac{x}{2} \times S = c o s \frac{x}{2} - c o s (\frac{2 n + 1}{2}) x

S = \frac{c o s (\frac{x}{2}) - c o s (n + \frac{1}{2}) x}{2 s i n (\frac{x}{2})}

Commented by Kunal12588 last updated on 18/May/19

that's actually great

Commented by Tawa1 last updated on 18/May/19

God bless you sir

Answered by tanmay last updated on 18/May/19

method−2 p=cosx+cos2x+cos3x+...+cosxnx q=sinx+sin2x+sin3x+...+sinnx p+iq=e^(ix) +e^(i2x) +...+e^(inx) p+iq=((e^(ix) (e^(inx) −1))/(e^(ix) −1)) p+iq=((e^(i(n+1)x) −e^(ix) )/(e^(ix) −1)) =((cos(n+1)x+isin(n+1)x−cosx−isinx)/(cosx+isinx−1)) =(([cos(n+1)x−cosx]+i[sin(n+1)x−sinx])/((cosx−1)+isinx)) =((2sin(x+((nx)/2))sin(((−nx)/2))+i[2cos(x+((nx)/2))sin(((nx)/2)))/(−2sin^2 (x/2)+i2sin(x/2)cos(x/2))) =((2sin((nx)/2))/(2sin(x/2)))×((−sin(x+((nx)/2))+icos(x+((nx)/2)))/(−sin(x/2)+icos(x/2))) =((sin((nx)/2))/(sin(x/2)))×(([sin(x+((nx)/2))−icos(x+((nx)/2))][sin(x/2)+icos(x/2)])/((sin(x/2)−icos(x/2))(sin(x/2)+icos(x/2)))) =((sin((nx)/2))/(sin(x/2)))×(([sin(x+((nx)/2))sin(x/2)+isin(x+((nx)/2))cos(x/2)−icos(x+((nx)/2))sin(x/2)+cos(x+((nx)/2))cos(x/2)])/(sin^2 (x/2)+cos^2 (x/2))) =((sin((nx)/2))/(sin(x/2)))×((cos(((nx)/2)+(x/2))+isin(((nx)/2)+(x/2)))/1) =[((sin((nx)/2))/(sin(x/2)))×cos(n+1)(x/2)]+i[((sin((nx)/2))/(sin(x/2)))×sin(n+1)(x/2)] =[((sin(((nx)/2)+((nx)/2)+(x/2))−sin((x/2)))/(2sin(x/2)))]+i[((cos(x/2)−cos(((nx)/2)+((nx)/2)+(x/2))/(2sin(x/2)))] So p=Σ_(r=1) ^n cosrx=[((sin(nx+(x/2)))/(2sin(x/2)))] q=Σ_(r=1) ^n sinrx=[((cos(x/2)−cos(nx+(x/2)))/(2sin(x/2)))] this is second method... =

m e t h o d - 2

p = c o s x + c o s 2 x + c o s 3 x + \dots + c o s x n x

q = s i n x + s i n 2 x + s i n 3 x + \dots + s i n n x

p + i q = e^{i x} + e^{i 2 x} + \dots + e^{i n x}

p + i q = \frac{e^{i x} (e^{i n x} - 1)}{e^{i x} - 1}

p + i q = \frac{e^{i (n + 1) x} - e^{i x}}{e^{i x} - 1}

= \frac{c o s (n + 1) x + i s i n (n + 1) x - c o s x - i s i n x}{c o s x + i s i n x - 1}

= \frac{[c o s (n + 1) x - c o s x] + i [s i n (n + 1) x - s i n x]}{(c o s x - 1) + i s i n x}

= \frac{2 s i n (x + \frac{n x}{2}) s i n (\frac{- n x}{2}) + i [2 c o s (x + \frac{n x}{2}) s i n (\frac{n x}{2})}{- 2 {s i n}^{2} \frac{x}{2} + i 2 s i n \frac{x}{2} c o s \frac{x}{2}}

= \frac{2 s i n \frac{n x}{2}}{2 s i n \frac{x}{2}} \times \frac{- s i n (x + \frac{n x}{2}) + i c o s (x + \frac{n x}{2})}{- s i n \frac{x}{2} + i c o s \frac{x}{2}}

= \frac{s i n \frac{n x}{2}}{s i n \frac{x}{2}} \times \frac{[s i n (x + \frac{n x}{2}) - i c o s (x + \frac{n x}{2})] [s i n \frac{x}{2} + i c o s \frac{x}{2}]}{(s i n \frac{x}{2} - i c o s \frac{x}{2}) (s i n \frac{x}{2} + i c o s \frac{x}{2})}

= \frac{s i n \frac{n x}{2}}{s i n \frac{x}{2}} \times \frac{[s i n (x + \frac{n x}{2}) s i n \frac{x}{2} + i s i n (x + \frac{n x}{2}) c o s \frac{x}{2} - i c o s (x + \frac{n x}{2}) s i n \frac{x}{2} + c o s (x + \frac{n x}{2}) c o s \frac{x}{2}]}{{s i n}^{2} \frac{x}{2} + {c o s}^{2} \frac{x}{2}}

= \frac{s i n \frac{n x}{2}}{s i n \frac{x}{2}} \times \frac{c o s (\frac{n x}{2} + \frac{x}{2}) + i s i n (\frac{n x}{2} + \frac{x}{2})}{1}

= [\frac{s i n \frac{n x}{2}}{s i n \frac{x}{2}} \times c o s (n + 1) \frac{x}{2}] + i [\frac{s i n \frac{n x}{2}}{s i n \frac{x}{2}} \times s i n (n + 1) \frac{x}{2}]

= [\frac{s i n (\frac{n x}{2} + \frac{n x}{2} + \frac{x}{2}) - s i n (\frac{x}{2})}{2 s i n \frac{x}{2}}] + i [\frac{c o s \frac{x}{2} - c o s (\frac{n x}{2} + \frac{n x}{2} + \frac{x}{2}}{2 s i n \frac{x}{2}}]

S o

p = \underset{r = 1}{\sum^{n}} c o s r x = [\frac{s i n (n x + \frac{x}{2})}{2 s i n \frac{x}{2}}]

q = \underset{r = 1}{\sum^{n}} s i n r x = [\frac{c o s \frac{x}{2} - c o s (n x + \frac{x}{2})}{2 s i n \frac{x}{2}}]

t h i s i s s e c o n d m e t h o d \dots

=

Commented by Tawa1 last updated on 18/May/19

God bless you sir

Commented by Tawa1 last updated on 18/May/19

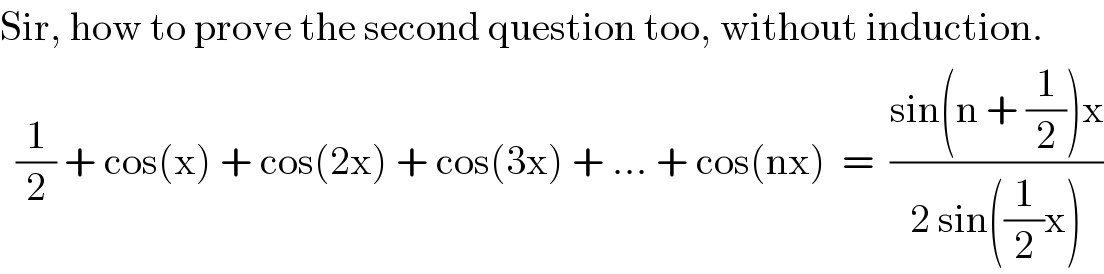
Sir, how to prove the second question too, without induction. (1/2) + cos(x) + cos(2x) + cos(3x) + ... + cos(nx) = ((sin(n + (1/2))x)/(2 sin((1/2)x)))

Sir, how to prove the second question too, without induction .

\frac{1}{2} + \cos (x) + \cos (2 x) + \cos (3 x) + \dots + \cos (nx) = \frac{\sin (n + \frac{1}{2}) x}{2 \sin (\frac{1}{2} x)}

Commented by Tawa1 last updated on 18/May/19

Thanks for your time .

Commented by tanmay last updated on 18/May/19

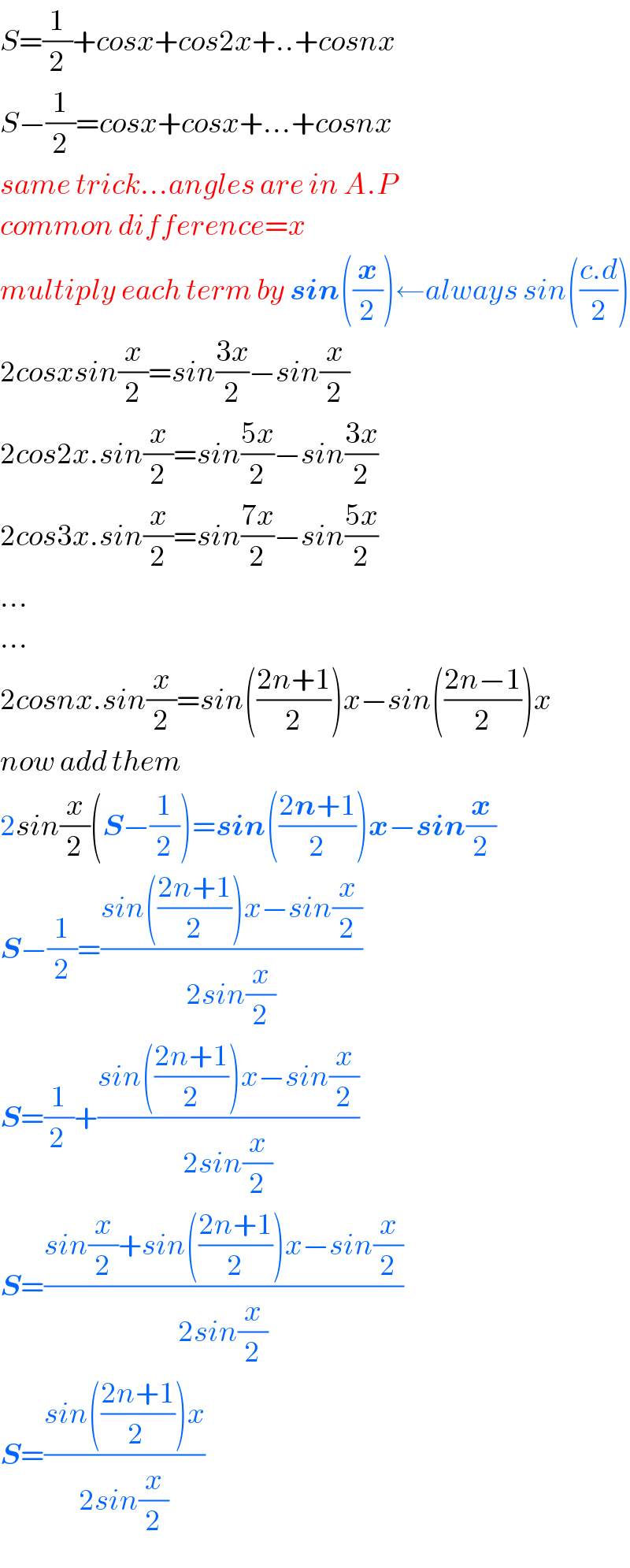
S=(1/2)+cosx+cos2x+..+cosnx S−(1/2)=cosx+cosx+...+cosnx same trick...angles are in A.P common difference=x multiply each term by sin((x/2))←always sin(((c.d)/2)) 2cosxsin(x/2)=sin((3x)/2)−sin(x/2) 2cos2x.sin(x/2)=sin((5x)/2)−sin((3x)/2) 2cos3x.sin(x/2)=sin((7x)/2)−sin((5x)/2) ... ... 2cosnx.sin(x/2)=sin(((2n+1)/2))x−sin(((2n−1)/2))x now add them 2sin(x/2)(S−(1/2))=sin(((2n+1)/2))x−sin(x/2) S−(1/2)=((sin(((2n+1)/2))x−sin(x/2))/(2sin(x/2))) S=(1/(2 ))+((sin(((2n+1)/2))x−sin(x/2))/(2sin(x/2))) S=((sin(x/2)+sin(((2n+1)/2))x−sin(x/2))/(2sin(x/2))) S=((sin(((2n+1)/(2 )))x)/(2sin(x/2)))

S = \frac{1}{2} + c o s x + c o s 2 x + . . + c o s n x

S - \frac{1}{2} = c o s x + c o s x + \dots + c o s n x

s a m e t r i c k \dots a n g l e s a r e i n A . P

c o m m o n d i f f e r e n c e = x

m u l t i p l y e a c h t e r m b y s i n (\frac{x}{2}) \leftarrow a l w a y s s i n (\frac{c . d}{2})

2 c o s x s i n \frac{x}{2} = s i n \frac{3 x}{2} - s i n \frac{x}{2}

2 c o s 2 x . s i n \frac{x}{2} = s i n \frac{5 x}{2} - s i n \frac{3 x}{2}

2 c o s 3 x . s i n \frac{x}{2} = s i n \frac{7 x}{2} - s i n \frac{5 x}{2}

\dots

\dots

2 c o s n x . s i n \frac{x}{2} = s i n (\frac{2 n + 1}{2}) x - s i n (\frac{2 n - 1}{2}) x

n o w a d d t h e m

2 s i n \frac{x}{2} (S - \frac{1}{2}) = s i n (\frac{2 n + 1}{2}) x - s i n \frac{x}{2}

S - \frac{1}{2} = \frac{s i n (\frac{2 n + 1}{2}) x - s i n \frac{x}{2}}{2 s i n \frac{x}{2}}

S = \frac{1}{2} + \frac{s i n (\frac{2 n + 1}{2}) x - s i n \frac{x}{2}}{2 s i n \frac{x}{2}}

S = \frac{s i n \frac{x}{2} + s i n (\frac{2 n + 1}{2}) x - s i n \frac{x}{2}}{2 s i n \frac{x}{2}}

S = \frac{s i n (\frac{2 n + 1}{2}) x}{2 s i n \frac{x}{2}}

Commented by Tawa1 last updated on 18/May/19

I really appreciate sir . God bless you .

Commented by malwaan last updated on 19/May/19

FANTASTIC s i r

Facebook Tweet Pin

Prove-by-principle-of-mathematical-induction-sin-x-sin-2x-sin-3x-sin-nx-cos-1-2-x-cos-n-1-2-x-2-sin-1-2-x-

Leave a Reply Cancel reply