prove-Fg-G-m-1-m-2-r-2-

Question Number 98270 by M±th+et+s last updated on 12/Jun/20

p r o v e

F g = G \frac{m_{1} m_{2}}{r^{2}}

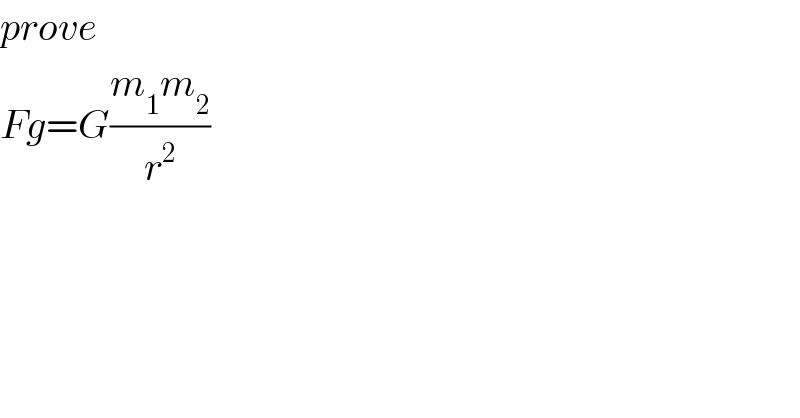
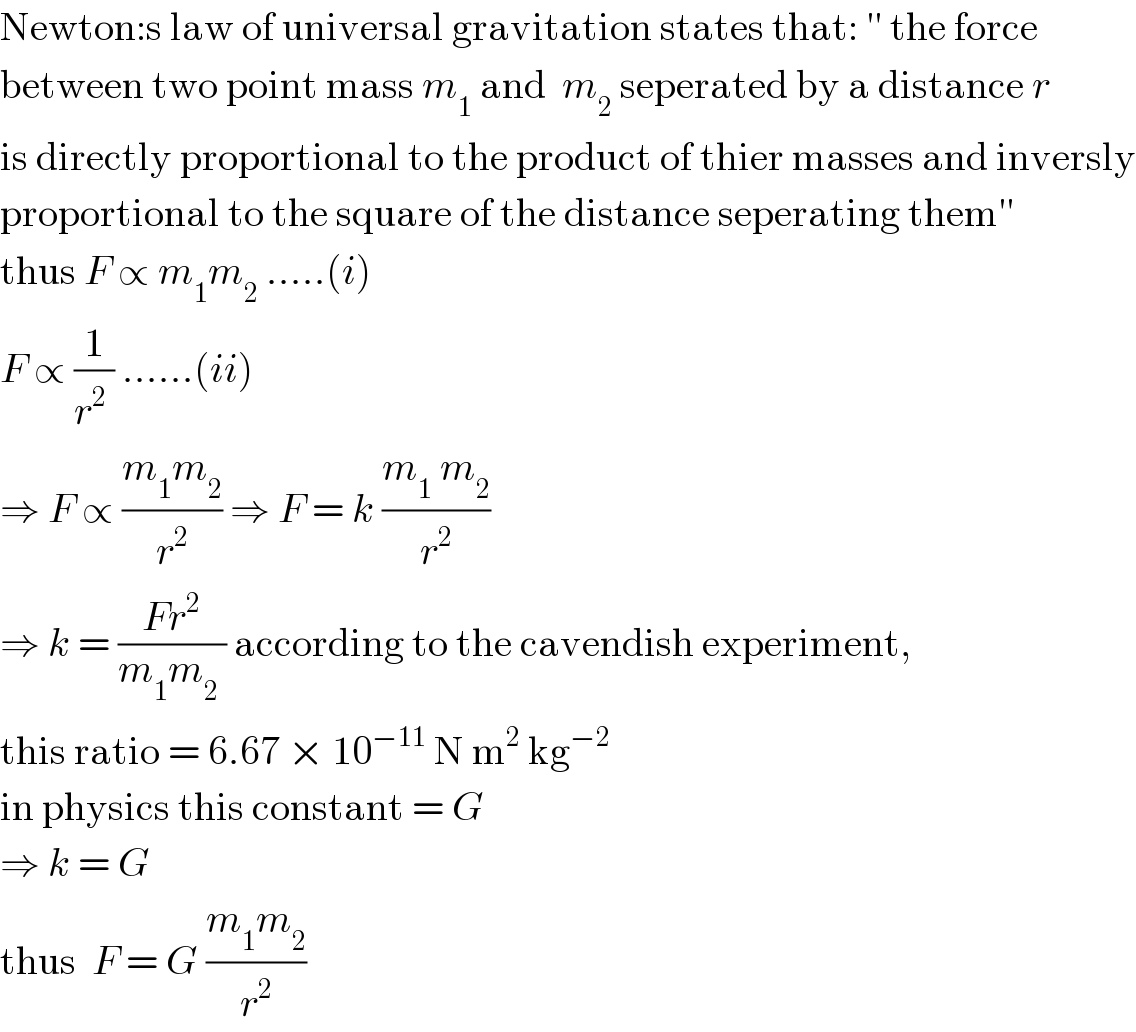
Answered by Rio Michael last updated on 12/Jun/20

Newton : s law of universal gravitation states that :^{″} the force

between two point mass m_{1} and m_{2} seperated by a distance r

is directly proportional to the product of thier masses and inversly

proportional to the square of the distance seperating {them}^{″}

thus F \propto m_{1} m_{2} \dots . . (i)

F \propto \frac{1}{r^{2}} \dots \dots (i i)

\Rightarrow F \propto \frac{m_{1} m_{2}}{r^{2}} \Rightarrow F = k \frac{m_{1} m_{2}}{r^{2}}

\Rightarrow k = \frac{{F r}^{2}}{m_{1} m_{2}} according to the cavendish experiment,

this ratio = 6.67 \times 10^{- 11} N m^{2} {kg}^{- 2}

in physics this constant = G

\Rightarrow k = G

thus F = G \frac{m_{1} m_{2}}{r^{2}}

Commented by M±th+et+s last updated on 12/Jun/20

t h a n k y o u s i r

Answered by smridha last updated on 13/Jun/20

t h i s i s v e r y s i l l y q u e s t i o n!! {d o n}^{'} t

m i n d . . b u t i t i s . w e d e r i v e d

t h i s f o r m u l a f r o m N e w {t o n}^{'} s

g r a v i t a t i o n a l l a w \dots w h i c h i s a

s t a t e m e n t w h o e s m a t h e m a t i c a l

e x p r e s s i o n i s t h a t \dots y o u c a n n o t

p r o v e a n y p o s t u l a t e!!

b u t {i t}^{'} s t r u e t h a t i t c a n b e s h o w n

f r o m E i n s t e i n G e n e r a l r e l a t i v i t y

w i t h m u c h m o r e a p p r o x i m a t i o n .

A n d o n c e m o r e f a c t I w a n t t o

s h a r e t h a t d o n t c a l l {N e w t o n}^{'} s

g r a v i t a t i o n a l L a w i s u n i v e r s a l

l a w \dots b e c a u s e i t d o e s n o t v a l l i d

f o r s t r o n g g r a v i t a t i o n a l f i e l d,

b u t E i n {s t e i n}^{'} G T R c a n e x p l a i n

h o w g r a v i t y w o r k s \dots t h i s t h e

r e g o r u s d e s c r i p t i o n a b o u t

g r a v i t y \dots . .

G_{μ ν} = R_{μ ν} - \frac{1}{2} {R g}_{μ ν} = \frac{8 π G}{c^{4}} T

t h i s i s c a l l e d t h e {E i n s t e i n}^{'} s

t e n s o r {e q}^{n} . t h i s i s t h e e x p l a n a t i o n

o f h o w g r a v i t y w o r k s \dots w h a t i s

g r a v i t y ? ? ?

Commented by M±th+et+s last updated on 13/Jun/20

n i c e e x p l a i n i n g s i r