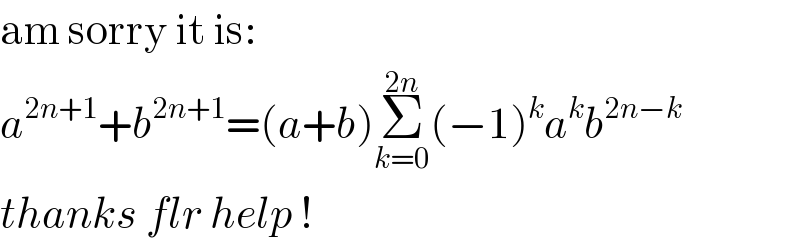Menu Close

prove-n-N-a-b-C-2-a-2n-1-b-2n-1-k-o-2n-1-k-a-k-b-2n-k-

Tinku Tara 0 Comments
Pin




Question Number 21154 by youssoufab last updated on 14/Sep/17
prove: ∀n∈N^∗ ,∀(a,b)∈C^2 , a^(2n+1) +b^(2n+1) = Σ_(k=o) ^(2n) (−1)^k a^k b^(2n−k)
$${prove}:\:\forall{n}\in\mathbb{N}^{\ast} ,\forall\left({a},{b}\right)\in\mathbb{C}^{\mathrm{2}} ,\:{a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} = \\ $$$$\underset{{k}={o}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}−{k}} \\ $$
Commented by alex041103 last updated on 15/Sep/17
What does C^2 mean?? Sorry for the question.
$${What}\:{does}\:\mathbb{C}^{\mathrm{2}} \:{mean}??\:{Sorry}\:{for}\:{the} \\ $$$${question}. \\ $$
Commented by sma3l2996 last updated on 15/Sep/17
that′s mean a∈C and b∈C
$${that}'{s}\:{mean}\:{a}\in\mathbb{C}\:{and}\:{b}\in\mathbb{C} \\ $$
Answered by alex041103 last updated on 15/Sep/17
Let′s start with the expresion (a+b)(Σ_(k=0) ^(2n) (−1)^k a^k b^(2n−k) )= =Σ_(k=0) ^(2n) (−1)^k a^(k+1) b^(2n−k) +Σ_(k=0) ^(2n) (−1)^k a^k b^(2n+1−k) = =(−1)^(2n) a^(2n+1) b^0 +Σ_(k=0) ^(2n−1) (−1)^k a^(k+1) b^(2n−k) +(−1)^0 a^0 b^(2n+1) +Σ_(k=1) ^(2n) (−1)^k a^k b^(2n+1−k) = =a^(2n+1) +b^(2n+1) +Σ_(k=1) ^(2n) (−1)^(k−1) a^k b^(2n+1−k) +Σ_(k=1) ^(2n) (−1)^k a^k b^(2n+1−k) Because (−1)^(k−1) =(((−1)^k )/(−1))=−(−1)^k ⇒(a+b)(Σ_(k=0) ^(2n) (−1)^k a^k b^(2n−k) )= =a^(2n+1) +b^(2n+1) −Σ_(k=1) ^(2n) (−1)^k a^k b^(2n+1−k) +Σ_(k=1) ^(2n) (−1)^k a^k b^(2n+1−k) = =a^(2n+1) +b^(2n+1) Note: The statement a^(2n+1) +b^(2n+1) =Σ_(k=0) ^(2n) (−1)^k a^k b^(2n−k) is False. The true statement is that a^(2n+1) +b^(2n+1) =(a+b)[Σ_(k=0) ^(2n) (−1)^k a^k b^(2n−k) ]
$${Let}'{s}\:{start}\:{with}\:{the}\:{expresion} \\ $$$$\left({a}+{b}\right)\left(\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}−{k}} \right)= \\ $$$$=\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}+\mathrm{1}} {b}^{\mathrm{2}{n}−{k}} +\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}+\mathrm{1}−{k}} = \\ $$$$=\left(−\mathrm{1}\right)^{\mathrm{2}{n}} {a}^{\mathrm{2}{n}+\mathrm{1}} {b}^{\mathrm{0}} +\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}−\mathrm{1}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}+\mathrm{1}} {b}^{\mathrm{2}{n}−{k}} +\left(−\mathrm{1}\right)^{\mathrm{0}} {a}^{\mathrm{0}} {b}^{\mathrm{2}{n}+\mathrm{1}} +\underset{{k}=\mathrm{1}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}+\mathrm{1}−{k}} = \\ $$$$={a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} +\underset{{k}=\mathrm{1}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}−\mathrm{1}} {a}^{{k}} {b}^{\mathrm{2}{n}+\mathrm{1}−{k}} +\underset{{k}=\mathrm{1}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}+\mathrm{1}−{k}} \\ $$$${Because}\:\left(−\mathrm{1}\right)^{{k}−\mathrm{1}} =\frac{\left(−\mathrm{1}\right)^{{k}} }{−\mathrm{1}}=−\left(−\mathrm{1}\right)^{{k}} \\ $$$$\Rightarrow\left({a}+{b}\right)\left(\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}−{k}} \right)= \\ $$$$={a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} −\underset{{k}=\mathrm{1}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}+\mathrm{1}−{k}} +\underset{{k}=\mathrm{1}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}+\mathrm{1}−{k}} = \\ $$$$={a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} \\ $$$$ \\ $$$${Note}:\:{The}\:{statement} \\ $$$${a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} =\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}−{k}} \\ $$$${is}\:{False}. \\ $$$${The}\:{true}\:{statement}\:{is}\:{that} \\ $$$${a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} =\left({a}+{b}\right)\left[\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}−{k}} \right] \\ $$$$ \\ $$
Commented by alex041103 last updated on 15/Sep/17
Note: The false statement a^(2n+1) +b^(2n+1) =Σ_(k=0) ^(2n) (−1)^k a^k b^(2n−k) is in fact allways true when a+b=1 or { ((Re(a)+Re(b)=1)),((Im(a)=−Im(b))) :} And also when a+b≠0 and a^(2n+1) +b^(2n+1) =0 (both of those are true for n≠0)
$${Note}:\:{The}\:{false}\:{statement} \\ $$$${a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} =\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}−{k}} \\ $$$${is}\:{in}\:{fact}\:{allways}\:{true}\:{when}\:{a}+{b}=\mathrm{1} \\ $$$${or}\:\begin{cases}{{Re}\left({a}\right)+{Re}\left({b}\right)=\mathrm{1}}\\{{Im}\left({a}\right)=−{Im}\left({b}\right)}\end{cases} \\ $$$${And}\:{also}\:{when}\:{a}+{b}\neq\mathrm{0}\:{and}\: \\ $$$${a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} =\mathrm{0}\:\left({both}\:{of}\:{those}\:{are}\:{true}\right. \\ $$$$\left.{for}\:{n}\neq\mathrm{0}\right) \\ $$
Commented by youssoufab last updated on 15/Sep/17
am sorry it is: a^(2n+1) +b^(2n+1) =(a+b)Σ_(k=0) ^(2n) (−1)^k a^k b^(2n−k) thanks flr help !
$$\mathrm{am}\:\mathrm{sorry}\:\mathrm{it}\:\mathrm{is}: \\ $$$${a}^{\mathrm{2}{n}+\mathrm{1}} +{b}^{\mathrm{2}{n}+\mathrm{1}} =\left({a}+{b}\right)\underset{{k}=\mathrm{0}} {\overset{\mathrm{2}{n}} {\sum}}\left(−\mathrm{1}\right)^{{k}} {a}^{{k}} {b}^{\mathrm{2}{n}−{k}} \\ $$$${thanks}\:{flr}\:{help}\:! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *