Question Number 165072 by mathls last updated on 25/Jan/22
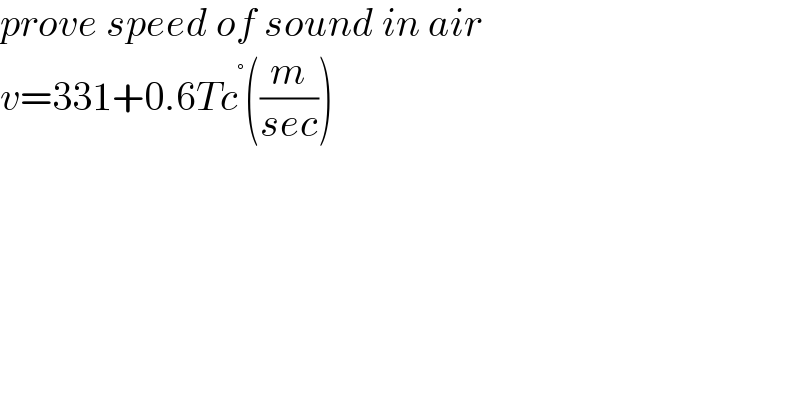
Commented by mr W last updated on 25/Jan/22

Commented by mr W last updated on 25/Jan/22

Commented by mathls last updated on 25/Jan/22
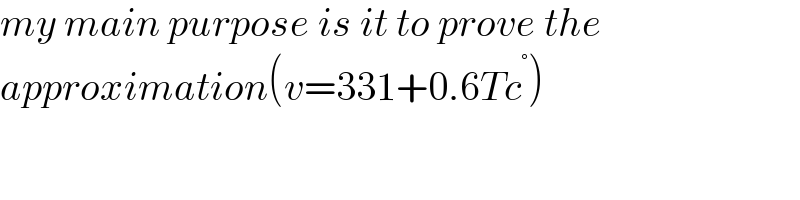
Commented by mr W last updated on 25/Jan/22

Commented by mathls last updated on 25/Jan/22
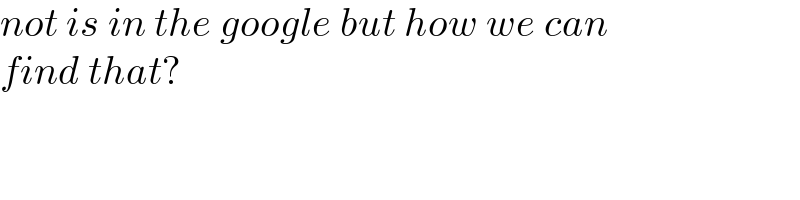
Commented by mathls last updated on 26/Jan/22
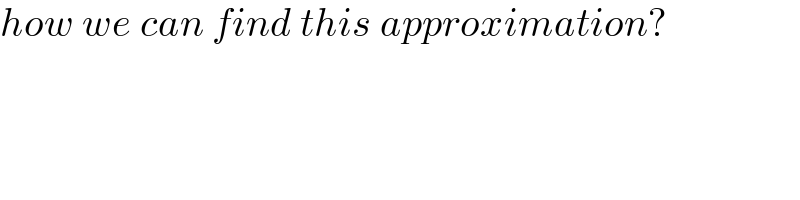
Commented by mr W last updated on 26/Jan/22

Commented by mr W last updated on 26/Jan/22
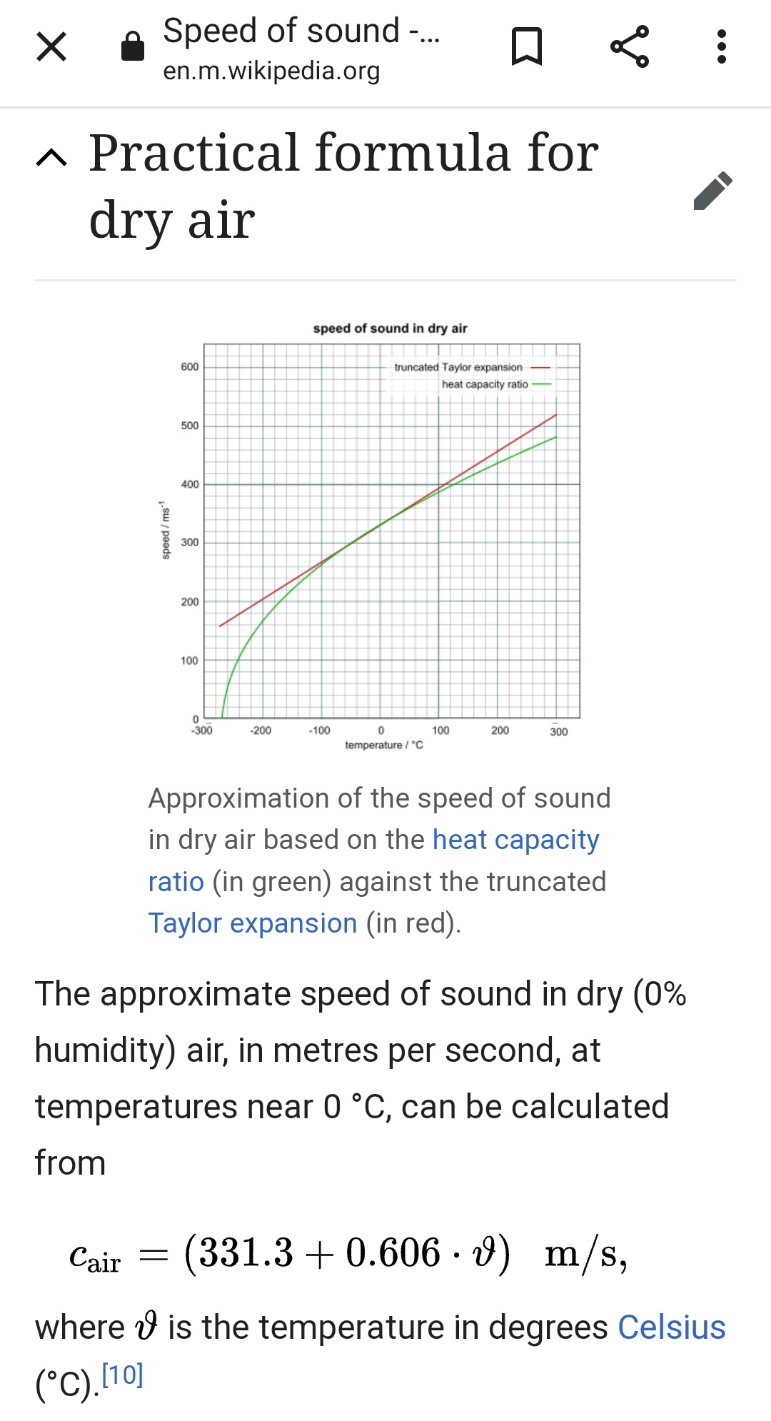
Commented by mr W last updated on 26/Jan/22
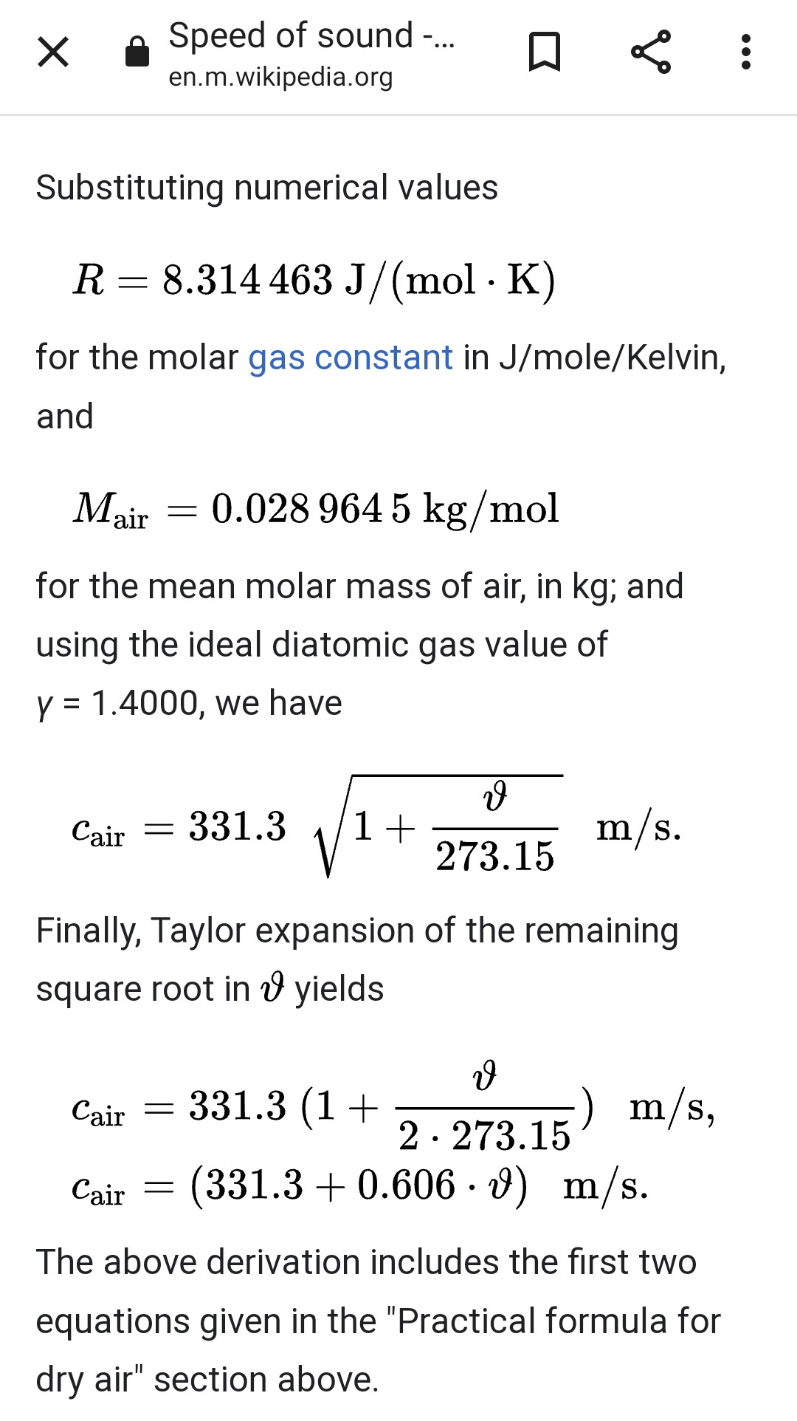
Commented by mr W last updated on 26/Jan/22

Commented by Tinku Tara last updated on 26/Jan/22

Commented by mathls last updated on 26/Jan/22
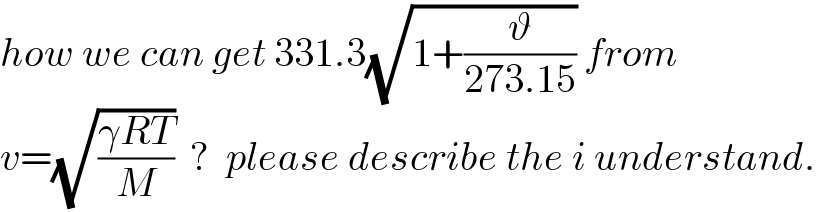
Commented by mathls last updated on 26/Jan/22
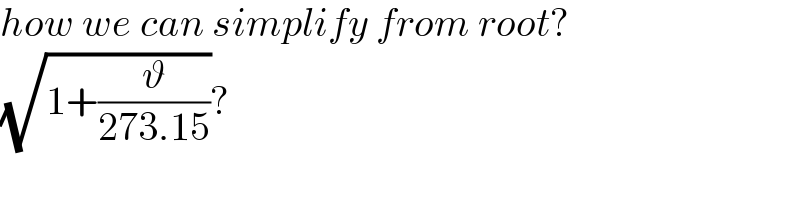
Commented by mr W last updated on 26/Jan/22

