prove-that-0-cos-x-2-dx-0-sin-x-2-dx-pi-2-2-

Question Number 113004 by malwan last updated on 10/Sep/20

p r o v e t h a t

_{0} \int^{\infty} c o s (x^{2}) d x =_{0} \int^{\infty} s i n (x^{2}) d x = \frac{\sqrt{π}}{2 \sqrt{2}}

Answered by mathdave last updated on 10/Sep/20

s o l u t i o n

l e t y = x^{2}, x = y^{\frac{1}{2}} a n d d x = \frac{1}{2} y^{\frac{1}{2} - 1}

I = \frac{1}{2} \int_{0}^{\infty} \frac{\sin y}{y^{\frac{1}{2}}} d y

r e c a l l t o M a z i d e n t i t y w h i c h s t a t e d a t

\int_{0}^{\infty} \frac{\sin (a x)}{x^{n}} d x = \frac{π a^{n - 1}}{2 Γ (n) \sin (\frac{π n}{2})} (a = 1, n = \frac{1}{2}, Γ (\frac{1}{2}) = \sqrt{π})

I = \frac{1}{2} \int_{0}^{\infty} \frac{\sin y}{y^{\frac{1}{2}}} d y = \frac{1}{2} ∙ \frac{π}{2 Γ (\frac{1}{2}) \sin (\frac{π}{4})}

I = \frac{1}{4} ∙ \frac{π}{\sqrt{π} \times \frac{1}{\sqrt{2}}} = \frac{\sqrt{π} \times \sqrt{2}}{4} = \frac{\sqrt{π}}{2 \sqrt{2}}

\int_{0}^{\infty} \sin (x^{2}) d x = \frac{\sqrt{π}}{2 \sqrt{2}} Q . E . D

b y m a t h d a v e (11 / 09 / 2020)

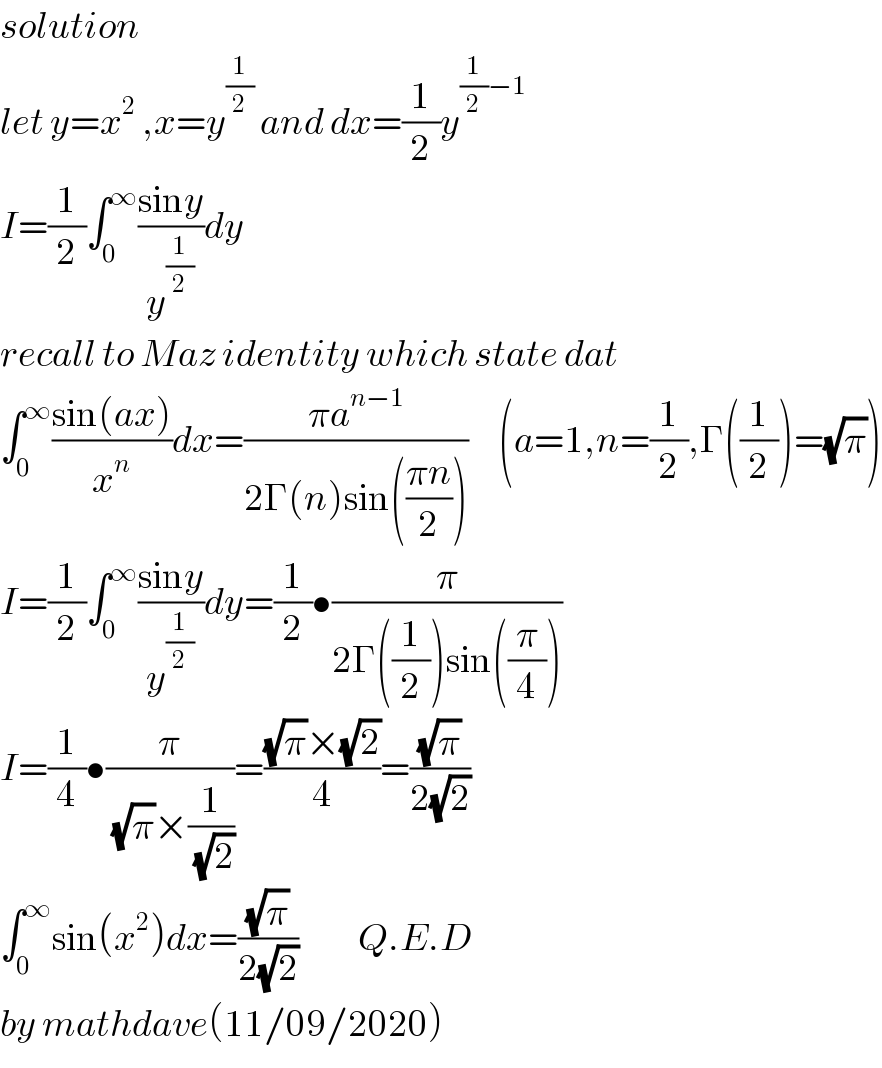
Commented by malwan last updated on 11/Sep/20

t h a n k y o u s o m u c h

Commented by Tawa11 last updated on 06/Sep/21

great sir

Answered by mathmax by abdo last updated on 10/Sep/20

let I = \int_{0}^{\infty} \cos (x^{2}) dx and J = \int_{0}^{\infty} \sin (x^{2}) dx \Rightarrow

I - iJ = \int_{0}^{\infty} e^{- {ix}^{2}} dx = \int_{0}^{\infty} e^{- {(\sqrt{i} x)}^{2}} dx =_{x \sqrt{i} = t} \int_{0}^{\infty} e^{- t^{2}} \frac{dt}{\sqrt{i}}

= \frac{1}{\sqrt{i}} \int_{0}^{\infty} e^{- t^{2}} dt = \frac{1}{e^{\frac{i π}{4}}} \times \frac{\sqrt{π}}{2} = e^{- \frac{i π}{4}} \times \sqrt{π} = \frac{\sqrt{π}}{2} {\cos (\frac{π}{4}) - isin (\frac{π}{4})}

= \frac{\sqrt{π}}{2} {\frac{1}{\sqrt{2}} - \frac{i}{\sqrt{2}}} = \frac{\sqrt{π}}{2 \sqrt{2}} - i \frac{\sqrt{π}}{2 \sqrt{2}} \Rightarrow I = J = \frac{\sqrt{π}}{2 \sqrt{2}}

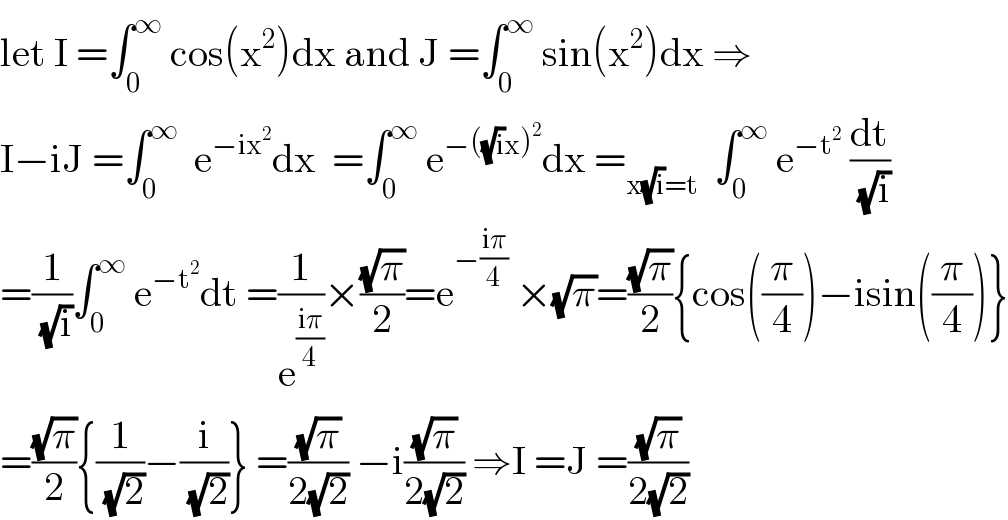
Commented by malwan last updated on 11/Sep/20

g r e a t S i r

t h a n k y o u

Facebook Tweet Pin