Question Number 128788 by bramlexs22 last updated on 10/Jan/21
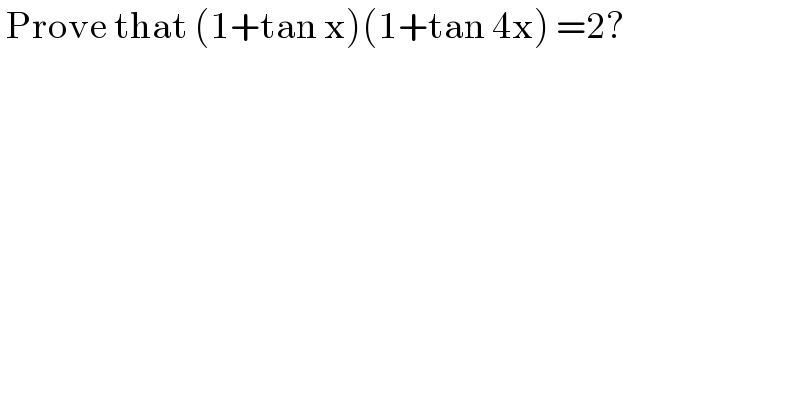
Commented by bramlexs22 last updated on 10/Jan/21
Commented by mr W last updated on 10/Jan/21

Commented by liberty last updated on 10/Jan/21
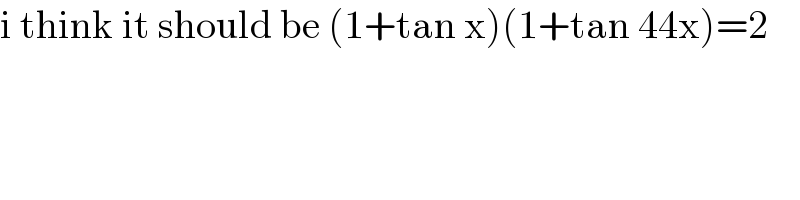
Commented by mr W last updated on 10/Jan/21
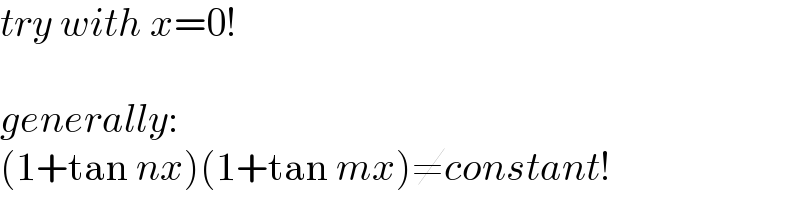
Commented by liberty last updated on 10/Jan/21

Commented by liberty last updated on 10/Jan/21

Commented by mr W last updated on 10/Jan/21
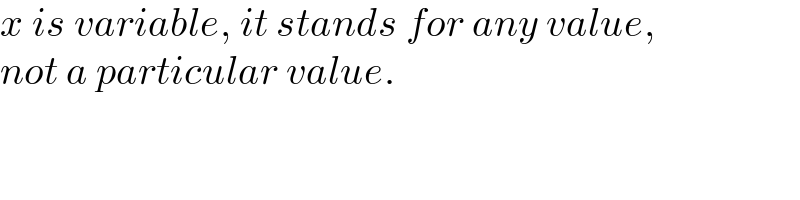
Commented by benjo_mathlover last updated on 10/Jan/21
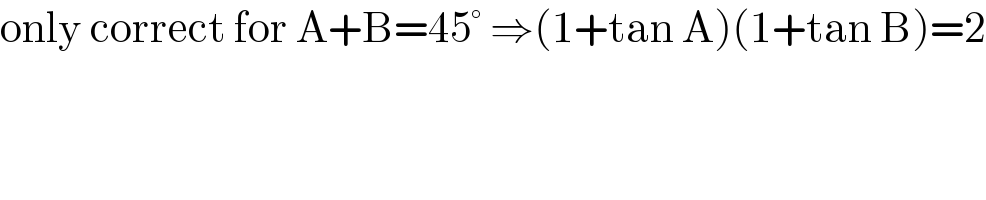
Answered by Olaf last updated on 10/Jan/21
![f(x) = (1+tanx)(1+tan4x) f(x) = (((cosx+sinx)(cos4x+sin4x))/(cosxcos4x)) f(x) = ((cosxcos4x+sinxsin4x+sinxcos4x+cosxsin4x)/(cosxcos4x)) f(x) = ((cos3x+sin5x)/(cosxcos4x)) f(x) = ((cos3x+cos((π/2)−5x))/(cosxcos4x)) f(x) = ((2cos(((3x+(π/2)−5x)/2))cos(((3x−(π/2)+5x)/2)))/(cosxcos4x)) f(x) = ((2cos(x−(π/4))cos(4x−(π/4)))/(cosxcos4x)) f(x) = 2 ⇔ cos(x−(π/4))cos(4x−(π/4)) = cosxcos4x ⇔ (1/2)[cos3x+cos(5x−(π/2))] = (1/2)[cos3x+cos5x] ⇔ cos(5x−(π/2)) = cos5x ⇔ 5x−(π/2) = ±5x+2kπ, k∈Z ⇔ x = (π/(20)) +((kπ)/(10)), k∈Z S = {(π/(20))+((kπ)/(10)), k∈Z} Numerically we can verify for example that, for k = 0 : (1+tan(π/(20)))(1+tan(π/5)) = 2](https://www.tinkutara.com/question/Q128804.png)
Commented by liberty last updated on 10/Jan/21

