Menu Close

prove-that-2-cos-2-n-1-2-cos-1-2-cos-1-2-cos-2-1-2-cos-2-2-1-2-cos-2-n-1-

Tinku Tara 0 Comments
Pin




Question Number 38557 by kunal1234523 last updated on 27/Jun/18
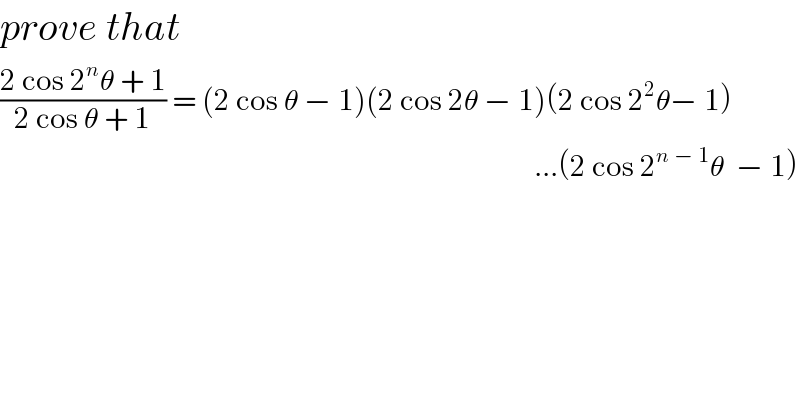
prove that ((2 cos 2^n θ + 1)/(2 cos θ + 1)) = (2 cos θ − 1)(2 cos 2θ − 1)(2 cos 2^2 θ− 1) ...(2 cos 2^(n − 1) θ − 1)
$${prove}\:{that} \\ $$$$\frac{\mathrm{2}\:\mathrm{cos}\:\mathrm{2}^{{n}} \theta\:+\:\mathrm{1}}{\mathrm{2}\:\mathrm{cos}\:\theta\:+\:\mathrm{1}}\:=\:\left(\mathrm{2}\:\mathrm{cos}\:\theta\:−\:\mathrm{1}\right)\left(\mathrm{2}\:\mathrm{cos}\:\mathrm{2}\theta\:−\:\mathrm{1}\right)\left(\mathrm{2}\:\mathrm{cos}\:\mathrm{2}^{\mathrm{2}} \theta−\:\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:…\left(\mathrm{2}\:\mathrm{cos}\:\mathrm{2}^{{n}\:−\:\mathrm{1}} \theta\:\:−\:\mathrm{1}\right) \\ $$
Answered by kunal1234523 last updated on 27/Jun/18
2 cos 2^x a + 1 =2 cos 2(2^(x−1) )a + 1 =2(2 cos^2 2^(x−1) a −1) + 1 =4 cos^2 2^(x−1) a −2 + 1 =(2 cos 2^(x−1) a)^2 − (1)^2 =(2 cos 2^(x−1) a + 1)(2 cos 2^(x−1) a −1) ........(1) L.H.S ((2 cos 2^n θ + 1)/(2 cos θ + 1)) from (1), taking x=n =(((2 cos 2^(n −1) a + 1)(2 cos 2^(n −1) a −1))/(2 cos a + 1)) now from (1), taking x=n−1 =(((2 cos 2^(n −2) a + 1)(2 cos 2^(n −2) a −1)(2 cos 2^(n −1) a −1))/(2 cos a + 1)) after some time =(((2 cos 2^(n −n) a + 1)(2 cos 2^(n −n) a −1)............(2 cos 2^(n −3) a −1)(2 cos 2^(n −2) a −1)(2 cos 2^(n −1) a −1))/(2 cos a + 1)) =(((2 cos a + 1)(2 cos a −1)(2 cos 2a −1)(2 cos 2^2 a −1)............(2 cos 2^(n −3) a −1)(2 cos 2^(n −2) a −1)(2 cos 2^(n −1) a −1))/((2 cos a + 1))) =(2 cos a −1)(2 cos 2a −1)(2 cos 2^2 a −1)............(2 cos 2^(n −3) a −1)(2 cos 2^(n −2) a −1)(2 cos 2^(n −1) a −1) proved
$$\mathrm{2}\:{cos}\:\mathrm{2}^{{x}} {a}\:+\:\mathrm{1} \\ $$$$=\mathrm{2}\:{cos}\:\mathrm{2}\left(\mathrm{2}^{{x}−\mathrm{1}} \right){a}\:+\:\mathrm{1} \\ $$$$=\mathrm{2}\left(\mathrm{2}\:{cos}^{\mathrm{2}} \:\mathrm{2}^{{x}−\mathrm{1}} {a}\:−\mathrm{1}\right)\:+\:\mathrm{1} \\ $$$$=\mathrm{4}\:{cos}^{\mathrm{2}} \:\mathrm{2}^{{x}−\mathrm{1}} {a}\:−\mathrm{2}\:+\:\mathrm{1} \\ $$$$=\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{x}−\mathrm{1}} {a}\right)^{\mathrm{2}} \:−\:\left(\mathrm{1}\right)^{\mathrm{2}} \\ $$$$=\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{x}−\mathrm{1}} {a}\:+\:\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{x}−\mathrm{1}} {a}\:−\mathrm{1}\right)\:……..\left(\mathrm{1}\right) \\ $$$$\mathrm{L}.\mathrm{H}.\mathrm{S} \\ $$$$\frac{\mathrm{2}\:\mathrm{cos}\:\mathrm{2}^{{n}} \theta\:+\:\mathrm{1}}{\mathrm{2}\:\mathrm{cos}\:\theta\:+\:\mathrm{1}} \\ $$$${from}\:\left(\mathrm{1}\right),\:{taking}\:{x}={n} \\ $$$$=\frac{\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{1}} {a}\:+\:\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{1}} {a}\:−\mathrm{1}\right)}{\mathrm{2}\:{cos}\:{a}\:+\:\mathrm{1}} \\ $$$${now}\:{from}\:\left(\mathrm{1}\right),\:{taking}\:{x}={n}−\mathrm{1} \\ $$$$=\frac{\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{2}} {a}\:+\:\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{2}} {a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{1}} {a}\:−\mathrm{1}\right)}{\mathrm{2}\:{cos}\:{a}\:+\:\mathrm{1}} \\ $$$${after}\:{some}\:{time} \\ $$$$=\frac{\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−{n}} {a}\:+\:\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−{n}} {a}\:−\mathrm{1}\right)…………\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{3}} {a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{2}} {a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{1}} {a}\:−\mathrm{1}\right)}{\mathrm{2}\:{cos}\:{a}\:+\:\mathrm{1}} \\ $$$$=\frac{\left(\mathrm{2}\:{cos}\:{a}\:+\:\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:{a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}{a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{\mathrm{2}} {a}\:−\mathrm{1}\right)…………\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{3}} {a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{2}} {a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{1}} {a}\:−\mathrm{1}\right)}{\left(\mathrm{2}\:{cos}\:{a}\:+\:\mathrm{1}\right)} \\ $$$$=\left(\mathrm{2}\:{cos}\:{a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}{a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{\mathrm{2}} {a}\:−\mathrm{1}\right)…………\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{3}} {a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{2}} {a}\:−\mathrm{1}\right)\left(\mathrm{2}\:{cos}\:\mathrm{2}^{{n}\:−\mathrm{1}} {a}\:−\mathrm{1}\right) \\ $$$$\:{proved} \\ $$
Commented by kunal1234523 last updated on 05/Jul/18
is this right sir
$${is}\:{this}\:{right}\:{sir} \\ $$
Commented by math khazana by abdo last updated on 06/Jul/18
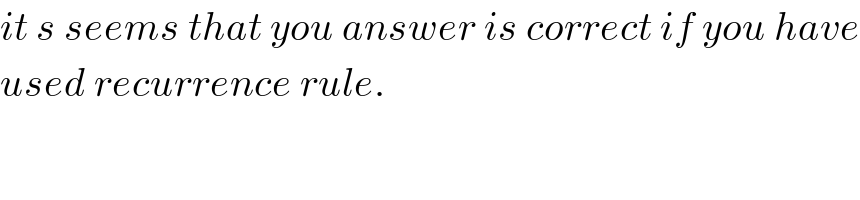
it s seems that you answer is correct if you have used recurrence rule.
$${it}\:{s}\:{seems}\:{that}\:{you}\:{answer}\:{is}\:{correct}\:{if}\:{you}\:{have} \\ $$$${used}\:{recurrence}\:{rule}. \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *