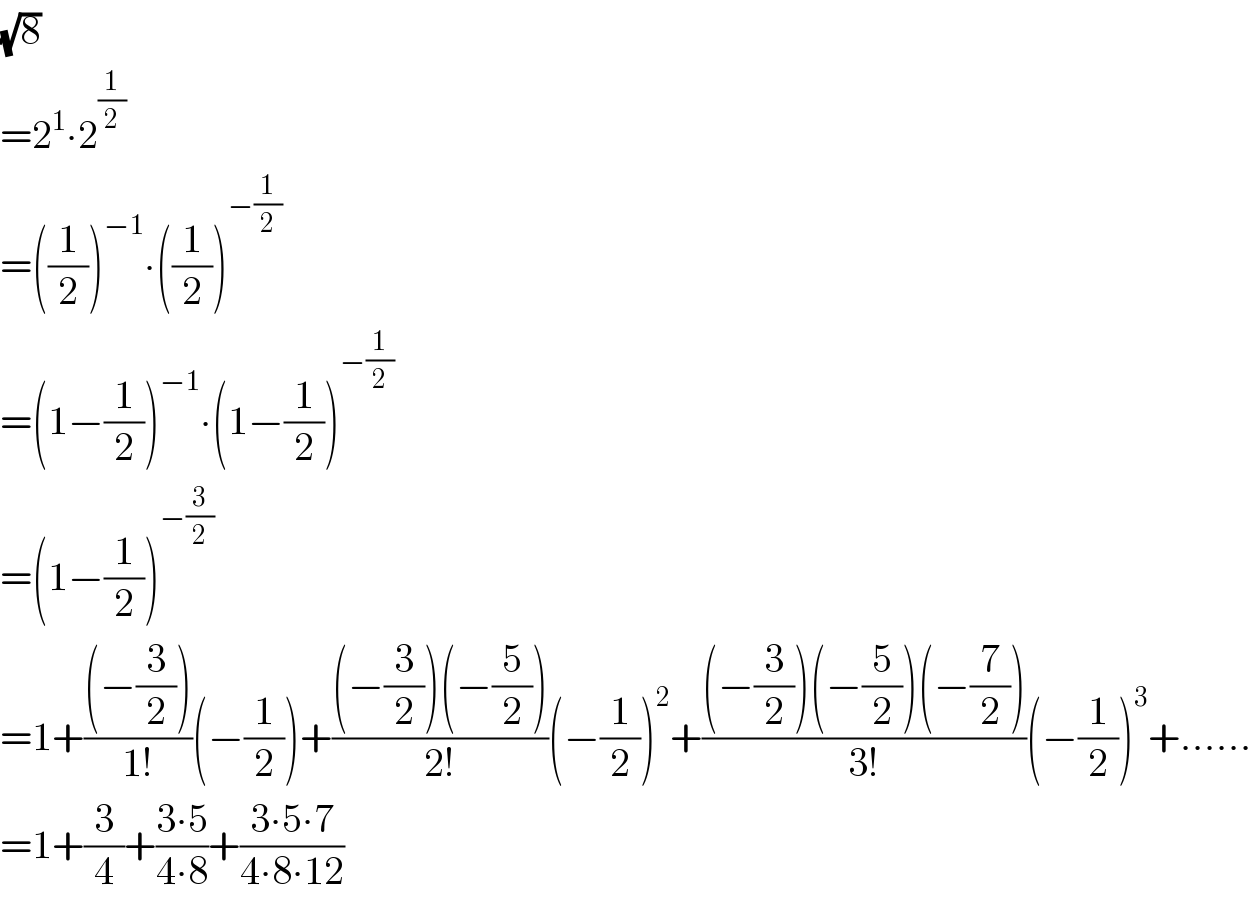Prove-that-8-1-3-4-3-5-4-8-3-5-7-4-8-12-

Question Number 107187 by Dwaipayan Shikari last updated on 09/Aug/20

Prove that

\sqrt{8} = 1 + \frac{3}{4} + \frac{3.5}{4.8} + \frac{3.5.7}{4.8.12} + \dots \dots

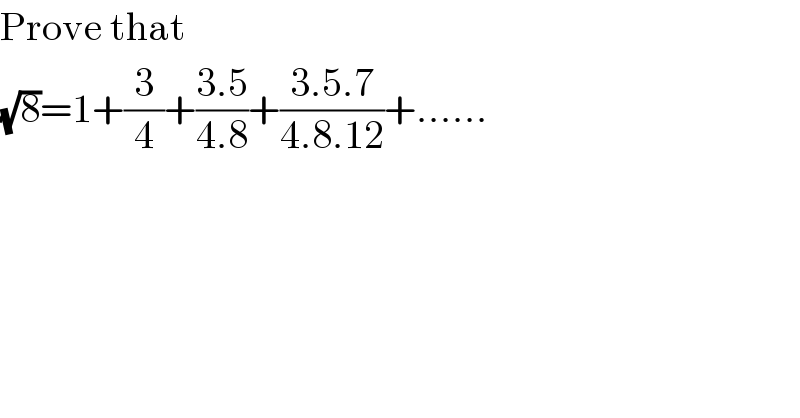
Commented by john santu last updated on 09/Aug/20

\sqrt{8} = \sqrt{9 - 1} = {(9 - 1)}^{\frac{1}{2}}

consider {(9 - 1)}^{n} = \underset{k = 0}{\sum^{n}} C_{k}^{n} {(9)}^{n - k} {(- 1)}^{k}

= {(9)}^{n} + n {(9)}^{n - 1} (- 1) + \frac{n . (n - 1)}{2!} {(9)}^{n - 2} {(- 1)}^{2}

+ \frac{n (n - 1) (n - 2)}{3!} {(9)}^{n - 3} {(- 1)}^{3} + \dots

put n = \frac{1}{2}

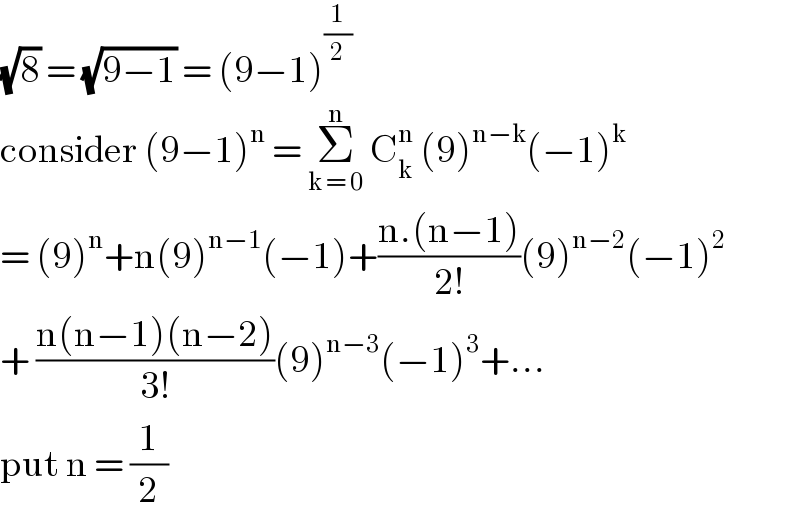
Commented by Dwaipayan Shikari last updated on 09/Aug/20

{(1 + 1)}^{\frac{3}{2}} = 1 + \frac{3}{2} + \frac{3}{8} + (- \frac{3 .}{8.6}) + . . \frac{3}{16.24} + \dots

= 1 + \frac{3}{4} + \frac{3}{4} + \frac{3}{8} - \frac{3}{8.6} + . .

= 1 + \frac{3}{4} + \frac{3.12}{4.8} - \frac{3}{8.6} + .

= 1 + \frac{3}{4} + \frac{3.5}{4.8} + \frac{7}{4.8} - \frac{3}{8.6} + . .

= 1 + \frac{3}{4} + \frac{3.5}{4.8} + \frac{30}{4.8.6} + \frac{3}{16.24} + . .

= 1 + \frac{3}{4} + \frac{3.5}{4.8} + \frac{3.5.4}{4.8.12} + \frac{3}{16.24} + . .

= 1 + \frac{3}{4} + \frac{3.5}{4.8} + \frac{3.5.7}{4.8.12} - \frac{3.5.3}{4.8.6} + \frac{3}{16.24} + \dots

= 1 + \frac{3}{4} + \frac{3.5}{4.8} + \frac{3.5.7}{4.8.12} + \dots . . + C

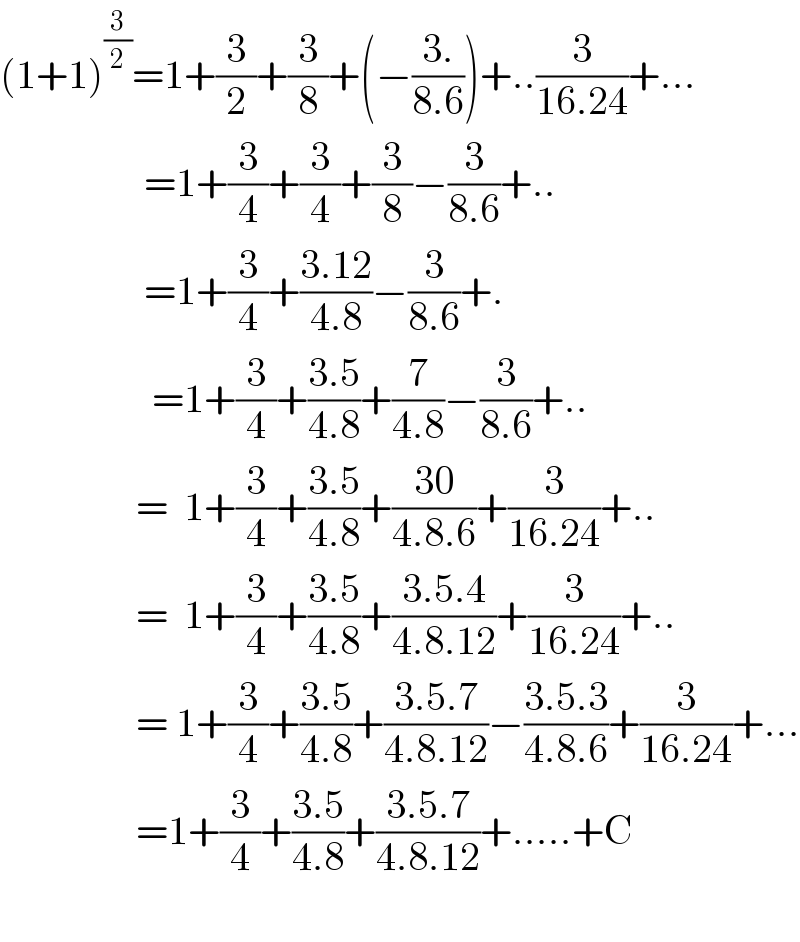
Commented by ZiYangLee last updated on 10/Aug/20

Why \frac{3 \cdot 12}{4 \cdot 8} = \frac{3 \cdot 5}{4 \cdot 8} + \frac{7}{4 \cdot 8} ? ? ?

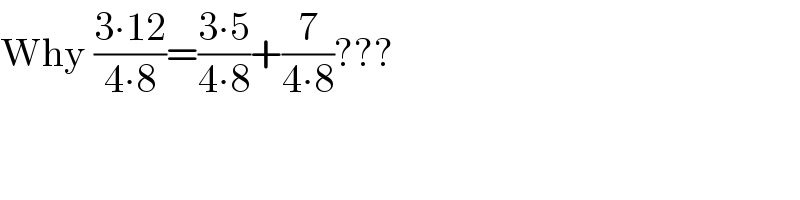
Answered by ZiYangLee last updated on 10/Aug/20

\sqrt{8}

= 2^{1} \cdot 2^{\frac{1}{2}}

= {(\frac{1}{2})}^{- 1} \cdot {(\frac{1}{2})}^{- \frac{1}{2}}

= {(1 - \frac{1}{2})}^{- 1} \cdot {(1 - \frac{1}{2})}^{- \frac{1}{2}}

= {(1 - \frac{1}{2})}^{- \frac{3}{2}}

= 1 + \frac{(- \frac{3}{2})}{1!} (- \frac{1}{2}) + \frac{(- \frac{3}{2}) (- \frac{5}{2})}{2!} {(- \frac{1}{2})}^{2} + \frac{(- \frac{3}{2}) (- \frac{5}{2}) (- \frac{7}{2})}{3!} {(- \frac{1}{2})}^{3} + \dots \dots

= 1 + \frac{3}{4} + \frac{3 \cdot 5}{4 \cdot 8} + \frac{3 \cdot 5 \cdot 7}{4 \cdot 8 \cdot 12}