Prove-that-if-a-b-c-Z-and-a-2-b-2-c-2-then-3-ab-

Question Number 44584 by Joel578 last updated on 01/Oct/18

Prove that if a, b, c \in Z and a^{2} + b^{2} = c^{2}, then

3 ∣ a b

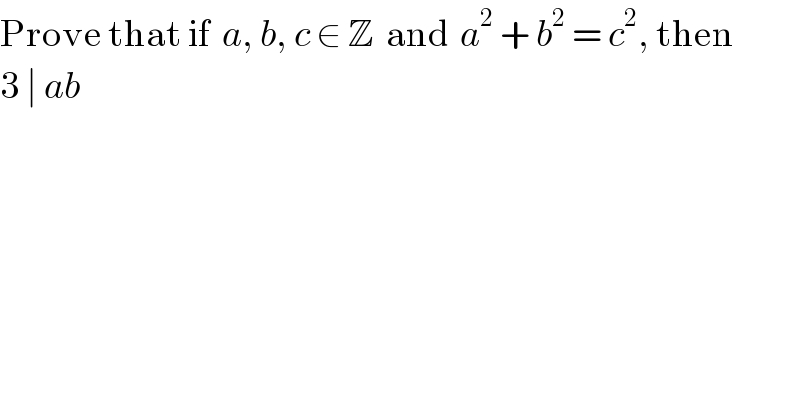
Commented by ajfour last updated on 02/Oct/18

t h e n 3 ∣ a b m e a n s ?! . .

Commented by rahul 19 last updated on 02/Oct/18

3 d i v i d e s a b \dots . .

{I t}^{'} s j u s t a n o t a t i o n

Commented by ajfour last updated on 02/Oct/18

t h a n k s r a h u l .

Commented by Joel578 last updated on 03/Oct/18

Yes, 3 ∣ a b means there is such number x that

satisfy a b = 3 x

Commented by Joel578 last updated on 03/Oct/18

I^{'} m having trouble how to start the proof

Answered by arcana last updated on 03/Oct/18

podemos ver solamente para el caso

de que a, b, c sea una s o l u c i o n p r i m i t i v a,

es decir (a, b, c) = 1, ya que las soluciones primitivas

pueden generar los demas casos .

A hora solo uno de los enteros a, b debe

ser impar, pues si los lo son e n t o n c e s a^{2} + b^{2} es

de la forma 4 k + 2 pero todo cuadrado

perfecto es de la forma 4 k o 4 k + 1 (\to\leftarrow) .

B ajo estas condiciones podemos usar el

siguiente teorema :

S i a, b, c \in Z e s u n a s o l u c i o n p r i m i t i v a

d o n d e a e s i m p a r p a r a l a e c u a c i o n

a^{2} + b^{2} = c^{2} s i i e x i s t e n e n t e r o s x, y

t a l e s q u e :

a = x^{2} - y^{2}

\Rightarrow c = x^{2} + y^{2}

b = 2 x y

c o n (x, y) = 1, x > y, x e y t i e n e n d i s t i n t a

p a r i d a d .

p o r l o a n t e r i o r t e n e m o s a b = 2 x y (x^{2} - y^{2}) .

y e s t o s e r e d u c e e n v e r s i 3 ∣ x \lor 3 ∣ y \lor

3 ∣ (x^{2} - y^{2}) .

s i 3 ∣ x \lor 3 ∣ y e n t o n c e a e s t a h e c h o .

s i 3 n o d i v i d e a x n i y, e n t o n c e s

x, y s o n d e l a f o r m a 3 k + 1 o 3 k + 2, k e n t e r o

p a r a k_{0}, k_{1} \in Z

c a s o 1 : s i x = 3 k_{0} + 1, y = 3 k_{1} + 1

l u e g o x^{2} - y^{2} = 3 (3 k_{0}^{2} + 2 k_{0} - 3 k_{1}^{2} - 2 k_{1})

e s t o e s 3 ∣ (x^{2} - y^{2}) \Rightarrow 3 ∣ a b

c a s o 2 : s i x = 3 k_{0} + 1, y = 3 k_{1} + 2

\Rightarrow x^{2} - y^{2} = 3 (3 k_{0}^{2} + 2 k_{0} - 3 k_{1}^{2} - 2 k_{1} - 1)

l u e g o 3 ∣ (x^{2} - y^{2}) \Rightarrow 3 ∣ a b

c a s o 3 : s i x = 3 k_{0} + 2, y = 3 k_{1} + 1

e s s i m i l a r a l c a s o 2

c a s o 4 : s i x = 3 k_{0} + 2, y = 3 k_{1} + 2

s i m i l a r a l c a s o 1 .

La demostracion del teorema se puede encontrar en el libro : teoria de numeros,

Rubiano

Facebook Tweet Pin