prove-that-ln-z-0-1-z-1-1-t-z-1-dt-

Question Number 54371 by maxmathsup by imad last updated on 02/Feb/19

p r o v e t h a t l n (z) = \int_{0}^{1} \frac{z - 1}{1 + t (z - 1)} d t .

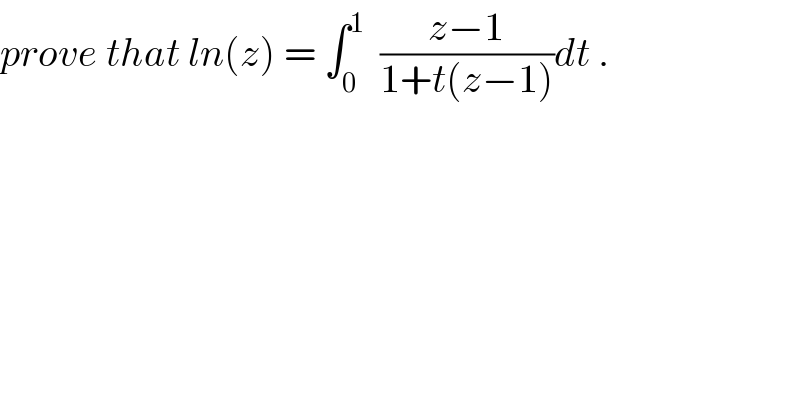
Commented by maxmathsup by imad last updated on 02/Feb/19

z i s a c o m p l e x n u m b e r .

Answered by Smail last updated on 02/Feb/19

l e t u = t (z - 1) \Rightarrow d u = (z - 1) d t

\int_{0}^{1} \frac{z - 1}{1 + t (z - 1)} d t = \int_{0}^{z - 1} \frac{d u}{1 + u} = {[l n ∣ 1 + u ∣]}_{0}^{z - 1}

= l n ∣ 1 + z - 1 ∣= l n ∣ z ∣

Commented by Abdo msup. last updated on 03/Feb/19

s i r w h a t d o y o u w o r k i n u s a \dots

Commented by Smail last updated on 03/Feb/19

I a m s t i l l s t u d y i n g a n d m y m a j o r i s

e l e c t r i c a l e n g i n e e r i n g

Commented by rahul 19 last updated on 03/Feb/19

w o w! w h i c h u n i v e r s i t y ?

Commented by maxmathsup by imad last updated on 03/Feb/19

g o o d l u c k s i r S m a i l \dots i h o p p e t o v i s i t u s a s o m e d a y s \dots

Commented by Smail last updated on 03/Feb/19

T h a n k y o u

Facebook Tweet Pin