Question Number 85603 by M±th+et£s last updated on 23/Mar/20
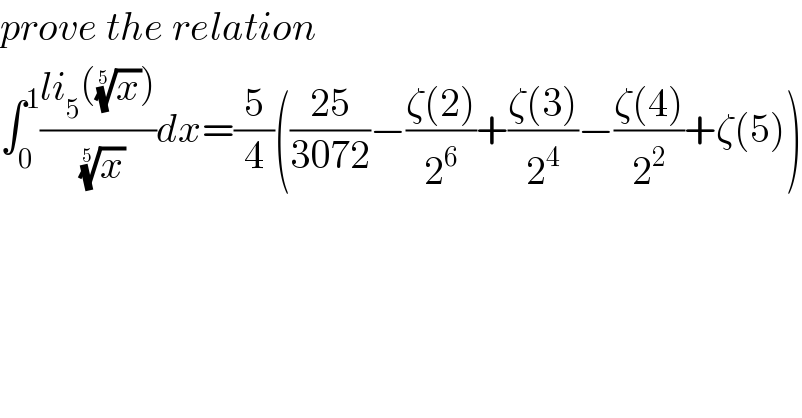
$${prove}\:{the}\:{relation} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{li}_{\mathrm{5}} \left(\sqrt[{\mathrm{5}}]{{x}}\right)}{\:\sqrt[{\mathrm{5}}]{{x}}}{dx}=\frac{\mathrm{5}}{\mathrm{4}}\left(\frac{\mathrm{25}}{\mathrm{3072}}−\frac{\zeta\left(\mathrm{2}\right)}{\mathrm{2}^{\mathrm{6}} }+\frac{\zeta\left(\mathrm{3}\right)}{\mathrm{2}^{\mathrm{4}} }−\frac{\zeta\left(\mathrm{4}\right)}{\mathrm{2}^{\mathrm{2}} }+\zeta\left(\mathrm{5}\right)\right) \\ $$
