Question Number 63218 by ajfour last updated on 30/Jun/19
![Q.63108 (A check) eq. of ellipse (x^2 /4)+y^2 =1 Inscribed equilateral △ABC of side s=((16(√6))/( (√(365)))) Do these points satisfy for A, B, C ? A((4/( (√(365)))), ((19)/( (√(365))))) ; B[−(((20+8(√3)))/( (√(365)))), ((8(√3)−5)/( (√(365))))] C[−(((20−8(√3)))/( (√(365)))) , −(((5+8(√3)))/( (√(365))))] θ=45°](https://www.tinkutara.com/question/Q63218.png)
$${Q}.\mathrm{63108}\:\:\:\left({A}\:{check}\right) \\ $$$${eq}.\:{of}\:{ellipse} \\ $$$$\frac{{x}^{\mathrm{2}} }{\mathrm{4}}+{y}^{\mathrm{2}} =\mathrm{1} \\ $$$${Inscribed}\:{equilateral}\:\bigtriangleup{ABC} \\ $$$${of}\:{side}\:\boldsymbol{{s}}=\frac{\mathrm{16}\sqrt{\mathrm{6}}}{\:\sqrt{\mathrm{365}}} \\ $$$${Do}\:{these}\:{points}\:{satisfy}\:{for} \\ $$$${A},\:{B},\:{C}\:? \\ $$$${A}\left(\frac{\mathrm{4}}{\:\sqrt{\mathrm{365}}},\:\frac{\mathrm{19}}{\:\sqrt{\mathrm{365}}}\right)\:\:\:;\:\: \\ $$$${B}\left[−\frac{\left(\mathrm{20}+\mathrm{8}\sqrt{\mathrm{3}}\right)}{\:\sqrt{\mathrm{365}}},\:\frac{\mathrm{8}\sqrt{\mathrm{3}}−\mathrm{5}}{\:\sqrt{\mathrm{365}}}\right] \\ $$$${C}\left[−\frac{\left(\mathrm{20}−\mathrm{8}\sqrt{\mathrm{3}}\right)}{\:\sqrt{\mathrm{365}}}\:,\:−\frac{\left(\mathrm{5}+\mathrm{8}\sqrt{\mathrm{3}}\right)}{\:\sqrt{\mathrm{365}}}\right] \\ $$$$\:\theta=\mathrm{45}° \\ $$
Commented by Tinkutara@ last updated on 02/Jul/19
hello Mr W ! remember me?
btw are you active on WhatsApp, Facebook, Snapchat, Twitter, LinkedIn, Pinterest ?
p.s- recently, my Vacations got over. ������ ( went to phi phi Island!!! { Thailand})
Commented by ajfour last updated on 01/Jul/19
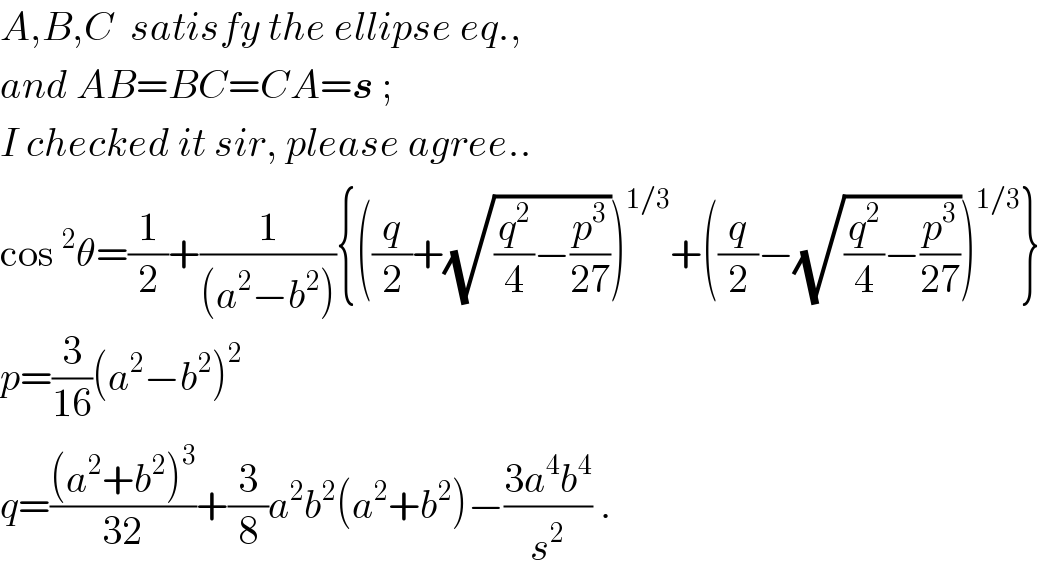
$${A},{B},{C}\:\:{satisfy}\:{the}\:{ellipse}\:{eq}., \\ $$$${and}\:{AB}={BC}={CA}=\boldsymbol{{s}}\:; \\ $$$${I}\:{checked}\:{it}\:{sir},\:{please}\:{agree}.. \\ $$$$\mathrm{cos}\:^{\mathrm{2}} \theta=\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)}\left\{\left(\frac{{q}}{\mathrm{2}}+\sqrt{\frac{{q}^{\mathrm{2}} }{\mathrm{4}}−\frac{{p}^{\mathrm{3}} }{\mathrm{27}}}\right)^{\mathrm{1}/\mathrm{3}} +\left(\frac{{q}}{\mathrm{2}}−\sqrt{\frac{{q}^{\mathrm{2}} }{\mathrm{4}}−\frac{{p}^{\mathrm{3}} }{\mathrm{27}}}\right)^{\mathrm{1}/\mathrm{3}} \right\} \\ $$$${p}=\frac{\mathrm{3}}{\mathrm{16}}\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)^{\mathrm{2}} \\ $$$${q}=\frac{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)^{\mathrm{3}} }{\mathrm{32}}+\frac{\mathrm{3}}{\mathrm{8}}{a}^{\mathrm{2}} {b}^{\mathrm{2}} \left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)−\frac{\mathrm{3}{a}^{\mathrm{4}} {b}^{\mathrm{4}} }{{s}^{\mathrm{2}} }\:. \\ $$
Commented by kaivan.ahmadi last updated on 01/Jul/19

$${hi}\:{mr}\:{w}\:{where}\:{are}\:{you}\:{from}? \\ $$$${do}\:{you}\:{have}\:{instagram}\:{page}? \\ $$
Commented by mr W last updated on 01/Jul/19

$${yes}\:{sir}!\:{after}\:{new}\:{consideration}\:{now} \\ $$$${i}\:{think}\:{solutions}\:{other}\:{than} \\ $$$$\theta=\mathrm{0}°\:{are}\:{possible}.\:{i}'{ll}\:{try}\:{to}\:{find}\:{out} \\ $$$${the}\:{mistakes}\:{in}\:{my}\:{working}. \\ $$
Commented by Tinkutara@ last updated on 02/Jul/19
thanks sir!
I wish the same for you...
Commented by ajfour last updated on 04/Jul/19
Tinkutara, how did you perform in jee advanced, where are you probably going to join.
Commented by Tinkutara@ last updated on 03/Jul/19
my paper went okayish.
Unfortunately , I 'm not getting CSE in top 5 IITS ( due to reservation , sigh!). So Most probably I will be going to Bits Pilani CSE . ( since it's better than rest iits ) .
- At the end of the day , I'm thankful to you and rest of the members of this awesome community to provide elegant solutions to the silly doubts thrown at you guys :P
Commented by Tinkutara last updated on 18/Jul/19
@ajfour Sir:
I got a rank between 5900-6k in advanced and got IIT KGP.
I am also thankful to all of them who solved my doubts on this platform which meant a lot to me!
But @above is not my id.
