Question Number 36031 by kami last updated on 27/May/18
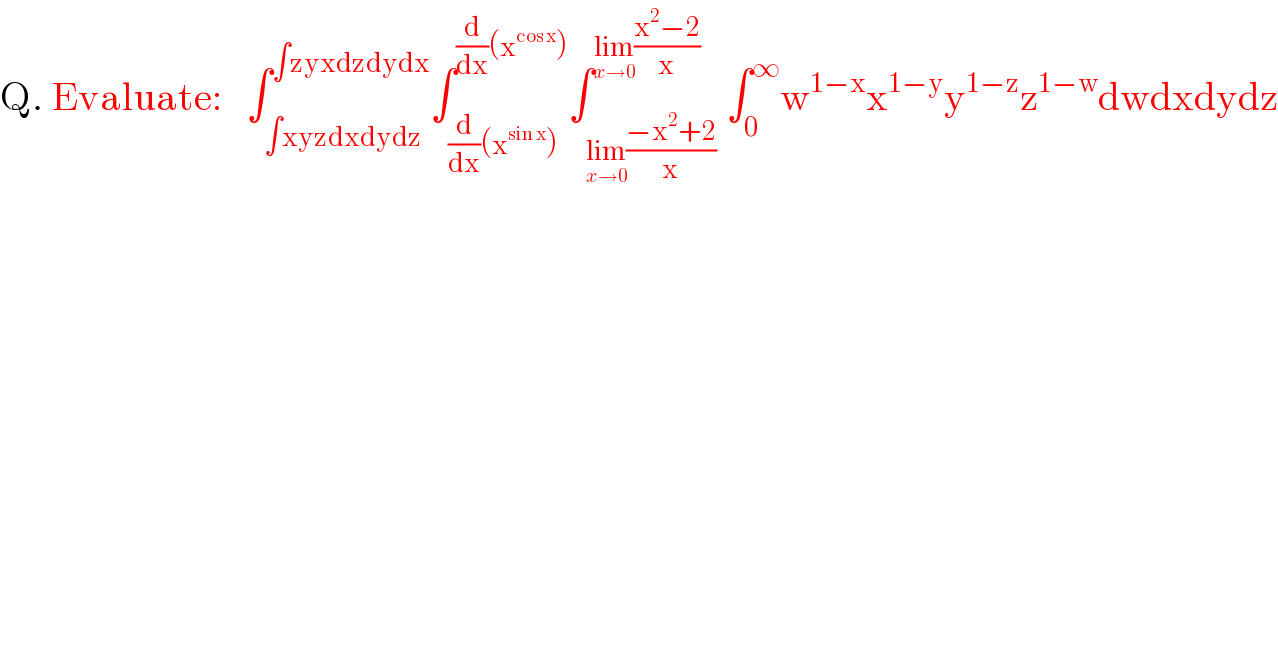
$$\mathrm{Q}.\:\mathrm{Evaluate}:\:\:\:\int_{\int\mathrm{xyzdxdydz}} ^{\int\mathrm{zyxdzdydx}} \int_{\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^{\mathrm{sin}\:\mathrm{x}} \right)} ^{\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^{\mathrm{cos}\:\mathrm{x}} \right)} \int_{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\mathrm{x}^{\mathrm{2}} +\mathrm{2}}{\mathrm{x}}} ^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{2}}{\mathrm{x}}} \int_{\mathrm{0}} ^{\infty} \mathrm{w}^{\mathrm{1}−\mathrm{x}} \mathrm{x}^{\mathrm{1}−\mathrm{y}} \mathrm{y}^{\mathrm{1}−\mathrm{z}} \mathrm{z}^{\mathrm{1}−\mathrm{w}} \mathrm{dwdxdydz} \\ $$
