Question Number 54858 by Otchere Abdullai last updated on 13/Feb/19
$$\left({Q}\mathrm{1}\right)\:{The}\:{expansion}\:\left({x}−\frac{\mathrm{1}}{\mathrm{3}{x}}\right)^{\mathrm{12}} \\ $$$$\left({i}\right)\:{find}\:{the}\:{term}\:{independent}\:{of}\:{x} \\ $$$$\left({ii}\right)\:{find}\:{the}\:{term}\:{x}^{\mathrm{4}} \\ $$$$\left({Q}\mathrm{2}\right)\:{There}\:{are}\:{some}\:{nut}\:{in}\:{a}\:{bag}.\:{when} \\ $$$$\:\mathrm{2}\:{ounces}\:{of}\:{peanut}\:{are}\:{added}\:{to}\:{the} \\ $$$${mixture},\:{the}\:{percentage}\:{of}\:{peanut}\: \\ $$$${becomes}\:\mathrm{20\%}\:.\:{Richard}\:\:{added}\:\:\mathrm{2}\: \\ $$$${ounces}\:{of}\:{cashew}\:{to}\:{the}\:{mixture}\:{and} \\ $$$${the}\:{percentage}\:{of}\:{cashew}\:{nut}\:{was}\: \\ $$$$\mathrm{33}.\mathrm{33\%}\:.\:{find}\:{the}\:{percentage}\:{of}\: \\ $$$${cashew}\:{nut}\:{that}\:{were}\:{there}\:{initially}. \\ $$$${please}\:{sir}\:{help} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 13/Feb/19
![1)let (r+1)th term independent of x [i^ e x^0 ] 12c_r (x)^(12−r) (−(1/(3x)))^r 12c_r ×x^(12−r) ×(−1)^r ×(1/(3^r x^r )) 12c_r ×(−1)^r ×(1/3^r )×x^(12−r−r) so x^(12−2r) =x^0 →2r=12 so r=6 x independent term is 12c_6 ×(−1)^6 ×(1/3^6 )×x^(12−6−6) =((12!)/(6!6!))×1×(1/3^6 ) pls calculate by your self... next question x^4 x^(12−2r) =x^4 →2r=8 r=4 so required answer is 12c_4 ×(−1)^4 ×(1/3^4 )×x^(12−4−4) 12c_4 ×1×(1/3^4 )×x^4 →calculate the value by your self](https://www.tinkutara.com/question/Q54862.png)
$$\left.\mathrm{1}\right){let}\:\left({r}+\mathrm{1}\right){th}\:{term}\:{independent}\:{of}\:{x}\:\left[\bar {{i}e}\:{x}^{\mathrm{0}} \right] \\ $$$$\mathrm{12}{c}_{{r}} \left({x}\right)^{\mathrm{12}−{r}} \left(−\frac{\mathrm{1}}{\mathrm{3}{x}}\right)^{{r}} \\ $$$$\mathrm{12}{c}_{{r}} ×{x}^{\mathrm{12}−{r}} ×\left(−\mathrm{1}\right)^{{r}} ×\frac{\mathrm{1}}{\mathrm{3}^{{r}} {x}^{{r}} } \\ $$$$\mathrm{12}{c}_{{r}} ×\left(−\mathrm{1}\right)^{{r}} ×\frac{\mathrm{1}}{\mathrm{3}^{{r}} }×{x}^{\mathrm{12}−{r}−{r}} \\ $$$${so}\:{x}^{\mathrm{12}−\mathrm{2}{r}} ={x}^{\mathrm{0}} \rightarrow\mathrm{2}{r}=\mathrm{12}\:\:\:{so}\:{r}=\mathrm{6} \\ $$$${x}\:{independent}\:{term}\:{is} \\ $$$$ \\ $$$$\mathrm{12}{c}_{\mathrm{6}} ×\left(−\mathrm{1}\right)^{\mathrm{6}} ×\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{6}} }×{x}^{\mathrm{12}−\mathrm{6}−\mathrm{6}} \\ $$$$=\frac{\mathrm{12}!}{\mathrm{6}!\mathrm{6}!}×\mathrm{1}×\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{6}} }\: \\ $$$${pls}\:{calculate}\:{by}\:{your}\:{self}… \\ $$$${next}\:{question}\:{x}^{\mathrm{4}} \\ $$$${x}^{\mathrm{12}−\mathrm{2}{r}} ={x}^{\mathrm{4}} \rightarrow\mathrm{2}{r}=\mathrm{8}\:\:\:{r}=\mathrm{4} \\ $$$${so}\:{required}\:{answer}\:{is}\: \\ $$$$\mathrm{12}{c}_{\mathrm{4}} ×\left(−\mathrm{1}\right)^{\mathrm{4}} ×\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{4}} }×{x}^{\mathrm{12}−\mathrm{4}−\mathrm{4}} \\ $$$$\mathrm{12}{c}_{\mathrm{4}} ×\mathrm{1}×\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{4}} }×{x}^{\mathrm{4}} \rightarrow{calculate}\:{the}\:{value}\:{by}\:{your}\:{self} \\ $$$$ \\ $$
Commented by Otchere Abdullai last updated on 13/Feb/19
$${Thank}\:{you}\:{profesor}\:{chaudhunry}\:{am} \\ $$$${much}\:{greatful} \\ $$
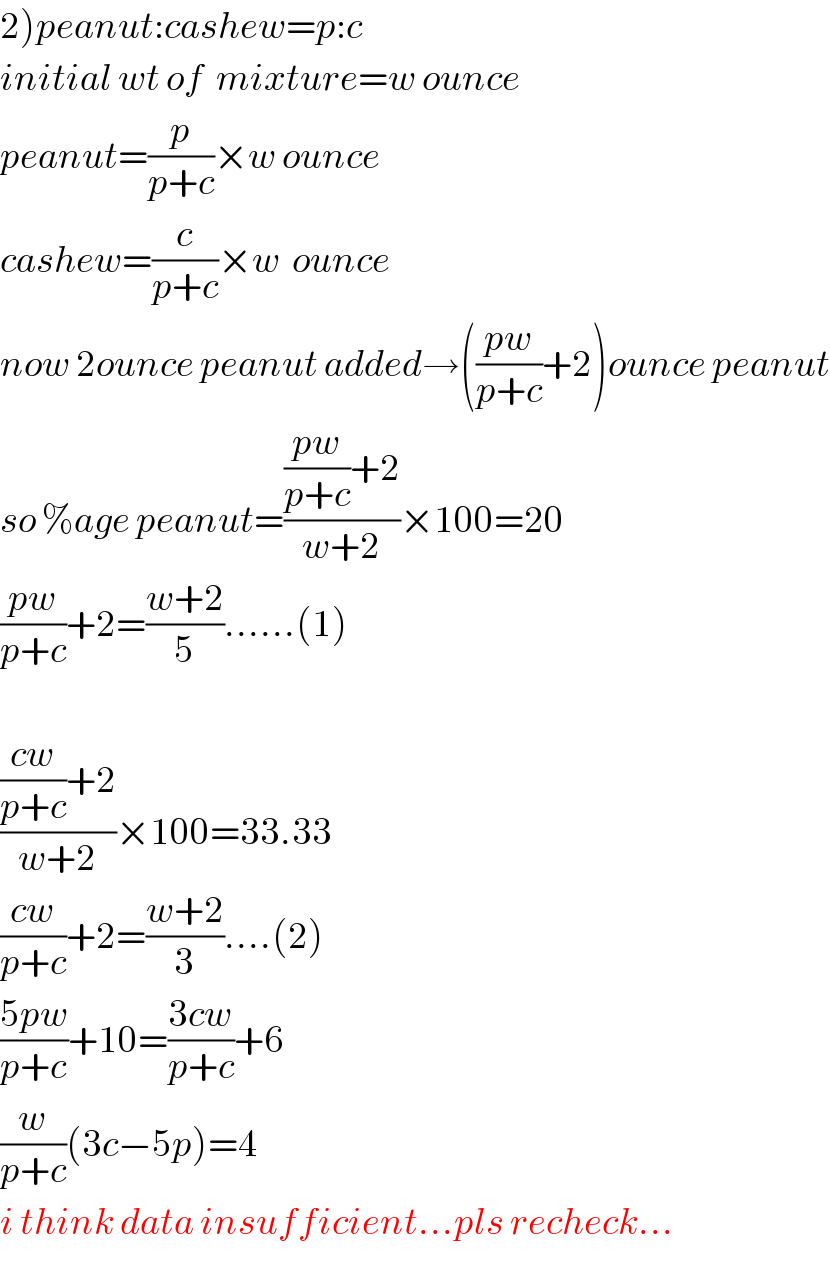
Answered by tanmay.chaudhury50@gmail.com last updated on 13/Feb/19
$$\left.\mathrm{2}\right){peanut}:{cashew}={p}:{c} \\ $$$${initial}\:{wt}\:{of}\:\:{mixture}={w}\:{ounce} \\ $$$${peanut}=\frac{{p}}{{p}+{c}}×{w}\:{ounce} \\ $$$${cashew}=\frac{{c}}{{p}+{c}}×{w}\:\:{ounce} \\ $$$${now}\:\mathrm{2}{ounce}\:{peanut}\:{added}\rightarrow\left(\frac{{pw}}{{p}+{c}}+\mathrm{2}\right){ounce}\:{peanut} \\ $$$${so}\:\%{age}\:{peanut}=\frac{\frac{{pw}}{{p}+{c}}+\mathrm{2}}{{w}+\mathrm{2}}×\mathrm{100}=\mathrm{20} \\ $$$$\frac{{pw}}{{p}+{c}}+\mathrm{2}=\frac{{w}+\mathrm{2}}{\mathrm{5}}……\left(\mathrm{1}\right) \\ $$$$ \\ $$$$\frac{\frac{{cw}}{{p}+{c}}+\mathrm{2}}{{w}+\mathrm{2}}×\mathrm{100}=\mathrm{33}.\mathrm{33} \\ $$$$\frac{{cw}}{{p}+{c}}+\mathrm{2}=\frac{{w}+\mathrm{2}}{\mathrm{3}}….\left(\mathrm{2}\right) \\ $$$$\frac{\mathrm{5}{pw}}{{p}+{c}}+\mathrm{10}=\frac{\mathrm{3}{cw}}{{p}+{c}}+\mathrm{6} \\ $$$$\frac{{w}}{{p}+{c}}\left(\mathrm{3}{c}−\mathrm{5}{p}\right)=\mathrm{4} \\ $$$${i}\:{think}\:{data}\:{insufficient}…{pls}\:{recheck}… \\ $$