Question Number 100703 by bramlex last updated on 28/Jun/20
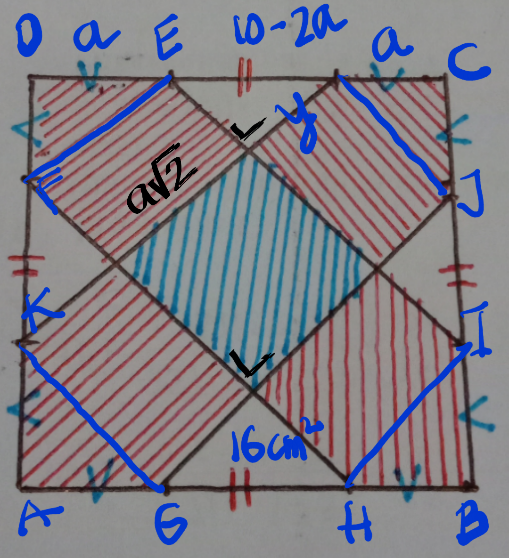
Commented by bramlex last updated on 28/Jun/20
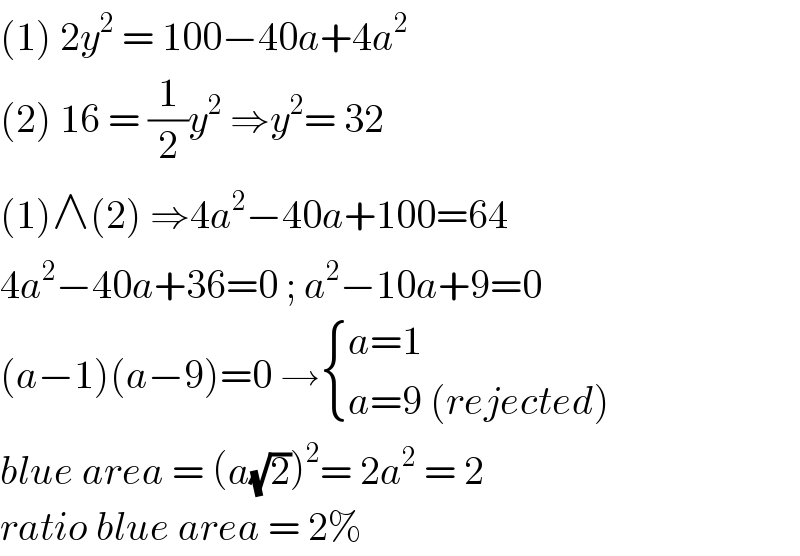
$$\left(\mathrm{1}\right)\:\mathrm{2}{y}^{\mathrm{2}} \:=\:\mathrm{100}−\mathrm{40}{a}+\mathrm{4}{a}^{\mathrm{2}} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{16}\:=\:\frac{\mathrm{1}}{\mathrm{2}}{y}^{\mathrm{2}} \:\Rightarrow{y}^{\mathrm{2}} =\:\mathrm{32} \\ $$$$\left(\mathrm{1}\right)\wedge\left(\mathrm{2}\right)\:\Rightarrow\mathrm{4}{a}^{\mathrm{2}} −\mathrm{40}{a}+\mathrm{100}=\mathrm{64}\: \\ $$$$\mathrm{4}{a}^{\mathrm{2}} −\mathrm{40}{a}+\mathrm{36}=\mathrm{0}\:;\:{a}^{\mathrm{2}} −\mathrm{10}{a}+\mathrm{9}=\mathrm{0} \\ $$$$\left({a}−\mathrm{1}\right)\left({a}−\mathrm{9}\right)=\mathrm{0}\:\rightarrow\begin{cases}{{a}=\mathrm{1}}\\{{a}=\mathrm{9}\:\left({rejected}\right)}\end{cases} \\ $$$${blue}\:{area}\:=\:\left({a}\sqrt{\mathrm{2}}\right)^{\mathrm{2}} =\:\mathrm{2}{a}^{\mathrm{2}} \:=\:\mathrm{2} \\ $$$${ratio}\:{blue}\:{area}\:=\:\mathrm{2\%} \\ $$
Commented by bemath last updated on 28/Jun/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
