Menu Close

Question-102260

Tinku Tara 0 Comments
Pin




Question Number 102260 by ajfour last updated on 07/Jul/20
Commented by ajfour last updated on 07/Jul/20
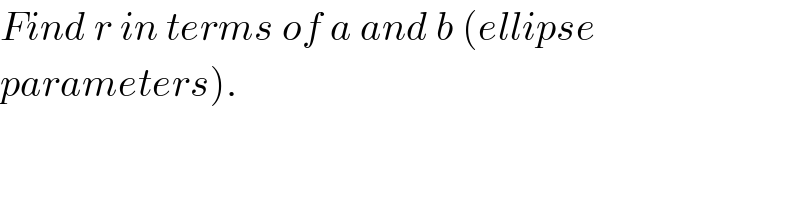
Find r in terms of a and b (ellipse parameters).
$${Find}\:{r}\:{in}\:{terms}\:{of}\:{a}\:{and}\:{b}\:\left({ellipse}\right. \\ $$$$\left.{parameters}\right). \\ $$
Commented by mr W last updated on 07/Jul/20
won′t be easy sir...
$${won}'{t}\:{be}\:{easy}\:{sir}… \\ $$
Answered by mr W last updated on 07/Jul/20
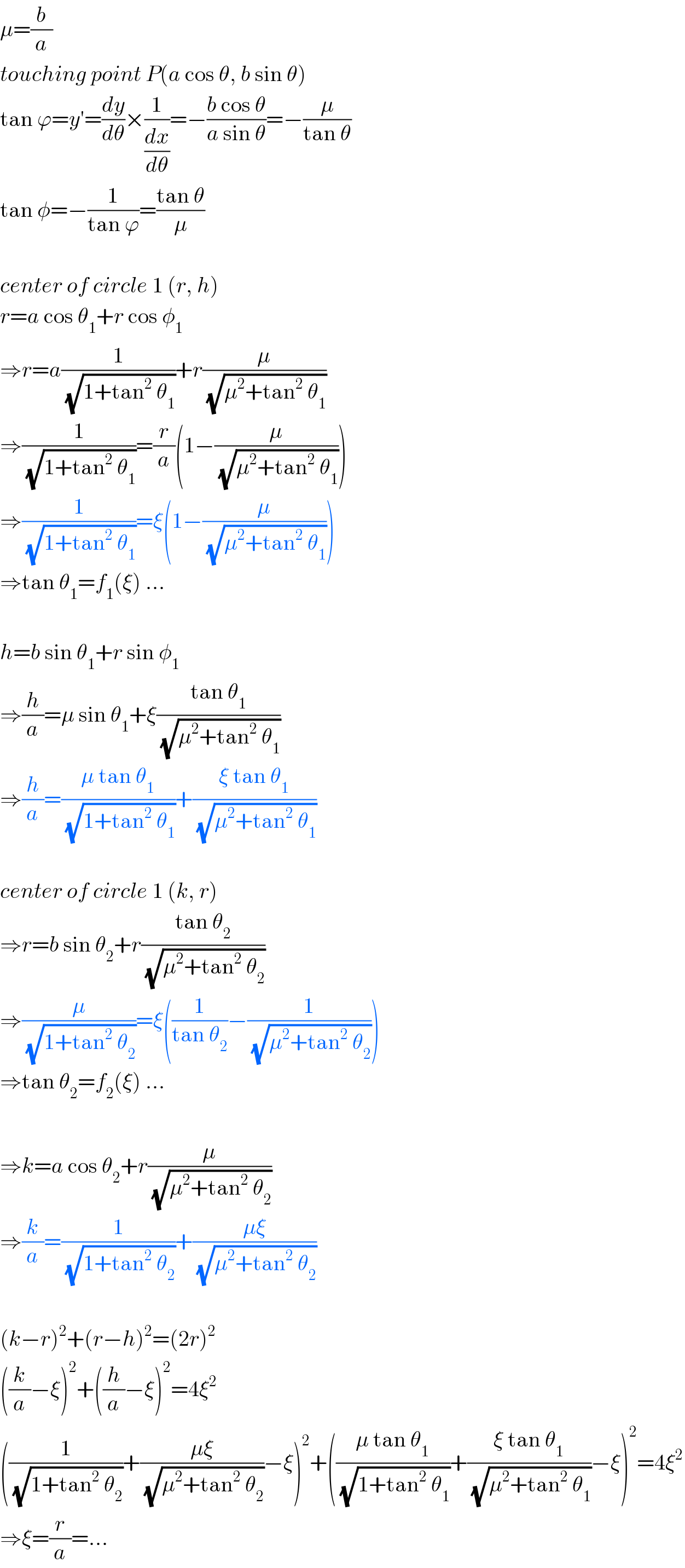
μ=(b/a) touching point P(a cos θ, b sin θ) tan ϕ=y′=(dy/dθ)×(1/(dx/dθ))=−((b cos θ)/(a sin θ))=−(μ/(tan θ)) tan φ=−(1/(tan ϕ))=((tan θ)/μ) center of circle 1 (r, h) r=a cos θ_1 +r cos φ_1 ⇒r=a(1/( (√(1+tan^2 θ_1 ))))+r(μ/( (√(μ^2 +tan^2 θ_1 )))) ⇒(1/( (√(1+tan^2 θ_1 ))))=(r/a)(1−(μ/( (√(μ^2 +tan^2 θ_1 ))))) ⇒(1/( (√(1+tan^2 θ_1 ))))=ξ(1−(μ/( (√(μ^2 +tan^2 θ_1 ))))) ⇒tan θ_1 =f_1 (ξ) ... h=b sin θ_1 +r sin φ_1 ⇒(h/a)=μ sin θ_1 +ξ((tan θ_1 )/( (√(μ^2 +tan^2 θ_1 )))) ⇒(h/a)=((μ tan θ_1 )/( (√(1+tan^2 θ_1 ))))+((ξ tan θ_1 )/( (√(μ^2 +tan^2 θ_1 )))) center of circle 1 (k, r) ⇒r=b sin θ_2 +r((tan θ_2 )/( (√(μ^2 +tan^2 θ_2 )))) ⇒(μ/( (√(1+tan^2 θ_2 ))))=ξ((1/(tan θ_2 ))−(1/( (√(μ^2 +tan^2 θ_2 ))))) ⇒tan θ_2 =f_2 (ξ) ... ⇒k=a cos θ_2 +r(μ/( (√(μ^2 +tan^2 θ_2 )))) ⇒(k/a)=(1/( (√(1+tan^2 θ_2 ))))+((μξ)/( (√(μ^2 +tan^2 θ_2 )))) (k−r)^2 +(r−h)^2 =(2r)^2 ((k/a)−ξ)^2 +((h/a)−ξ)^2 =4ξ^2 ((1/( (√(1+tan^2 θ_2 ))))+((μξ)/( (√(μ^2 +tan^2 θ_2 ))))−ξ)^2 +(((μ tan θ_1 )/( (√(1+tan^2 θ_1 ))))+((ξ tan θ_1 )/( (√(μ^2 +tan^2 θ_1 ))))−ξ)^2 =4ξ^2 ⇒ξ=(r/a)=...
$$\mu=\frac{{b}}{{a}} \\ $$$${touching}\:{point}\:{P}\left({a}\:\mathrm{cos}\:\theta,\:{b}\:\mathrm{sin}\:\theta\right) \\ $$$$\mathrm{tan}\:\varphi={y}'=\frac{{dy}}{{d}\theta}×\frac{\mathrm{1}}{\frac{{dx}}{{d}\theta}}=−\frac{{b}\:\mathrm{cos}\:\theta}{{a}\:\mathrm{sin}\:\theta}=−\frac{\mu}{\mathrm{tan}\:\theta} \\ $$$$\mathrm{tan}\:\phi=−\frac{\mathrm{1}}{\mathrm{tan}\:\varphi}=\frac{\mathrm{tan}\:\theta}{\mu} \\ $$$$ \\ $$$${center}\:{of}\:{circle}\:\mathrm{1}\:\left({r},\:{h}\right) \\ $$$${r}={a}\:\mathrm{cos}\:\theta_{\mathrm{1}} +{r}\:\mathrm{cos}\:\phi_{\mathrm{1}} \\ $$$$\Rightarrow{r}={a}\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}+{r}\frac{\mu}{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}=\frac{{r}}{{a}}\left(\mathrm{1}−\frac{\mu}{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}\right) \\ $$$$\Rightarrow\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}=\xi\left(\mathrm{1}−\frac{\mu}{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}\right) \\ $$$$\Rightarrow\mathrm{tan}\:\theta_{\mathrm{1}} ={f}_{\mathrm{1}} \left(\xi\right)\:… \\ $$$$ \\ $$$${h}={b}\:\mathrm{sin}\:\theta_{\mathrm{1}} +{r}\:\mathrm{sin}\:\phi_{\mathrm{1}} \\ $$$$\Rightarrow\frac{{h}}{{a}}=\mu\:\mathrm{sin}\:\theta_{\mathrm{1}} +\xi\frac{\mathrm{tan}\:\theta_{\mathrm{1}} }{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }} \\ $$$$\Rightarrow\frac{{h}}{{a}}=\frac{\mu\:\mathrm{tan}\:\theta_{\mathrm{1}} }{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}+\frac{\xi\:\mathrm{tan}\:\theta_{\mathrm{1}} }{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }} \\ $$$$ \\ $$$${center}\:{of}\:{circle}\:\mathrm{1}\:\left({k},\:{r}\right) \\ $$$$\Rightarrow{r}={b}\:\mathrm{sin}\:\theta_{\mathrm{2}} +{r}\frac{\mathrm{tan}\:\theta_{\mathrm{2}} }{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }} \\ $$$$\Rightarrow\frac{\mu}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }}=\xi\left(\frac{\mathrm{1}}{\mathrm{tan}\:\theta_{\mathrm{2}} }−\frac{\mathrm{1}}{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }}\right) \\ $$$$\Rightarrow\mathrm{tan}\:\theta_{\mathrm{2}} ={f}_{\mathrm{2}} \left(\xi\right)\:… \\ $$$$ \\ $$$$\Rightarrow{k}={a}\:\mathrm{cos}\:\theta_{\mathrm{2}} +{r}\frac{\mu}{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }} \\ $$$$\Rightarrow\frac{{k}}{{a}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }}+\frac{\mu\xi}{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }} \\ $$$$ \\ $$$$\left({k}−{r}\right)^{\mathrm{2}} +\left({r}−{h}\right)^{\mathrm{2}} =\left(\mathrm{2}{r}\right)^{\mathrm{2}} \\ $$$$\left(\frac{{k}}{{a}}−\xi\right)^{\mathrm{2}} +\left(\frac{{h}}{{a}}−\xi\right)^{\mathrm{2}} =\mathrm{4}\xi^{\mathrm{2}} \\ $$$$\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }}+\frac{\mu\xi}{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{2}} }}−\xi\right)^{\mathrm{2}} +\left(\frac{\mu\:\mathrm{tan}\:\theta_{\mathrm{1}} }{\:\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}+\frac{\xi\:\mathrm{tan}\:\theta_{\mathrm{1}} }{\:\sqrt{\mu^{\mathrm{2}} +\mathrm{tan}^{\mathrm{2}} \:\theta_{\mathrm{1}} }}−\xi\right)^{\mathrm{2}} =\mathrm{4}\xi^{\mathrm{2}} \\ $$$$\Rightarrow\xi=\frac{{r}}{{a}}=… \\ $$
Commented by ajfour last updated on 10/Jul/20
Thanks for the path Sir.. but its really a challenge yet..!
$${Thanks}\:{for}\:{the}\:{path}\:{Sir}.. \\ $$$${but}\:{its}\:{really}\:{a}\:{challenge}\:{yet}..! \\ $$
Answered by ajfour last updated on 08/Jul/20
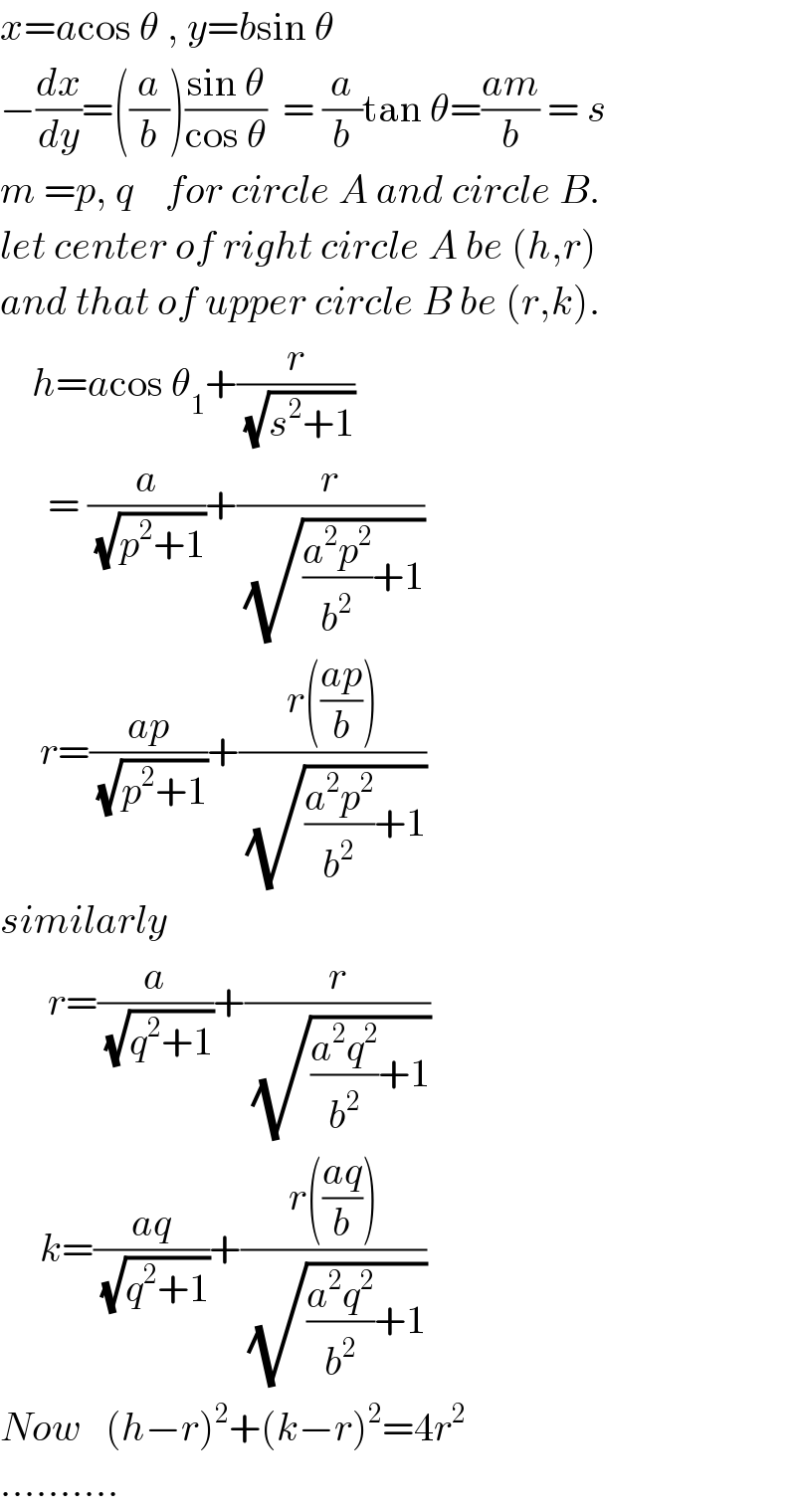
x=acos θ , y=bsin θ −(dx/dy)=((a/b))((sin θ)/(cos θ)) = (a/b)tan θ=((am)/b) = s m =p, q for circle A and circle B. let center of right circle A be (h,r) and that of upper circle B be (r,k). h=acos θ_1 +(r/( (√(s^2 +1)))) = (a/( (√(p^2 +1))))+(r/( ((((a^2 p^2 )/b^2 )+1))^(1/) )) r=((ap)/( (√(p^2 +1))))+((r(((ap)/b)))/( ((((a^2 p^2 )/b^2 )+1))^(1/) )) similarly r=(a/( (√(q^2 +1))))+(r/( ((((a^2 q^2 )/b^2 )+1))^(1/) )) k=((aq)/( (√(q^2 +1))))+((r(((aq)/b)))/( ((((a^2 q^2 )/b^2 )+1))^(1/) )) Now (h−r)^2 +(k−r)^2 =4r^2 ..........
$${x}={a}\mathrm{cos}\:\theta\:,\:{y}={b}\mathrm{sin}\:\theta \\ $$$$−\frac{{dx}}{{dy}}=\left(\frac{{a}}{{b}}\right)\frac{\mathrm{sin}\:\theta}{\mathrm{cos}\:\theta}\:\:=\:\frac{{a}}{{b}}\mathrm{tan}\:\theta=\frac{{am}}{{b}}\:=\:{s} \\ $$$${m}\:={p},\:{q}\:\:\:\:{for}\:{circle}\:{A}\:{and}\:{circle}\:{B}. \\ $$$${let}\:{center}\:{of}\:{right}\:{circle}\:{A}\:{be}\:\left({h},{r}\right) \\ $$$${and}\:{that}\:{of}\:{upper}\:{circle}\:{B}\:{be}\:\left({r},{k}\right). \\ $$$$\:\:\:\:{h}={a}\mathrm{cos}\:\theta_{\mathrm{1}} +\frac{{r}}{\:\sqrt{{s}^{\mathrm{2}} +\mathrm{1}}} \\ $$$$\:\:\:\:\:\:=\:\frac{{a}}{\:\sqrt{{p}^{\mathrm{2}} +\mathrm{1}}}+\frac{{r}}{\:\sqrt[{}]{\frac{{a}^{\mathrm{2}} {p}^{\mathrm{2}} }{{b}^{\mathrm{2}} }+\mathrm{1}}} \\ $$$$\:\:\:\:\:{r}=\frac{{ap}}{\:\sqrt{{p}^{\mathrm{2}} +\mathrm{1}}}+\frac{{r}\left(\frac{{ap}}{{b}}\right)}{\:\sqrt[{}]{\frac{{a}^{\mathrm{2}} {p}^{\mathrm{2}} }{{b}^{\mathrm{2}} }+\mathrm{1}}} \\ $$$${similarly} \\ $$$$\:\:\:\:\:\:{r}=\frac{{a}}{\:\sqrt{{q}^{\mathrm{2}} +\mathrm{1}}}+\frac{{r}}{\:\sqrt[{}]{\frac{{a}^{\mathrm{2}} {q}^{\mathrm{2}} }{{b}^{\mathrm{2}} }+\mathrm{1}}} \\ $$$$\:\:\:\:\:{k}=\frac{{aq}}{\:\sqrt{{q}^{\mathrm{2}} +\mathrm{1}}}+\frac{{r}\left(\frac{{aq}}{{b}}\right)}{\:\sqrt[{}]{\frac{{a}^{\mathrm{2}} {q}^{\mathrm{2}} }{{b}^{\mathrm{2}} }+\mathrm{1}}} \\ $$$${Now}\:\:\:\left({h}−{r}\right)^{\mathrm{2}} +\left({k}−{r}\right)^{\mathrm{2}} =\mathrm{4}{r}^{\mathrm{2}} \\ $$$$………. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *