Question Number 102698 by bramlex last updated on 10/Jul/20
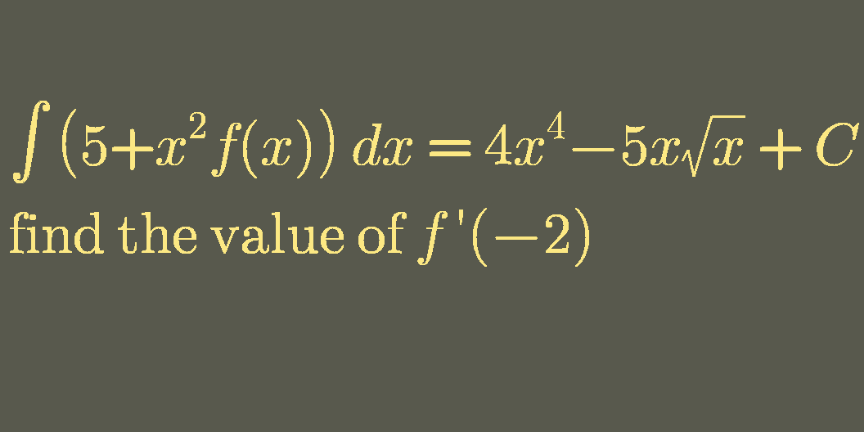
Answered by bramlex last updated on 10/Jul/20
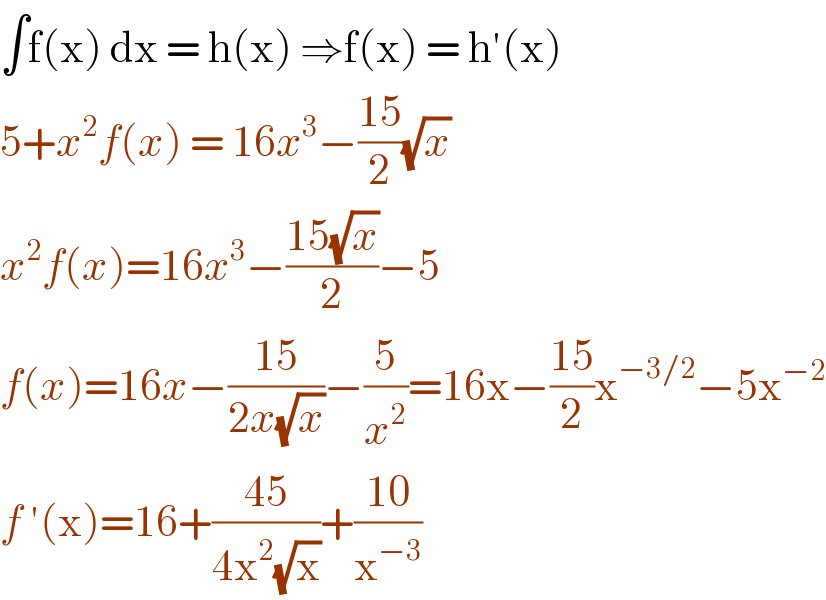
$$\int\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{dx}\:=\:\mathrm{h}\left(\mathrm{x}\right)\:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)\:=\:\mathrm{h}'\left(\mathrm{x}\right) \\ $$$$\mathrm{5}+{x}^{\mathrm{2}} {f}\left({x}\right)\:=\:\mathrm{16}{x}^{\mathrm{3}} −\frac{\mathrm{15}}{\mathrm{2}}\sqrt{{x}}\: \\ $$$${x}^{\mathrm{2}} {f}\left({x}\right)=\mathrm{16}{x}^{\mathrm{3}} −\frac{\mathrm{15}\sqrt{{x}}}{\mathrm{2}}−\mathrm{5} \\ $$$${f}\left({x}\right)=\mathrm{16}{x}−\frac{\mathrm{15}}{\mathrm{2}{x}\sqrt{{x}}}−\frac{\mathrm{5}}{{x}^{\mathrm{2}} }=\mathrm{16x}−\frac{\mathrm{15}}{\mathrm{2}}\mathrm{x}^{−\mathrm{3}/\mathrm{2}} −\mathrm{5x}^{−\mathrm{2}} \\ $$$${f}\:'\left(\mathrm{x}\right)=\mathrm{16}+\frac{\mathrm{45}}{\mathrm{4x}^{\mathrm{2}} \sqrt{\mathrm{x}}}+\frac{\mathrm{10}}{\mathrm{x}^{−\mathrm{3}} } \\ $$
Answered by floor(10²Eta[1]) last updated on 10/Jul/20

$$\mathrm{5}+\mathrm{x}^{\mathrm{2}} \mathrm{f}\left(\mathrm{x}\right)=\mathrm{16x}^{\mathrm{3}} −\frac{\mathrm{15}\sqrt{\mathrm{x}}}{\mathrm{2}} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{16x}−\frac{\mathrm{15}}{\mathrm{2}}\mathrm{x}^{−\mathrm{3}/\mathrm{2}} −\mathrm{5x}^{−\mathrm{2}} \\ $$$$\mathrm{f}'\left(\mathrm{x}\right)=\mathrm{16}+\frac{\mathrm{45}}{\mathrm{4}}\mathrm{x}^{−\mathrm{5}/\mathrm{2}} +\mathrm{10x}^{−\mathrm{3}} \\ $$$$\mathrm{f}'\left(−\mathrm{2}\right)=\mathrm{16}+\frac{\mathrm{45}}{\mathrm{4}}×\frac{\mathrm{1}}{\:\sqrt{\left(−\mathrm{2}\right)^{\mathrm{5}} }}+\mathrm{10}×\frac{\mathrm{1}}{\left(−\mathrm{2}\right)^{\mathrm{3}} } \\ $$$$=\frac{\mathrm{59}}{\mathrm{4}}+\frac{\mathrm{45}}{\mathrm{16i}\sqrt{\mathrm{2}}} \\ $$
Commented by bramlex last updated on 10/Jul/20

$$\smile\bullet\smile\bullet\smile \\ $$
