Menu Close

Question-102838

Tinku Tara 0 Comments
Pin




Question Number 102838 by ajfour last updated on 11/Jul/20
Commented by ajfour last updated on 11/Jul/20
If regions 1 and 2 have equal areas, find OB=y in terms of OA=a .
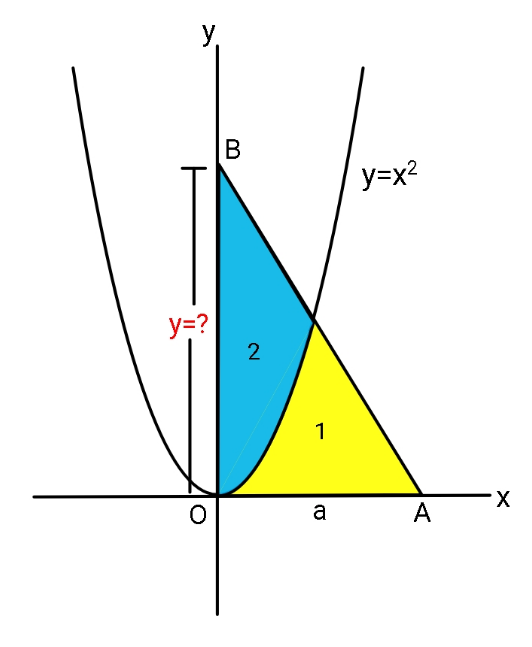
$${If}\:{regions}\:\mathrm{1}\:{and}\:\mathrm{2}\:{have}\:{equal} \\ $$$${areas},\:{find}\:{OB}={y}\:\:{in}\:{terms}\:{of} \\ $$$${OA}={a}\:. \\ $$
Commented by ajfour last updated on 11/Jul/20
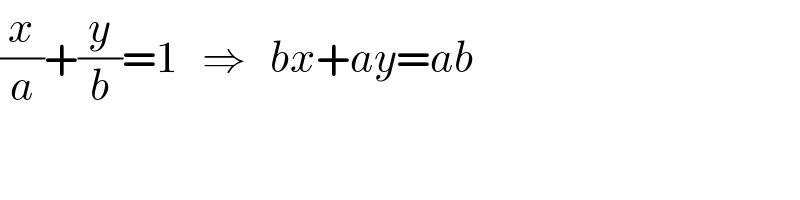
(x/a)+(y/b)=1 ⇒ bx+ay=ab
$$\frac{{x}}{{a}}+\frac{{y}}{{b}}=\mathrm{1}\:\:\:\Rightarrow\:\:\:{bx}+{ay}={ab} \\ $$
Answered by mr W last updated on 11/Jul/20
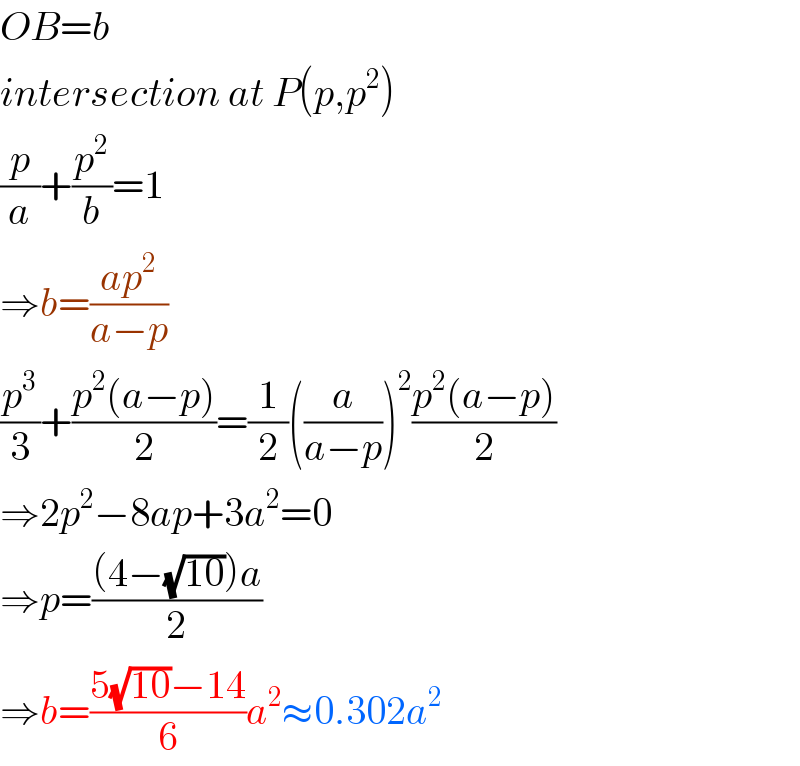
OB=b intersection at P(p,p^2 ) (p/a)+(p^2 /b)=1 ⇒b=((ap^2 )/(a−p)) (p^3 /3)+((p^2 (a−p))/2)=(1/2)((a/(a−p)))^2 ((p^2 (a−p))/2) ⇒2p^2 −8ap+3a^2 =0 ⇒p=(((4−(√(10)))a)/2) ⇒b=((5(√(10))−14)/6)a^2 ≈0.302a^2
$${OB}={b} \\ $$$${intersection}\:{at}\:{P}\left({p},{p}^{\mathrm{2}} \right) \\ $$$$\frac{{p}}{{a}}+\frac{{p}^{\mathrm{2}} }{{b}}=\mathrm{1} \\ $$$$\Rightarrow{b}=\frac{{ap}^{\mathrm{2}} }{{a}−{p}} \\ $$$$\frac{{p}^{\mathrm{3}} }{\mathrm{3}}+\frac{{p}^{\mathrm{2}} \left({a}−{p}\right)}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{{a}}{{a}−{p}}\right)^{\mathrm{2}} \frac{{p}^{\mathrm{2}} \left({a}−{p}\right)}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{2}{p}^{\mathrm{2}} −\mathrm{8}{ap}+\mathrm{3}{a}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow{p}=\frac{\left(\mathrm{4}−\sqrt{\mathrm{10}}\right){a}}{\mathrm{2}} \\ $$$$\Rightarrow{b}=\frac{\mathrm{5}\sqrt{\mathrm{10}}−\mathrm{14}}{\mathrm{6}}{a}^{\mathrm{2}} \approx\mathrm{0}.\mathrm{302}{a}^{\mathrm{2}} \\ $$
Commented by mr W last updated on 11/Jul/20
Commented by ajfour last updated on 11/Jul/20
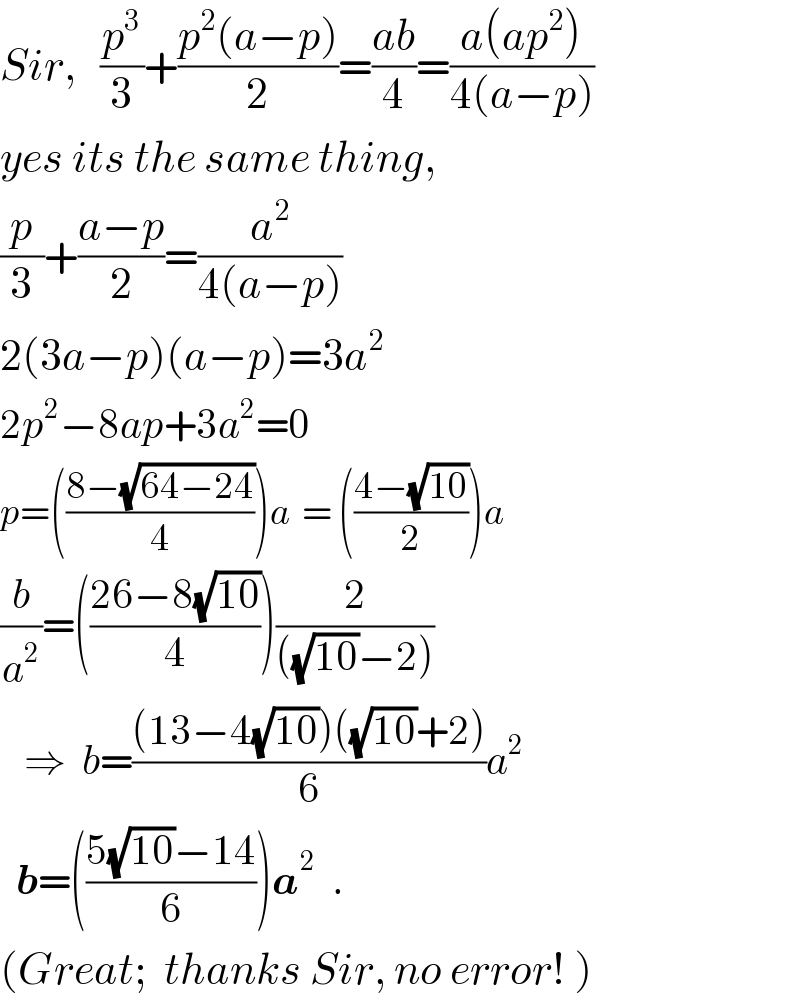
Sir, (p^3 /3)+((p^2 (a−p))/2)=((ab)/4)=((a(ap^2 ))/(4(a−p))) yes its the same thing, (p/3)+((a−p)/2)=(a^2 /(4(a−p))) 2(3a−p)(a−p)=3a^2 2p^2 −8ap+3a^2 =0 p=(((8−(√(64−24)))/4))a = (((4−(√(10)))/2))a (b/a^2 )=(((26−8(√(10)))/4))(2/(((√(10))−2))) ⇒ b=(((13−4(√(10)))((√(10))+2))/6)a^2 b=(((5(√(10))−14)/6))a^2 . (Great; thanks Sir, no error! )
$${Sir},\:\:\:\frac{{p}^{\mathrm{3}} }{\mathrm{3}}+\frac{{p}^{\mathrm{2}} \left({a}−{p}\right)}{\mathrm{2}}=\frac{{ab}}{\mathrm{4}}=\frac{{a}\left({ap}^{\mathrm{2}} \right)}{\mathrm{4}\left({a}−{p}\right)} \\ $$$${yes}\:{its}\:{the}\:{same}\:{thing},\: \\ $$$$\frac{{p}}{\mathrm{3}}+\frac{{a}−{p}}{\mathrm{2}}=\frac{{a}^{\mathrm{2}} }{\mathrm{4}\left({a}−{p}\right)} \\ $$$$\mathrm{2}\left(\mathrm{3}{a}−{p}\right)\left({a}−{p}\right)=\mathrm{3}{a}^{\mathrm{2}} \\ $$$$\mathrm{2}{p}^{\mathrm{2}} −\mathrm{8}{ap}+\mathrm{3}{a}^{\mathrm{2}} =\mathrm{0} \\ $$$${p}=\left(\frac{\mathrm{8}−\sqrt{\mathrm{64}−\mathrm{24}}}{\mathrm{4}}\right){a}\:\:=\:\left(\frac{\mathrm{4}−\sqrt{\mathrm{10}}}{\mathrm{2}}\right){a} \\ $$$$\frac{{b}}{{a}^{\mathrm{2}} }=\left(\frac{\mathrm{26}−\mathrm{8}\sqrt{\mathrm{10}}}{\mathrm{4}}\right)\frac{\mathrm{2}}{\left(\sqrt{\mathrm{10}}−\mathrm{2}\right)} \\ $$$$\:\:\:\Rightarrow\:\:{b}=\frac{\left(\mathrm{13}−\mathrm{4}\sqrt{\mathrm{10}}\right)\left(\sqrt{\mathrm{10}}+\mathrm{2}\right)}{\mathrm{6}}{a}^{\mathrm{2}} \\ $$$$\:\:\boldsymbol{{b}}=\left(\frac{\mathrm{5}\sqrt{\mathrm{10}}−\mathrm{14}}{\mathrm{6}}\right)\boldsymbol{{a}}^{\mathrm{2}} \:\:. \\ $$$$\left({Great};\:\:{thanks}\:{Sir},\:{no}\:{error}!\:\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *