Question-103021

Question Number 103021 by dw last updated on 12/Jul/20

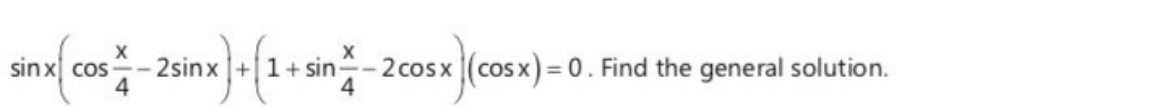
Answered by Dwaipayan Shikari last updated on 12/Jul/20

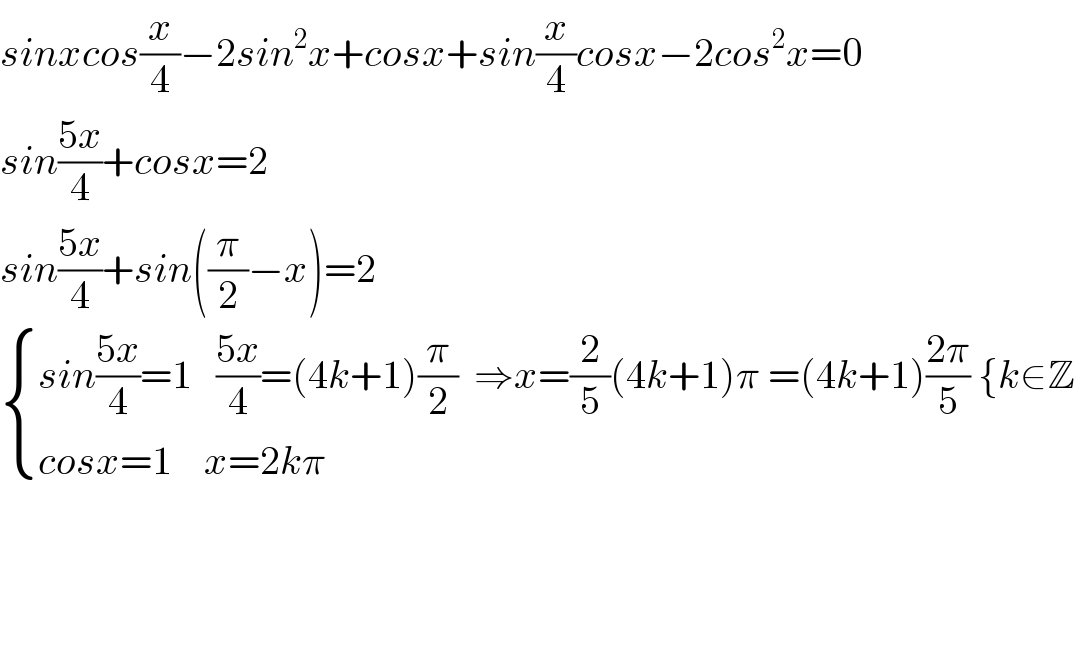
sinxcos(x/4)−2sin^2 x+cosx+sin(x/4)cosx−2cos^2 x=0 sin((5x)/4)+cosx=2 sin((5x)/4)+sin((π/2)−x)=2 { ((sin((5x)/4)=1 ((5x)/4)=(4k+1)(π/2) ⇒x=(2/5)(4k+1)π =(4k+1)((2π)/5) {k∈Z)),((cosx=1 x=2kπ)) :}

$${sinxcos}\frac{{x}}{\mathrm{4}}−\mathrm{2}{sin}^{\mathrm{2}} {x}+{cosx}+{sin}\frac{{x}}{\mathrm{4}}{cosx}−\mathrm{2}{cos}^{\mathrm{2}} {x}=\mathrm{0} \\ $$$${sin}\frac{\mathrm{5}{x}}{\mathrm{4}}+{cosx}=\mathrm{2} \\ $$$${sin}\frac{\mathrm{5}{x}}{\mathrm{4}}+{sin}\left(\frac{\pi}{\mathrm{2}}−{x}\right)=\mathrm{2} \\ $$$$\begin{cases}{{sin}\frac{\mathrm{5}{x}}{\mathrm{4}}=\mathrm{1}\:\:\:\frac{\mathrm{5}{x}}{\mathrm{4}}=\left(\mathrm{4}{k}+\mathrm{1}\right)\frac{\pi}{\mathrm{2}}\:\:\Rightarrow{x}=\frac{\mathrm{2}}{\mathrm{5}}\left(\mathrm{4}{k}+\mathrm{1}\right)\pi\:=\left(\mathrm{4}{k}+\mathrm{1}\right)\frac{\mathrm{2}\pi}{\mathrm{5}}\:\left\{{k}\in\mathbb{Z}\right.}\\{{cosx}=\mathrm{1}\:\:\:\:{x}=\mathrm{2}{k}\pi}\end{cases} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$ \\ $$

Answered by 1549442205 last updated on 13/Jul/20

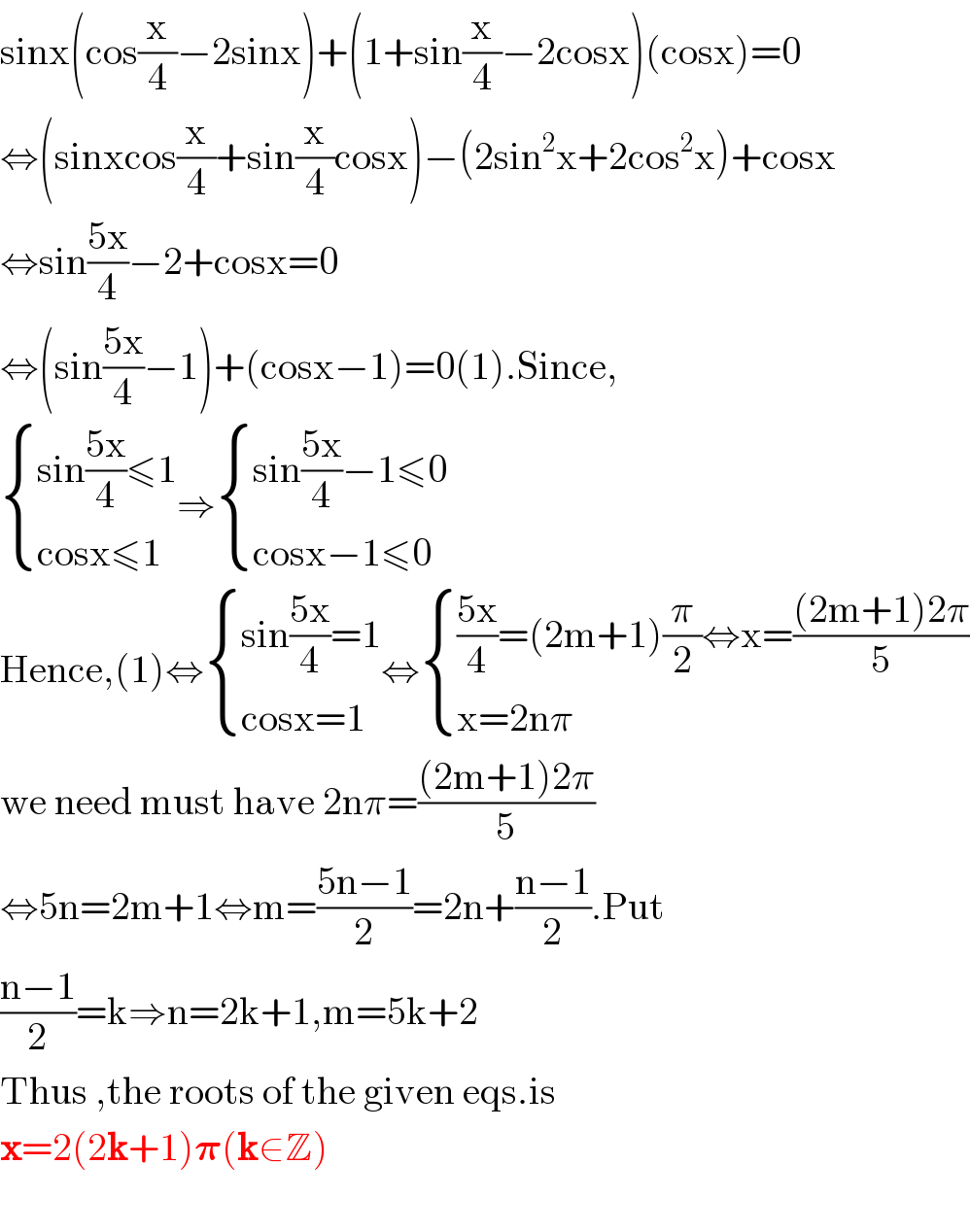
sinx(cos(x/4)−2sinx)+(1+sin(x/4)−2cosx)(cosx)=0 ⇔(sinxcos(x/4)+sin(x/4)cosx)−(2sin^2 x+2cos^2 x)+cosx ⇔sin((5x)/4)−2+cosx=0 ⇔(sin((5x)/4)−1)+(cosx−1)=0(1).Since, { ((sin((5x)/4)≤1)),((cosx≤1)) :}⇒ { ((sin((5x)/4)−1≤0)),((cosx−1≤0)) :} Hence,(1)⇔ { ((sin((5x)/4)=1)),((cosx=1)) :}⇔ { ((((5x)/4)=(2m+1)(π/2)⇔x=(((2m+1)2π)/5))),((x=2nπ)) :} we need must have 2nπ=(((2m+1)2π)/5) ⇔5n=2m+1⇔m=((5n−1)/2)=2n+((n−1)/2).Put ((n−1)/2)=k⇒n=2k+1,m=5k+2 Thus ,the roots of the given eqs.is x=2(2k+1)𝛑(k∈Z)

$$\mathrm{sinx}\left(\mathrm{cos}\frac{\mathrm{x}}{\mathrm{4}}−\mathrm{2sinx}\right)+\left(\mathrm{1}+\mathrm{sin}\frac{\mathrm{x}}{\mathrm{4}}−\mathrm{2cosx}\right)\left(\mathrm{cosx}\right)=\mathrm{0} \\ $$$$\Leftrightarrow\left(\mathrm{sinxcos}\frac{\mathrm{x}}{\mathrm{4}}+\mathrm{sin}\frac{\mathrm{x}}{\mathrm{4}}\mathrm{cosx}\right)−\left(\mathrm{2sin}^{\mathrm{2}} \mathrm{x}+\mathrm{2cos}^{\mathrm{2}} \mathrm{x}\right)+\mathrm{cosx} \\ $$$$\Leftrightarrow\mathrm{sin}\frac{\mathrm{5x}}{\mathrm{4}}−\mathrm{2}+\mathrm{cosx}=\mathrm{0} \\ $$$$\Leftrightarrow\left(\mathrm{sin}\frac{\mathrm{5x}}{\mathrm{4}}−\mathrm{1}\right)+\left(\mathrm{cosx}−\mathrm{1}\right)=\mathrm{0}\left(\mathrm{1}\right).\mathrm{Since}, \\ $$$$\begin{cases}{\mathrm{sin}\frac{\mathrm{5x}}{\mathrm{4}}\leqslant\mathrm{1}}\\{\mathrm{cosx}\leqslant\mathrm{1}}\end{cases}\Rightarrow\begin{cases}{\mathrm{sin}\frac{\mathrm{5x}}{\mathrm{4}}−\mathrm{1}\leqslant\mathrm{0}}\\{\mathrm{cosx}−\mathrm{1}\leqslant\mathrm{0}}\end{cases} \\ $$$$\mathrm{Hence},\left(\mathrm{1}\right)\Leftrightarrow\begin{cases}{\mathrm{sin}\frac{\mathrm{5x}}{\mathrm{4}}=\mathrm{1}}\\{\mathrm{cosx}=\mathrm{1}}\end{cases}\Leftrightarrow\begin{cases}{\frac{\mathrm{5x}}{\mathrm{4}}=\left(\mathrm{2m}+\mathrm{1}\right)\frac{\pi}{\mathrm{2}}\Leftrightarrow\mathrm{x}=\frac{\left(\mathrm{2m}+\mathrm{1}\right)\mathrm{2}\pi}{\mathrm{5}}}\\{\mathrm{x}=\mathrm{2n}\pi}\end{cases} \\ $$$$\mathrm{we}\:\mathrm{need}\:\mathrm{must}\:\mathrm{have}\:\mathrm{2n}\pi=\frac{\left(\mathrm{2m}+\mathrm{1}\right)\mathrm{2}\pi}{\mathrm{5}} \\ $$$$\Leftrightarrow\mathrm{5n}=\mathrm{2m}+\mathrm{1}\Leftrightarrow\mathrm{m}=\frac{\mathrm{5n}−\mathrm{1}}{\mathrm{2}}=\mathrm{2n}+\frac{\mathrm{n}−\mathrm{1}}{\mathrm{2}}.\mathrm{Put} \\ $$$$\frac{\mathrm{n}−\mathrm{1}}{\mathrm{2}}=\mathrm{k}\Rightarrow\mathrm{n}=\mathrm{2k}+\mathrm{1},\mathrm{m}=\mathrm{5k}+\mathrm{2} \\ $$$$\mathrm{Thus}\:,\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{given}\:\mathrm{eqs}.\mathrm{is} \\ $$$$\boldsymbol{\mathrm{x}}=\mathrm{2}\left(\mathrm{2}\boldsymbol{\mathrm{k}}+\mathrm{1}\right)\boldsymbol{\pi}\left(\boldsymbol{\mathrm{k}}\in\mathbb{Z}\right) \\ $$$$ \\ $$

Commented by dw last updated on 16/Jul/20

$${Thank}\:{you}\:{Sir}. \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply