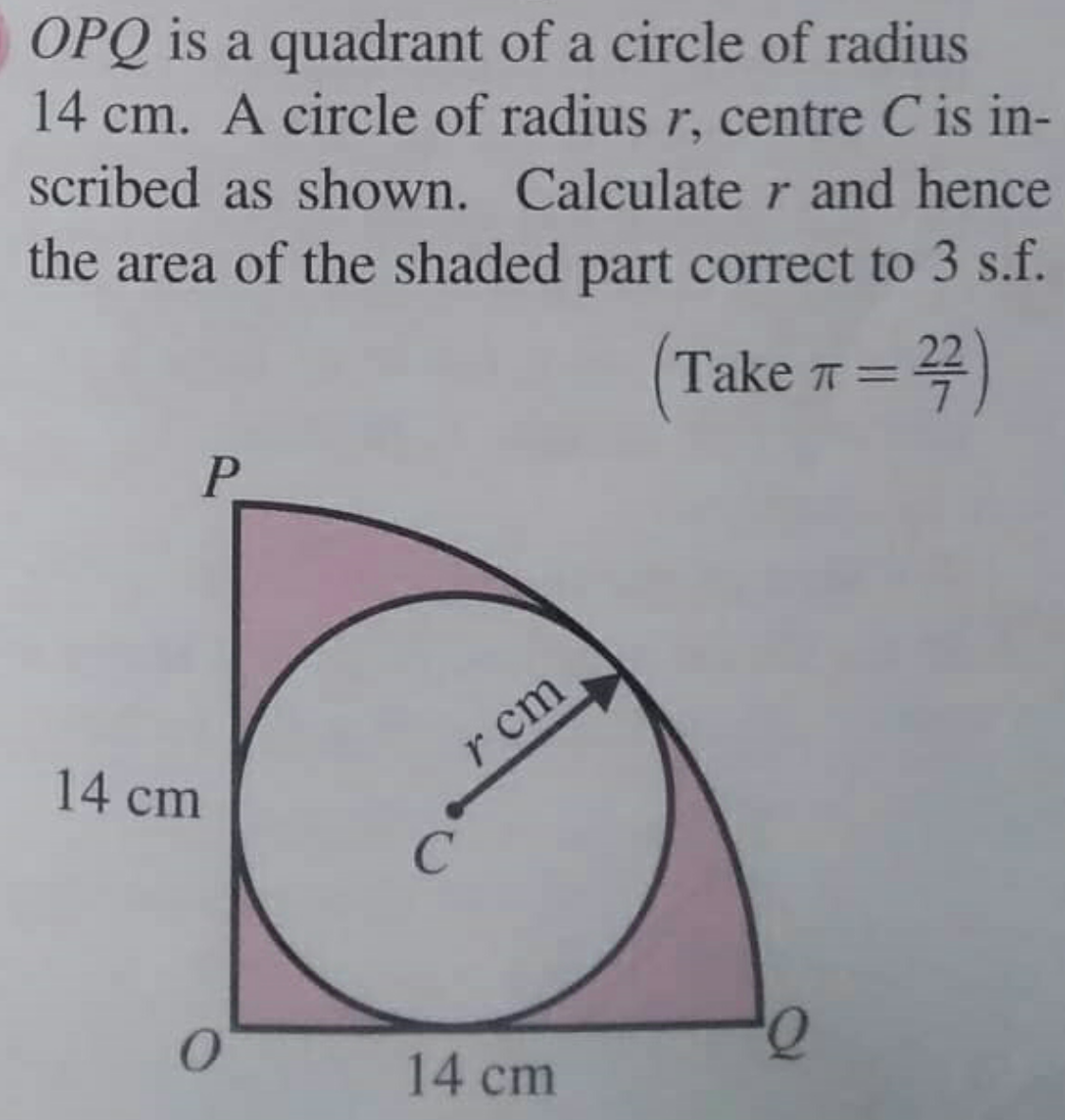
Question Number 103338 by I want to learn more last updated on 14/Jul/20
Commented by som(math1967) last updated on 14/Jul/20
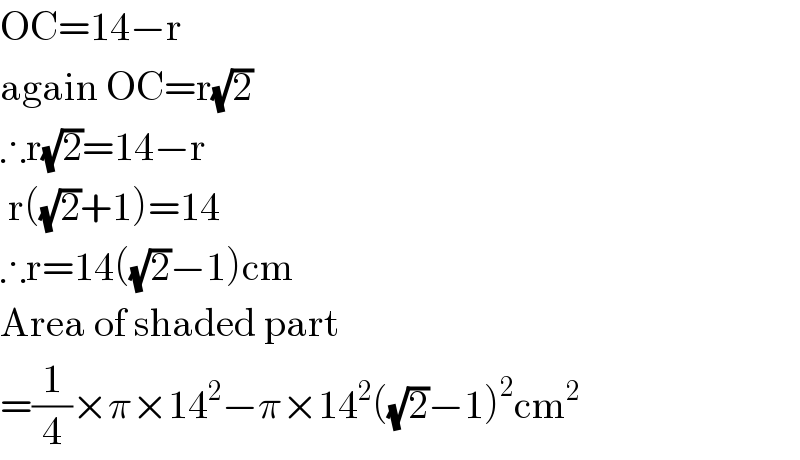
$$\mathrm{OC}=\mathrm{14}−\mathrm{r} \\ $$$$\mathrm{again}\:\mathrm{OC}=\mathrm{r}\sqrt{\mathrm{2}} \\ $$$$\therefore\mathrm{r}\sqrt{\mathrm{2}}=\mathrm{14}−\mathrm{r} \\ $$$$\:\mathrm{r}\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)=\mathrm{14} \\ $$$$\therefore\mathrm{r}=\mathrm{14}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\mathrm{cm} \\ $$$$\mathrm{Area}\:\mathrm{of}\:\mathrm{shaded}\:\mathrm{part} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}×\pi×\mathrm{14}^{\mathrm{2}} −\pi×\mathrm{14}^{\mathrm{2}} \left(\sqrt{\mathrm{2}}−\mathrm{1}\right)^{\mathrm{2}} \mathrm{cm}^{\mathrm{2}} \\ $$
Commented by I want to learn more last updated on 14/Jul/20

$$\mathrm{Wow},\:\mathrm{thanks}\:\mathrm{sir},\:\mathrm{i}\:\mathrm{appreciate}. \\ $$
Commented by harckinwunmy last updated on 14/Jul/20

$$\mathrm{are}\:\mathrm{you}\:\mathrm{from}\:\mathrm{Nigeria}? \\ $$
Commented by Study last updated on 14/Jul/20

$${why}\:{OC}={r}\sqrt{\mathrm{2}} \\ $$
Commented by som(math1967) last updated on 14/Jul/20
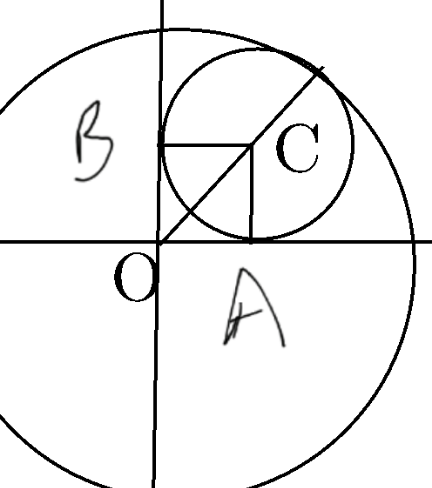
Commented by som(math1967) last updated on 14/Jul/20
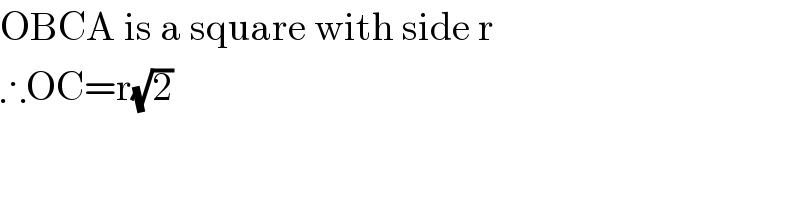
$$\mathrm{OBCA}\:\mathrm{is}\:\mathrm{a}\:\mathrm{square}\:\mathrm{with}\:\mathrm{side}\:\mathrm{r} \\ $$$$\therefore\mathrm{OC}=\mathrm{r}\sqrt{\mathrm{2}} \\ $$
Commented by Tawa11 last updated on 15/Sep/21

$$\mathrm{nice} \\ $$
