Menu Close

Question-103716

Tinku Tara 0 Comments
Pin




Question Number 103716 by mr W last updated on 16/Jul/20
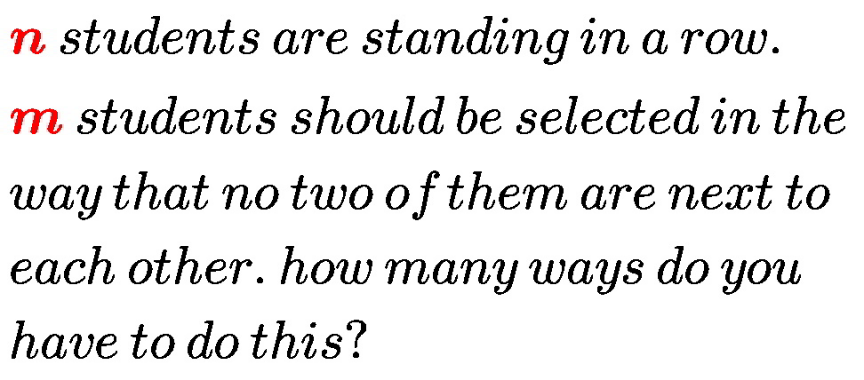
Commented by mr W last updated on 16/Jul/20
after thinking about it for two days, i think i have found the general solution which is simply C_m ^(n−m+1) . please give a try, prove or disprove it!
$${after}\:{thinking}\:{about}\:{it}\:{for}\:{two}\:{days}, \\ $$$${i}\:{think}\:{i}\:{have}\:{found}\:{the}\:{general} \\ $$$${solution}\:{which}\:{is}\:{simply} \\ $$$$\boldsymbol{{C}}_{\boldsymbol{{m}}} ^{\boldsymbol{{n}}−\boldsymbol{{m}}+\mathrm{1}} . \\ $$$${please}\:{give}\:{a}\:{try},\:{prove}\:{or}\:{disprove}\:{it}! \\ $$
Commented by prakash jain last updated on 16/Jul/20
s_1 s_2 s_3 s_4 ...s_n if we draw m bars and select student to the left of bar we have total n postion for bars. s_1 ∙ s_2 ∙ s_3 ∙s_4 ∙...∙s_n So for example 6,2 case s∣ s s s s∣ s We need m bars, the first bar can have 1 or more students to the left. The remaining bars can only have 2 or more student to the left of bar. 0 or more student can be present after last bar. Σ_(i=1) ^n x(Σ_(i=2) ^n x^2 )^(m−1) Σ_(i=0) ^n x^i ((x(1−x^n ))/(1−x))×((x^(2(m−1)) (1−x^(n−1) )^(m−1) )/((1−x)^(m−1) ))×(((1−x^(n+1) ))/(1−x)) m≥1 =x^(2m−1) ×(1/((1−x)^(m+1) )) =x^(2m−1) Σ_(i=0) ^∞ C_i ^(m+i) x^i sum of student must be n 2m−1+i=n⇒i=n+1−2m Required result C_(n+1−2m) ^(m+n+1−2m) =C_(n+1−2m) ^(n−m+1) =C_((n−m+1)−(n+1−2m)) ^(n−m+1) =C_m ^(n−m+1) Please feedback.
$$\mathrm{s}_{\mathrm{1}} \:\mathrm{s}_{\mathrm{2}} \:\:\mathrm{s}_{\mathrm{3}} \:\:\mathrm{s}_{\mathrm{4}} \:…\mathrm{s}_{\mathrm{n}} \\ $$$$\mathrm{if}\:\mathrm{we}\:\mathrm{draw}\:{m}\:\mathrm{bars}\:\mathrm{and}\:\mathrm{select} \\ $$$$\mathrm{student}\:\mathrm{to}\:\mathrm{the}\:\mathrm{left}\:\mathrm{of}\:\mathrm{bar}\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{total}\:\mathrm{n}\:\mathrm{postion}\:\mathrm{for}\:\mathrm{bars}. \\ $$$$\mathrm{s}_{\mathrm{1}} \centerdot\:\mathrm{s}_{\mathrm{2}} \centerdot\:\:\mathrm{s}_{\mathrm{3}} \:\:\centerdot\mathrm{s}_{\mathrm{4}} \:\centerdot…\centerdot\mathrm{s}_{\mathrm{n}} \\ $$$$\mathrm{So}\:\mathrm{for}\:\mathrm{example}\:\mathrm{6},\mathrm{2}\:\mathrm{case} \\ $$$$\mathrm{s}\mid\:\mathrm{s}\:\mathrm{s}\:\mathrm{s}\:\mathrm{s}\mid\:\mathrm{s} \\ $$$$\mathrm{We}\:\mathrm{need}\:\mathrm{m}\:\mathrm{bars}, \\ $$$$\mathrm{the}\:\mathrm{first}\:\mathrm{bar}\:\mathrm{can}\:\mathrm{have}\:\mathrm{1}\:\mathrm{or}\:\mathrm{more} \\ $$$$\mathrm{students}\:\mathrm{to}\:\mathrm{the}\:\mathrm{left}.\:\mathrm{The}\:\mathrm{remaining} \\ $$$$\mathrm{bars}\:\mathrm{can}\:\mathrm{only}\:\mathrm{have}\:\mathrm{2}\:\mathrm{or}\:\mathrm{more} \\ $$$$\mathrm{student}\:\mathrm{to}\:\mathrm{the}\:\mathrm{left}\:\mathrm{of}\:\mathrm{bar}. \\ $$$$\mathrm{0}\:\mathrm{or}\:\mathrm{more}\:\mathrm{student}\:\mathrm{can}\:\mathrm{be}\:\mathrm{present} \\ $$$$\mathrm{after}\:\mathrm{last}\:\mathrm{bar}. \\ $$$$\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{x}\left(\underset{{i}=\mathrm{2}} {\overset{{n}} {\sum}}{x}^{\mathrm{2}} \right)^{{m}−\mathrm{1}} \underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}{x}^{{i}} \\ $$$$\frac{{x}\left(\mathrm{1}−{x}^{{n}} \right)}{\mathrm{1}−{x}}×\frac{{x}^{\mathrm{2}\left({m}−\mathrm{1}\right)} \left(\mathrm{1}−{x}^{{n}−\mathrm{1}} \right)^{{m}−\mathrm{1}} }{\left(\mathrm{1}−{x}\right)^{{m}−\mathrm{1}} }×\frac{\left(\mathrm{1}−{x}^{{n}+\mathrm{1}} \right)}{\mathrm{1}−{x}} \\ $$$${m}\geqslant\mathrm{1} \\ $$$$={x}^{\mathrm{2}{m}−\mathrm{1}} ×\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)^{{m}+\mathrm{1}} } \\ $$$$={x}^{\mathrm{2}{m}−\mathrm{1}} \underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}{C}_{{i}} ^{{m}+{i}} {x}^{{i}} \\ $$$$\mathrm{sum}\:\mathrm{of}\:\mathrm{student}\:\mathrm{must}\:\mathrm{be}\:{n} \\ $$$$\mathrm{2}{m}−\mathrm{1}+{i}={n}\Rightarrow{i}={n}+\mathrm{1}−\mathrm{2}{m} \\ $$$$\mathrm{Required}\:\mathrm{result} \\ $$$$\mathrm{C}_{{n}+\mathrm{1}−\mathrm{2}{m}} ^{{m}+{n}+\mathrm{1}−\mathrm{2}{m}} ={C}_{{n}+\mathrm{1}−\mathrm{2}{m}} ^{{n}−{m}+\mathrm{1}} \\ $$$$={C}_{\left({n}−{m}+\mathrm{1}\right)−\left({n}+\mathrm{1}−\mathrm{2}{m}\right)} ^{{n}−{m}+\mathrm{1}} ={C}_{{m}} ^{{n}−{m}+\mathrm{1}} \\ $$$$\mathrm{Please}\:\mathrm{feedback}. \\ $$
Commented by mr W last updated on 16/Jul/20
correct sir!
$${correct}\:{sir}! \\ $$
Answered by mr W last updated on 17/Jul/20
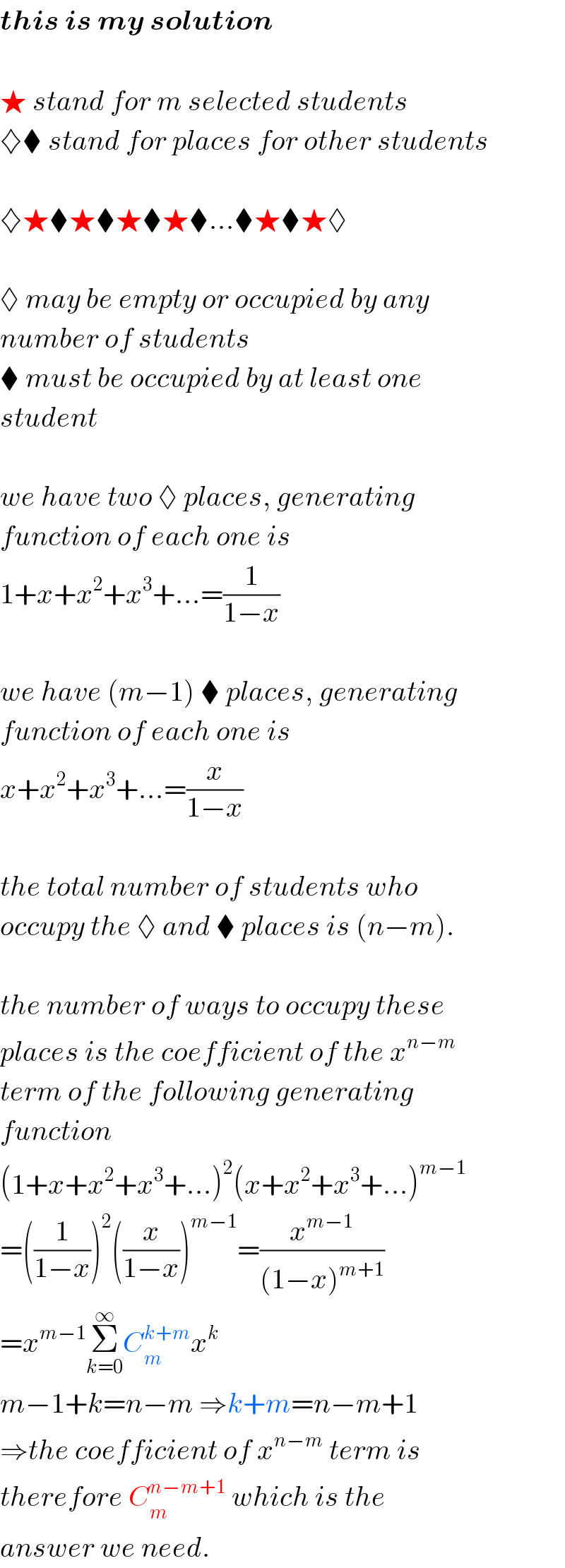
this is my solution ★ stand for m selected students ♦⧫ stand for places for other students ♦★⧫★⧫★⧫★⧫...⧫★⧫★◊ ◊ may be empty or occupied by any number of students ⧫ must be occupied by at least one student we have two ◊ places, generating function of each one is 1+x+x^2 +x^3 +...=(1/(1−x)) we have (m−1) ⧫ places, generating function of each one is x+x^2 +x^3 +...=(x/(1−x)) the total number of students who occupy the ◊ and ⧫ places is (n−m). the number of ways to occupy these places is the coefficient of the x^(n−m) term of the following generating function (1+x+x^2 +x^3 +...)^2 (x+x^2 +x^3 +...)^(m−1) =((1/(1−x)))^2 ((x/(1−x)))^(m−1) =(x^(m−1) /((1−x)^(m+1) )) =x^(m−1) Σ_(k=0) ^∞ C_m ^(k+m) x^k m−1+k=n−m ⇒k+m=n−m+1 ⇒the coefficient of x^(n−m) term is therefore C_m ^(n−m+1) which is the answer we need.
$$\boldsymbol{{this}}\:\boldsymbol{{is}}\:\boldsymbol{{my}}\:\boldsymbol{{solution}} \\ $$$$ \\ $$$$\bigstar\:{stand}\:{for}\:{m}\:{selected}\:{students} \\ $$$$\diamondsuit\blacklozenge\:{stand}\:{for}\:{places}\:{for}\:{other}\:{students} \\ $$$$ \\ $$$$\diamondsuit\bigstar\blacklozenge\bigstar\blacklozenge\bigstar\blacklozenge\bigstar\blacklozenge…\blacklozenge\bigstar\blacklozenge\bigstar\lozenge \\ $$$$ \\ $$$$\lozenge\:{may}\:{be}\:{empty}\:{or}\:{occupied}\:{by}\:{any} \\ $$$${number}\:{of}\:{students} \\ $$$$\blacklozenge\:{must}\:{be}\:{occupied}\:{by}\:{at}\:{least}\:{one} \\ $$$${student} \\ $$$$ \\ $$$${we}\:{have}\:{two}\:\lozenge\:{places},\:{generating} \\ $$$${function}\:{of}\:{each}\:{one}\:{is} \\ $$$$\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} +…=\frac{\mathrm{1}}{\mathrm{1}−{x}} \\ $$$$ \\ $$$${we}\:{have}\:\left({m}−\mathrm{1}\right)\:\blacklozenge\:{places},\:{generating} \\ $$$${function}\:{of}\:{each}\:{one}\:{is} \\ $$$${x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} +…=\frac{{x}}{\mathrm{1}−{x}} \\ $$$$ \\ $$$${the}\:{total}\:{number}\:{of}\:{students}\:{who} \\ $$$${occupy}\:{the}\:\lozenge\:{and}\:\blacklozenge\:{places}\:{is}\:\left({n}−{m}\right). \\ $$$$ \\ $$$${the}\:{number}\:{of}\:{ways}\:{to}\:{occupy}\:{these} \\ $$$${places}\:{is}\:{the}\:{coefficient}\:{of}\:{the}\:{x}^{{n}−{m}} \\ $$$${term}\:{of}\:{the}\:{following}\:{generating} \\ $$$${function} \\ $$$$\left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} +…\right)^{\mathrm{2}} \left({x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} +…\right)^{{m}−\mathrm{1}} \\ $$$$=\left(\frac{\mathrm{1}}{\mathrm{1}−{x}}\right)^{\mathrm{2}} \left(\frac{{x}}{\mathrm{1}−{x}}\right)^{{m}−\mathrm{1}} =\frac{{x}^{{m}−\mathrm{1}} }{\left(\mathrm{1}−{x}\right)^{{m}+\mathrm{1}} } \\ $$$$={x}^{{m}−\mathrm{1}} \underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}{C}_{{m}} ^{{k}+{m}} {x}^{{k}} \\ $$$${m}−\mathrm{1}+{k}={n}−{m}\:\Rightarrow{k}+{m}={n}−{m}+\mathrm{1} \\ $$$$\Rightarrow{the}\:{coefficient}\:{of}\:{x}^{{n}−{m}} \:{term}\:{is} \\ $$$${therefore}\:{C}_{{m}} ^{{n}−{m}+\mathrm{1}} \:{which}\:{is}\:{the} \\ $$$${answer}\:{we}\:{need}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *