Question Number 104157 by ajfour last updated on 19/Jul/20

Commented by ajfour last updated on 19/Jul/20
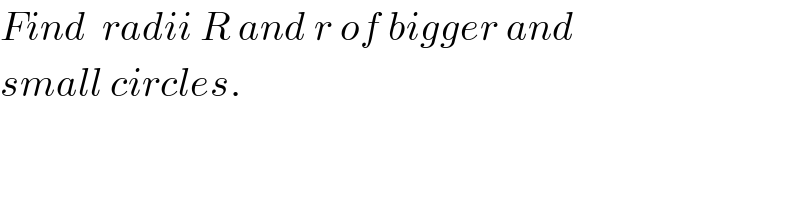
$${Find}\:\:{radii}\:{R}\:{and}\:{r}\:{of}\:{bigger}\:{and} \\ $$$${small}\:{circles}. \\ $$
Answered by mr W last updated on 19/Jul/20

$${H}\left(\mathrm{1},\mathrm{1}\right) \\ $$$${OH}=\sqrt{\mathrm{2}}{R}+{R}=\sqrt{\mathrm{2}} \\ $$$$\Rightarrow{R}=\frac{\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{2}}+\mathrm{1}}=\mathrm{2}−\sqrt{\mathrm{2}} \\ $$$${D}\left({R}+\mathrm{2}\sqrt{{Rr}},{r}\right)=\left({h},{r}\right) \\ $$$${F}\left({k},\frac{\mathrm{1}}{{k}}\right) \\ $$$$\mathrm{tan}\:\theta=\frac{\mathrm{1}}{{k}^{\mathrm{2}} } \\ $$$${k}={h}+{r}\:\mathrm{sin}\:\theta={R}+\mathrm{2}\sqrt{{Rr}}+{r}\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{k}^{\mathrm{4}} }} \\ $$$$\frac{\mathrm{1}}{{k}}={r}+{r}\:\mathrm{cos}\:\theta={r}\left(\mathrm{1}+\frac{{k}^{\mathrm{2}} }{\:\sqrt{\mathrm{1}+{k}^{\mathrm{4}} }}\right) \\ $$$$\Rightarrow{r}=\frac{\mathrm{1}}{{k}\left(\mathrm{1}+\frac{{k}^{\mathrm{2}} }{\:\sqrt{\mathrm{1}+{k}^{\mathrm{4}} }}\right)} \\ $$$${k}={R}+\mathrm{2}\sqrt{\frac{{R}}{{k}\left(\mathrm{1}+\frac{{k}^{\mathrm{2}} }{\:\sqrt{\mathrm{1}+{k}^{\mathrm{4}} }}\right)}}+\frac{\mathrm{1}}{{k}\left(\sqrt{\mathrm{1}+{k}^{\mathrm{4}} }+{k}^{\mathrm{2}} \right)} \\ $$$$\Rightarrow{k}\approx\mathrm{1}.\mathrm{5831} \\ $$$$\Rightarrow{r}\approx\mathrm{0}.\mathrm{3275} \\ $$
Commented by mr W last updated on 19/Jul/20

Commented by ajfour last updated on 19/Jul/20

$${I}\:{too}\:{think},\:{this}\:{as}\:{the}\:{possible}\:{way}, \\ $$$${Sir},\:{thanks}\:{for}\:{the}\:{solution}! \\ $$
Answered by ajfour last updated on 21/Jul/20

Commented by ajfour last updated on 22/Jul/20
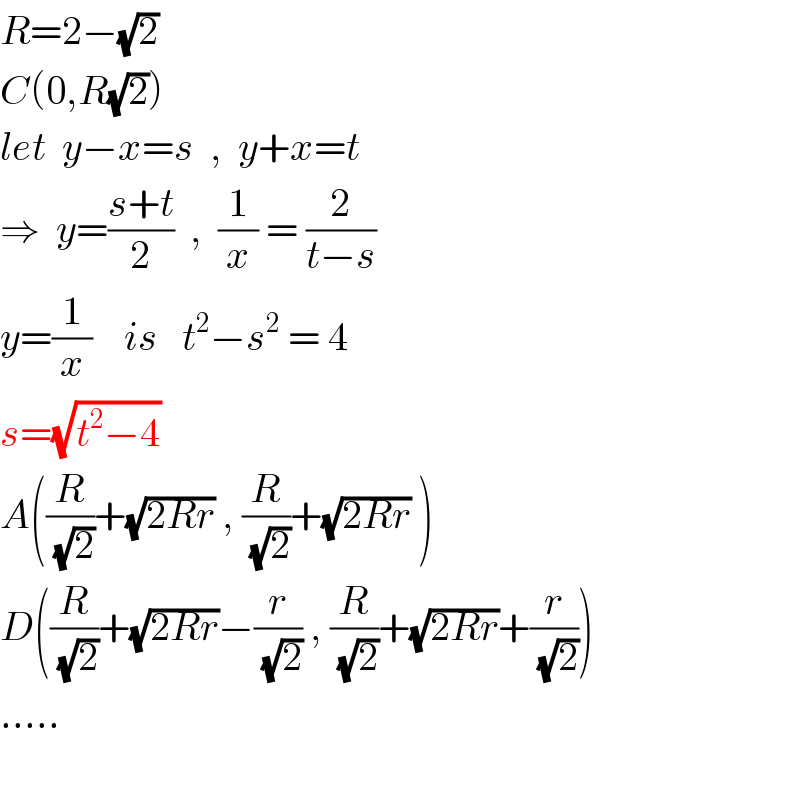
$${R}=\mathrm{2}−\sqrt{\mathrm{2}} \\ $$$${C}\left(\mathrm{0},{R}\sqrt{\mathrm{2}}\right)\: \\ $$$${let}\:\:{y}−{x}={s}\:\:,\:\:{y}+{x}={t} \\ $$$$\Rightarrow\:\:{y}=\frac{{s}+{t}}{\mathrm{2}}\:\:,\:\:\frac{\mathrm{1}}{{x}}\:=\:\frac{\mathrm{2}}{{t}−{s}} \\ $$$${y}=\frac{\mathrm{1}}{{x}}\:\:\:\:{is}\:\:\:{t}^{\mathrm{2}} −{s}^{\mathrm{2}} \:=\:\mathrm{4} \\ $$$${s}=\sqrt{{t}^{\mathrm{2}} −\mathrm{4}}\: \\ $$$${A}\left(\frac{{R}}{\:\sqrt{\mathrm{2}}}+\sqrt{\mathrm{2}{Rr}}\:,\:\frac{{R}}{\:\sqrt{\mathrm{2}}}+\sqrt{\mathrm{2}{Rr}}\:\right) \\ $$$${D}\left(\frac{{R}}{\:\sqrt{\mathrm{2}}}+\sqrt{\mathrm{2}{Rr}}−\frac{{r}}{\:\sqrt{\mathrm{2}}}\:,\:\frac{{R}}{\:\sqrt{\mathrm{2}}}+\sqrt{\mathrm{2}{Rr}}+\frac{{r}}{\:\sqrt{\mathrm{2}}}\right)\:\: \\ $$$$….. \\ $$$$ \\ $$
