Menu Close

Question-105594

Tinku Tara 0 Comments
Pin




Question Number 105594 by ajfour last updated on 30/Jul/20
Commented by ajfour last updated on 30/Jul/20
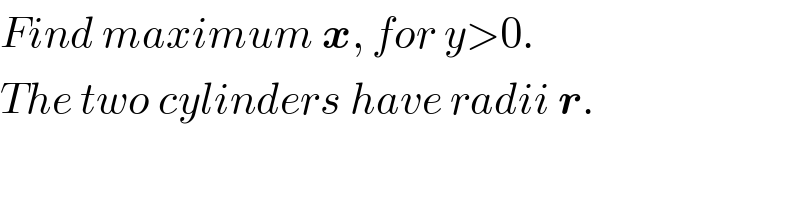
Find maximum x, for y>0. The two cylinders have radii r.
$${Find}\:{maximum}\:\boldsymbol{{x}},\:{for}\:{y}>\mathrm{0}. \\ $$$${The}\:{two}\:{cylinders}\:{have}\:{radii}\:\boldsymbol{{r}}. \\ $$
Commented by ajfour last updated on 30/Jul/20
Commented by ajfour last updated on 30/Jul/20
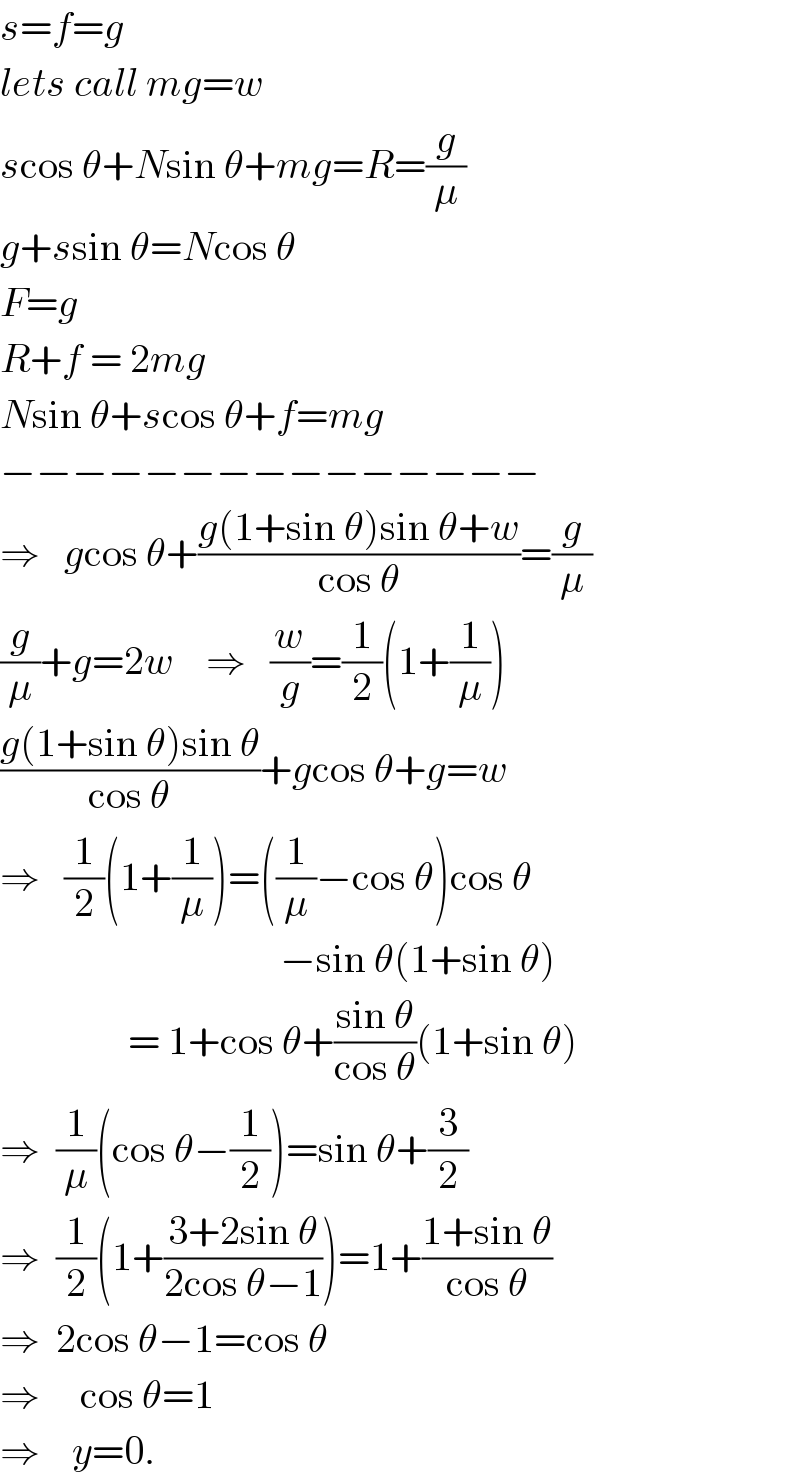
s=f=g lets call mg=w scos θ+Nsin θ+mg=R=(g/μ) g+ssin θ=Ncos θ F=g R+f = 2mg Nsin θ+scos θ+f=mg −−−−−−−−−−−−−−− ⇒ gcos θ+((g(1+sin θ)sin θ+w)/(cos θ))=(g/μ) (g/μ)+g=2w ⇒ (w/g)=(1/2)(1+(1/μ)) ((g(1+sin θ)sin θ)/(cos θ))+gcos θ+g=w ⇒ (1/2)(1+(1/μ))=((1/μ)−cos θ)cos θ −sin θ(1+sin θ) = 1+cos θ+((sin θ)/(cos θ))(1+sin θ) ⇒ (1/μ)(cos θ−(1/2))=sin θ+(3/2) ⇒ (1/2)(1+((3+2sin θ)/(2cos θ−1)))=1+((1+sin θ)/(cos θ)) ⇒ 2cos θ−1=cos θ ⇒ cos θ=1 ⇒ y=0.
$${s}={f}={g} \\ $$$${lets}\:{call}\:{mg}={w} \\ $$$${s}\mathrm{cos}\:\theta+{N}\mathrm{sin}\:\theta+{mg}={R}=\frac{{g}}{\mu} \\ $$$${g}+{s}\mathrm{sin}\:\theta={N}\mathrm{cos}\:\theta \\ $$$${F}={g} \\ $$$${R}+{f}\:=\:\mathrm{2}{mg} \\ $$$${N}\mathrm{sin}\:\theta+{s}\mathrm{cos}\:\theta+{f}={mg} \\ $$$$−−−−−−−−−−−−−−− \\ $$$$\Rightarrow\:\:\:{g}\mathrm{cos}\:\theta+\frac{{g}\left(\mathrm{1}+\mathrm{sin}\:\theta\right)\mathrm{sin}\:\theta+{w}}{\mathrm{cos}\:\theta}=\frac{{g}}{\mu} \\ $$$$\frac{{g}}{\mu}+{g}=\mathrm{2}{w}\:\:\:\:\Rightarrow\:\:\:\frac{{w}}{{g}}=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\frac{\mathrm{1}}{\mu}\right) \\ $$$$\frac{{g}\left(\mathrm{1}+\mathrm{sin}\:\theta\right)\mathrm{sin}\:\theta}{\mathrm{cos}\:\theta}+{g}\mathrm{cos}\:\theta+{g}={w} \\ $$$$\Rightarrow\:\:\:\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\frac{\mathrm{1}}{\mu}\right)=\left(\frac{\mathrm{1}}{\mu}−\mathrm{cos}\:\theta\right)\mathrm{cos}\:\theta \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\mathrm{sin}\:\theta\left(\mathrm{1}+\mathrm{sin}\:\theta\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{1}+\mathrm{cos}\:\theta+\frac{\mathrm{sin}\:\theta}{\mathrm{cos}\:\theta}\left(\mathrm{1}+\mathrm{sin}\:\theta\right) \\ $$$$\Rightarrow\:\:\frac{\mathrm{1}}{\mu}\left(\mathrm{cos}\:\theta−\frac{\mathrm{1}}{\mathrm{2}}\right)=\mathrm{sin}\:\theta+\frac{\mathrm{3}}{\mathrm{2}} \\ $$$$\Rightarrow\:\:\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+\frac{\mathrm{3}+\mathrm{2sin}\:\theta}{\mathrm{2cos}\:\theta−\mathrm{1}}\right)=\mathrm{1}+\frac{\mathrm{1}+\mathrm{sin}\:\theta}{\mathrm{cos}\:\theta} \\ $$$$\Rightarrow\:\:\mathrm{2cos}\:\theta−\mathrm{1}=\mathrm{cos}\:\theta \\ $$$$\Rightarrow\:\:\:\:\:\mathrm{cos}\:\theta=\mathrm{1} \\ $$$$\Rightarrow\:\:\:\:{y}=\mathrm{0}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *