Question Number 106029 by ajfour last updated on 02/Aug/20
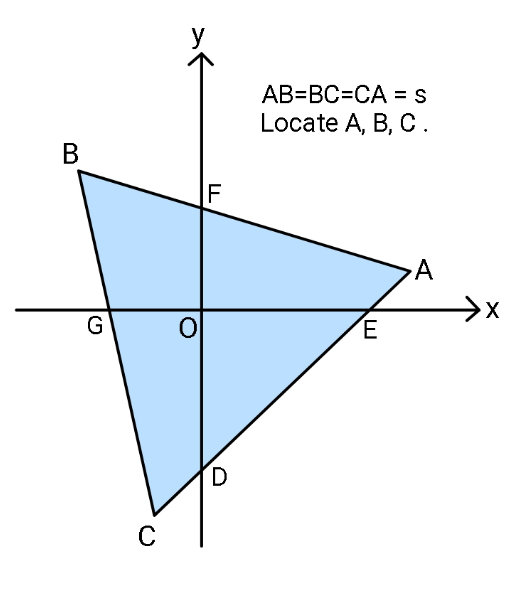
Commented by ajfour last updated on 02/Aug/20
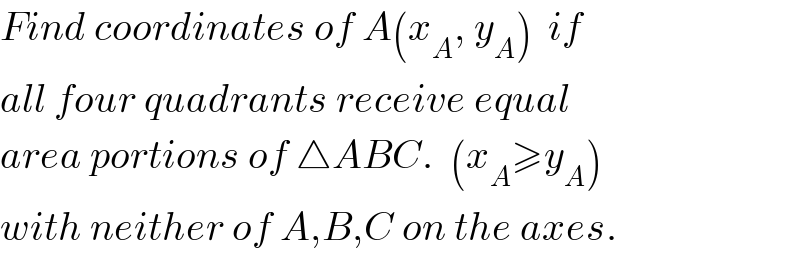
$${Find}\:{coordinates}\:{of}\:{A}\left({x}_{{A}} ,\:{y}_{{A}} \right)\:\:{if} \\ $$$${all}\:{four}\:{quadrants}\:{receive}\:{equal} \\ $$$${area}\:{portions}\:{of}\:\bigtriangleup{ABC}.\:\:\left({x}_{{A}} \geqslant{y}_{{A}} \right) \\ $$$${with}\:{neither}\:{of}\:{A},{B},{C}\:{on}\:{the}\:{axes}. \\ $$
Answered by ajfour last updated on 02/Aug/20
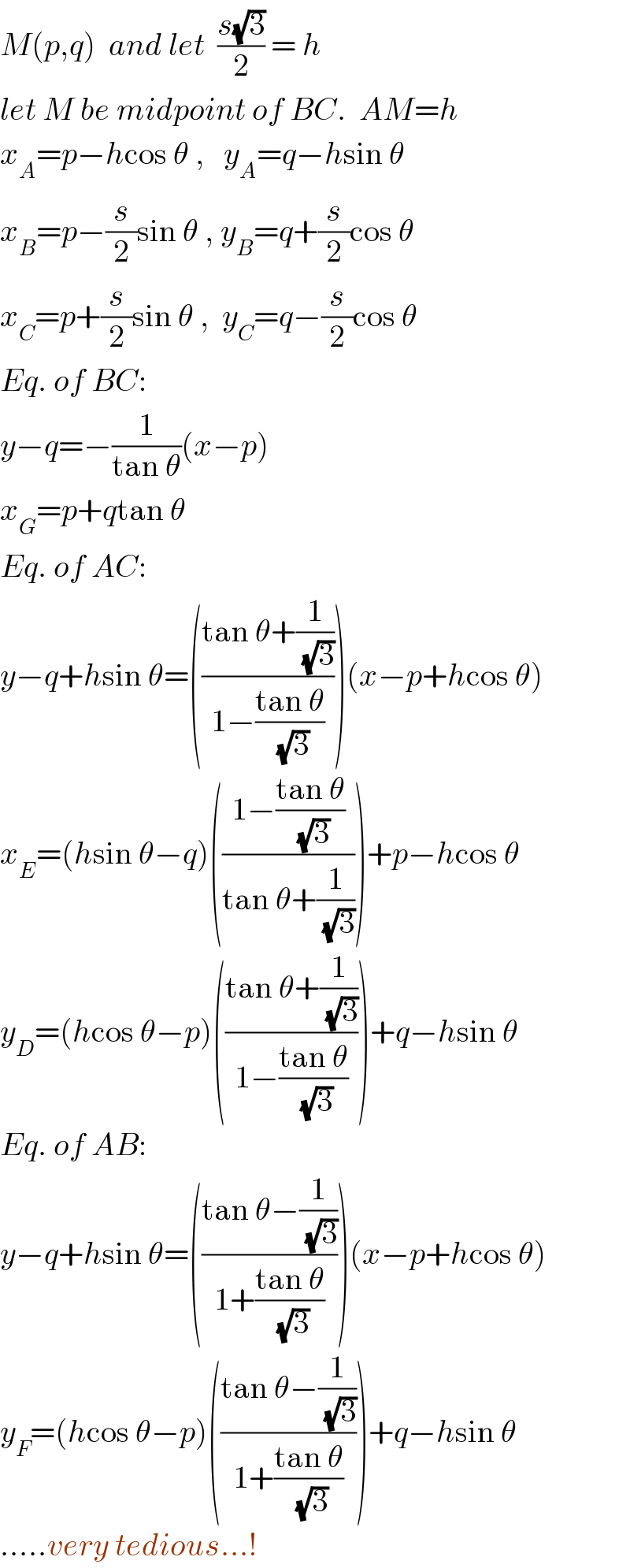
$${M}\left({p},{q}\right)\:\:{and}\:{let}\:\:\frac{{s}\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\:{h} \\ $$$${let}\:{M}\:{be}\:{midpoint}\:{of}\:{BC}.\:\:{AM}={h} \\ $$$${x}_{{A}} ={p}−{h}\mathrm{cos}\:\theta\:,\:\:\:{y}_{{A}} ={q}−{h}\mathrm{sin}\:\theta \\ $$$${x}_{{B}} ={p}−\frac{{s}}{\mathrm{2}}\mathrm{sin}\:\theta\:,\:{y}_{{B}} ={q}+\frac{{s}}{\mathrm{2}}\mathrm{cos}\:\theta \\ $$$${x}_{{C}} ={p}+\frac{{s}}{\mathrm{2}}\mathrm{sin}\:\theta\:,\:\:{y}_{{C}} ={q}−\frac{{s}}{\mathrm{2}}\mathrm{cos}\:\theta \\ $$$${Eq}.\:{of}\:{BC}: \\ $$$${y}−{q}=−\frac{\mathrm{1}}{\mathrm{tan}\:\theta}\left({x}−{p}\right) \\ $$$${x}_{{G}} ={p}+{q}\mathrm{tan}\:\theta \\ $$$${Eq}.\:{of}\:{AC}: \\ $$$${y}−{q}+{h}\mathrm{sin}\:\theta=\left(\frac{\mathrm{tan}\:\theta+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}}{\mathrm{1}−\frac{\mathrm{tan}\:\theta}{\:\sqrt{\mathrm{3}}}}\right)\left({x}−{p}+{h}\mathrm{cos}\:\theta\right) \\ $$$${x}_{{E}} =\left({h}\mathrm{sin}\:\theta−{q}\right)\left(\frac{\mathrm{1}−\frac{\mathrm{tan}\:\theta}{\:\sqrt{\mathrm{3}}}}{\mathrm{tan}\:\theta+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}}\right)+{p}−{h}\mathrm{cos}\:\theta \\ $$$${y}_{{D}} =\left({h}\mathrm{cos}\:\theta−{p}\right)\left(\frac{\mathrm{tan}\:\theta+\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}}{\mathrm{1}−\frac{\mathrm{tan}\:\theta}{\:\sqrt{\mathrm{3}}}}\right)+{q}−{h}\mathrm{sin}\:\theta \\ $$$${Eq}.\:{of}\:{AB}: \\ $$$${y}−{q}+{h}\mathrm{sin}\:\theta=\left(\frac{\mathrm{tan}\:\theta−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}}{\mathrm{1}+\frac{\mathrm{tan}\:\theta}{\:\sqrt{\mathrm{3}}}}\right)\left({x}−{p}+{h}\mathrm{cos}\:\theta\right) \\ $$$${y}_{{F}} =\left({h}\mathrm{cos}\:\theta−{p}\right)\left(\frac{\mathrm{tan}\:\theta−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}}{\mathrm{1}+\frac{\mathrm{tan}\:\theta}{\:\sqrt{\mathrm{3}}}}\right)+{q}−{h}\mathrm{sin}\:\theta \\ $$$$…..{very}\:{tedious}…! \\ $$
