Question-106604

Question Number 106604 by mohammad17 last updated on 06/Aug/20

Commented by bemath last updated on 06/Aug/20

Q4(5) lim_(x→1/2) ((6(√x)−6(√(2x−1)))/(x−1)) = (((6/( (√2)))−0)/(−(1/2))) = −((12)/( (√2))) = −6(√2) @bemath@

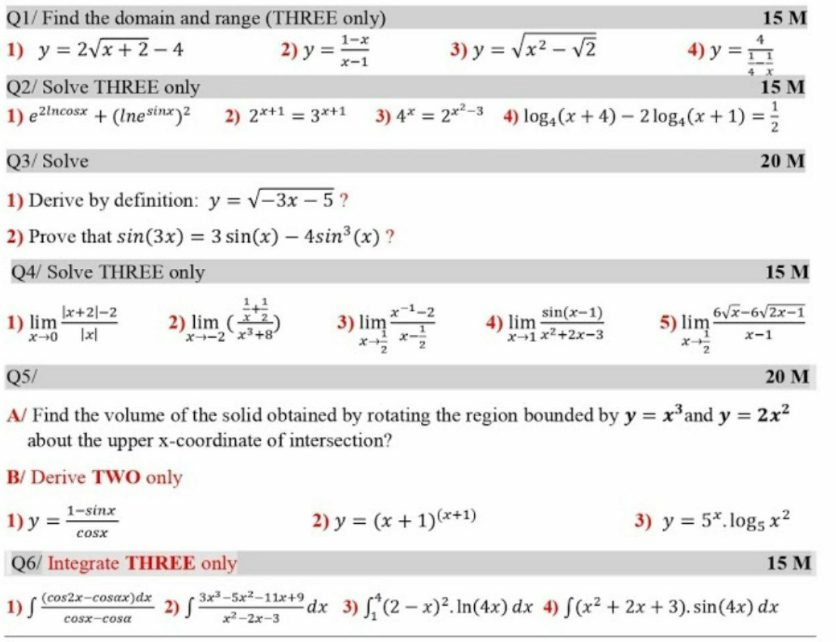
$$\mathrm{Q4}\left(\mathrm{5}\right)\:\underset{{x}\rightarrow\mathrm{1}/\mathrm{2}} {\mathrm{lim}}\:\frac{\mathrm{6}\sqrt{\mathrm{x}}−\mathrm{6}\sqrt{\mathrm{2x}−\mathrm{1}}}{\mathrm{x}−\mathrm{1}}\:=\:\frac{\frac{\mathrm{6}}{\:\sqrt{\mathrm{2}}}−\mathrm{0}}{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$=\:−\frac{\mathrm{12}}{\:\sqrt{\mathrm{2}}}\:=\:−\mathrm{6}\sqrt{\mathrm{2}}\:\:\:\:\:@\mathrm{bemath}@ \\ $$

Answered by Dwaipayan Shikari last updated on 06/Aug/20

y=2(√(x+2))−4 (x+2)≥0 Domain x∈[−2,∞) ((y+4)/2)=(√(x+2)) x=(((y+4)^2 )/4)−2 −2≤x<∞ −2≤(((y+4)^2 −8)/4)<∞ −8≤(y+4)^2 −8<∞ 0≤(y+4)^2 <∞ −4≤y<∞

$${y}=\mathrm{2}\sqrt{{x}+\mathrm{2}}−\mathrm{4} \\ $$$$\left({x}+\mathrm{2}\right)\geqslant\mathrm{0} \\ $$$${Domain}\:{x}\in\left[−\mathrm{2},\infty\right)\: \\ $$$$\frac{{y}+\mathrm{4}}{\mathrm{2}}=\sqrt{{x}+\mathrm{2}} \\ $$$${x}=\frac{\left({y}+\mathrm{4}\right)^{\mathrm{2}} }{\mathrm{4}}−\mathrm{2} \\ $$$$−\mathrm{2}\leqslant{x}<\infty \\ $$$$−\mathrm{2}\leqslant\frac{\left({y}+\mathrm{4}\right)^{\mathrm{2}} −\mathrm{8}}{\mathrm{4}}<\infty \\ $$$$−\mathrm{8}\leqslant\left({y}+\mathrm{4}\right)^{\mathrm{2}} −\mathrm{8}<\infty \\ $$$$\mathrm{0}\leqslant\left({y}+\mathrm{4}\right)^{\mathrm{2}} <\infty \\ $$$$−\mathrm{4}\leqslant{y}<\infty \\ $$$$ \\ $$

Answered by Dwaipayan Shikari last updated on 06/Aug/20

4)lim_(x→1) ((sin(x−1))/((x−1)(x+3)))=lim_(x→1) (((x−1))/((x−1)(x+3)))=(1/4)

$$\left.\mathrm{4}\right)\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{{sin}\left({x}−\mathrm{1}\right)}{\left({x}−\mathrm{1}\right)\left({x}+\mathrm{3}\right)}=\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\left({x}−\mathrm{1}\right)}{\left({x}−\mathrm{1}\right)\left({x}+\mathrm{3}\right)}=\frac{\mathrm{1}}{\mathrm{4}} \\ $$

Answered by Dwaipayan Shikari last updated on 06/Aug/20

4)∫(x^2 +2x+3)sin4xdx= ∫x^2 sin4x+2∫xsin4x+3∫sin4x =−(1/4)x^2 cos4x+(1/2)∫xcos4x−(1/2)xcos4x+(1/2)∫cos4x−(3/4)sin4x =−(1/4)x^2 cos4x+(1/8)xsin4x−(1/8)∫sin4x+(1/2)xcos4x+(1/8)sin4x−(3/4)sin4x =−(1/4)x^2 cos4x+sin4x((x/8)−(5/8))+(1/(32))cos4x+(1/2)cos4x+C

$$\left.\mathrm{4}\right)\int\left({x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{3}\right){sin}\mathrm{4}{xdx}= \\ $$$$\int{x}^{\mathrm{2}} {sin}\mathrm{4}{x}+\mathrm{2}\int{xsin}\mathrm{4}{x}+\mathrm{3}\int{sin}\mathrm{4}{x} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}{x}^{\mathrm{2}} {cos}\mathrm{4}{x}+\frac{\mathrm{1}}{\mathrm{2}}\int{xcos}\mathrm{4}{x}−\frac{\mathrm{1}}{\mathrm{2}}{xcos}\mathrm{4}{x}+\frac{\mathrm{1}}{\mathrm{2}}\int{cos}\mathrm{4}{x}−\frac{\mathrm{3}}{\mathrm{4}}{sin}\mathrm{4}{x} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}{x}^{\mathrm{2}} {cos}\mathrm{4}{x}+\frac{\mathrm{1}}{\mathrm{8}}{xsin}\mathrm{4}{x}−\frac{\mathrm{1}}{\mathrm{8}}\int{sin}\mathrm{4}{x}+\frac{\mathrm{1}}{\mathrm{2}}{xcos}\mathrm{4}{x}+\frac{\mathrm{1}}{\mathrm{8}}{sin}\mathrm{4}{x}−\frac{\mathrm{3}}{\mathrm{4}}{sin}\mathrm{4}{x} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}{x}^{\mathrm{2}} {cos}\mathrm{4}{x}+{sin}\mathrm{4}{x}\left(\frac{{x}}{\mathrm{8}}−\frac{\mathrm{5}}{\mathrm{8}}\right)+\frac{\mathrm{1}}{\mathrm{32}}{cos}\mathrm{4}{x}+\frac{\mathrm{1}}{\mathrm{2}}{cos}\mathrm{4}{x}+{C} \\ $$$$ \\ $$

Answered by bemath last updated on 06/Aug/20

Q4(1) lim_(x→0^+ ) ((∣x+2∣−2)/(∣x∣))=lim_(x→0^+ ) ((x+2−2)/x)=1 lim_(x→0^− ) ((∣x+2∣−2)/(∣x∣))= lim_(x→0^− ) ((x+2−2)/(−x))=−1 since lim_(x→0^+ ) f(x) ≠ lim_(x→0^− ) f(x) then lim_(x→0) ((∣x+2∣−2)/(∣x∣)) doesn′t exist @bemath@

$$\mathrm{Q4}\left(\mathrm{1}\right)\:\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\:\frac{\mid\mathrm{x}+\mathrm{2}\mid−\mathrm{2}}{\mid\mathrm{x}\mid}=\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\frac{\mathrm{x}+\mathrm{2}−\mathrm{2}}{\mathrm{x}}=\mathrm{1} \\ $$$$\underset{{x}\rightarrow\mathrm{0}^{−} } {\mathrm{lim}}\:\frac{\mid\mathrm{x}+\mathrm{2}\mid−\mathrm{2}}{\mid\mathrm{x}\mid}=\:\underset{{x}\rightarrow\mathrm{0}^{−} } {\mathrm{lim}}\:\frac{\mathrm{x}+\mathrm{2}−\mathrm{2}}{−\mathrm{x}}=−\mathrm{1} \\ $$$$\mathrm{since}\:\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}f}\left(\mathrm{x}\right)\:\neq\:\underset{{x}\rightarrow\mathrm{0}^{−} } {\mathrm{lim}f}\left(\mathrm{x}\right)\: \\ $$$$\mathrm{then}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mid\mathrm{x}+\mathrm{2}\mid−\mathrm{2}}{\mid\mathrm{x}\mid}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{exist} \\ $$$$@\mathrm{bemath}@ \\ $$

Commented by bemath last updated on 06/Aug/20

Answered by Dwaipayan Shikari last updated on 06/Aug/20

3)lim_(x→(1/2)) (((1/x)−2)/(x−(1/2)))=((1−2x)/(2x−1)).(2/x)=−1.4=−4

$$\left.\mathrm{3}\right)\underset{{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{\frac{\mathrm{1}}{{x}}−\mathrm{2}}{{x}−\frac{\mathrm{1}}{\mathrm{2}}}=\frac{\mathrm{1}−\mathrm{2}{x}}{\mathrm{2}{x}−\mathrm{1}}.\frac{\mathrm{2}}{{x}}=−\mathrm{1}.\mathrm{4}=−\mathrm{4} \\ $$

Answered by Dwaipayan Shikari last updated on 06/Aug/20

2)lim_(x→−2) (((x+2)/(2x))/(x^3 +8))=lim_(x→−2) ((1/(2x))/(x^2 −2x+4))=−(1/4).(1/(12))=−(1/(48))

$$\left.\mathrm{2}\right)\underset{{x}\rightarrow−\mathrm{2}} {\mathrm{lim}}\frac{\frac{{x}+\mathrm{2}}{\mathrm{2}{x}}}{{x}^{\mathrm{3}} +\mathrm{8}}=\underset{{x}\rightarrow−\mathrm{2}} {\mathrm{lim}}\frac{\frac{\mathrm{1}}{\mathrm{2}{x}}}{{x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{4}}=−\frac{\mathrm{1}}{\mathrm{4}}.\frac{\mathrm{1}}{\mathrm{12}}=−\frac{\mathrm{1}}{\mathrm{48}} \\ $$

Answered by 1549442205PVT last updated on 06/Aug/20

Q1a)y=2(√(x+2)) −4 .y is definited for ∀x∈[−2;+∞)⇒D(x)=[−2;+∞) We have y≥2.0−4=−4 (since (√(x+2))≥0) Thus,the domain of the values of y be D(y)=[−4;+∞) Q2a)Solve the equations: e^(2ln(cos x)) =(lne^(sinx) )^2 ⇔e^(ln(cosx)^2 ) +(sinx)^2 ⇔cos^2 x=sin^2 x(since e^(lnN) =N,lne^m =m) ⇔cos^2 x−sin^2 x=0⇔cos2x=0 ⇔2x=(𝛑/2)+k𝛑⇔x=(𝛑/4)+((k𝛑)/2) b)2^(x+1) =3^(x+1) ⇔((2/3))^(x+1) =1⇔x+1=0 ⇔x=−1 c)4^x =2^(x^2 −3) ⇔2^(2x) =2^(x^2 −3) ⇔x^2 −3=2x ⇔x^2 −2x−3=0 Δ;′=1+3=2^2 ⇒x=1±2⇒x∈{−1,3} d)log_4 (x+1)−2log_4 (x+1)=(1/2)(1) Condition for the eqn.is deined as x≥−1 (1)⇔log_4 (x+1)=−(1/2)⇔x+1=4^(−(1/2)) ⇔x+1=(1/( (√4)))=(1/2)⇔x=−(1/2) Q3.a)y=(√(−3x−5))⇒y′=((−3)/(2(√(−3x−5)))) b)sin3x=sin(x+2x)=sinxcos2x+cosxsin2x =sinx(1−2sin^2 x)+cosx.2sinxcosx =sinx−2sin^3 x+2sinx(1−sin^2 x) =3sinx−4sin^3 x (q.e.d) Q4.a)Lim_(x→0) ((∣x+2∣−2)/(∣x∣))=lim_(x→0) ((x+2−2)/(∣x∣)) =lim_(x→0) (x/(∣x∣))= { ((lim_(x→−0) (x/(−x))=−1)),((lim_(x→+0) (x/x)=1)) :} Since left−limit≠right−limit the given limit don′t exist b)lim_(x→−2) (((1/x)+(1/2))/(x^3 +8))=lim_(x→−2) (((x+2)/(2x))/((x+2)(x^2 −x+4)))= =lim_(x→−2) (1/(2x(x^2 −x+4)))=(1/(−4(4+2+4)))=((−1)/(40)) c)lim_(x→(1/2)) ((x^(−1) −2)/(x−(1/2)))=lim_(x→(1/2)) (((1/x)−2)/((2x−1)/2))=lim_(x→(1/2)) (((1−2x)/x)/((2x−1)/2)) =lim_(x→(1/2)) ((−2)/x)=−4 d)li m_(x→1) ((sin(x−1))/(x^2 +2x−3)) = _(x−1=t) lim_(x→1) ((sin(x−1))/((x−1)(x+3))) =lim_(t→0) ((sint)/(t(t+4))) =lim_(t→0) ((sint)/t)×(1/(t+4))=1×(1/4)=(1/4) e)lim_(x→(1/2)) ((6(√x)−6(√(2x−1)))/(x−1))=lim_(x→(1/2)) ((6[x−(2x−1))/((x−1)((√x)+(√(2x−1))))) =lim_(x→(1/2)) ((−6(x−1))/((x−1)((√x)+(√(2x−1)))))=lim_(x→(1/2)) ((−6)/( (√x)+(√(2x−1)))) =((−6)/( (√(1/2))))=−6(√2)

$$\left.\mathrm{Q1a}\right)\mathrm{y}=\mathrm{2}\sqrt{\mathrm{x}+\mathrm{2}}\:−\mathrm{4}\:.\mathrm{y}\:\mathrm{is}\:\mathrm{definited}\:\mathrm{for}\: \\ $$$$\forall\mathrm{x}\in\left[−\mathrm{2};+\infty\right)\Rightarrow\mathrm{D}\left(\mathrm{x}\right)=\left[−\mathrm{2};+\infty\right) \\ $$$$\mathrm{We}\:\mathrm{have}\:\mathrm{y}\geqslant\mathrm{2}.\mathrm{0}−\mathrm{4}=−\mathrm{4}\:\left(\mathrm{since}\:\sqrt{\mathrm{x}+\mathrm{2}}\geqslant\mathrm{0}\right) \\ $$$$\mathrm{Thus},\mathrm{the}\:\mathrm{domain}\:\mathrm{of}\:\mathrm{the}\:\mathrm{values}\:\mathrm{of}\:\mathrm{y}\:\mathrm{be}\: \\ $$$$\mathrm{D}\left(\mathrm{y}\right)=\left[−\mathrm{4};+\infty\right) \\ $$$$\left.\mathrm{Q2a}\right)\mathrm{Solve}\:\mathrm{the}\:\mathrm{equations}: \\ $$$$\mathrm{e}^{\mathrm{2ln}\left(\mathrm{cos}\:\mathrm{x}\right)} =\left(\mathrm{lne}^{\mathrm{sinx}} \right)^{\mathrm{2}} \Leftrightarrow\mathrm{e}^{\mathrm{ln}\left(\mathrm{cosx}\right)^{\mathrm{2}} } +\left(\mathrm{sinx}\right)^{\mathrm{2}} \\ $$$$\Leftrightarrow\mathrm{cos}^{\mathrm{2}} \mathrm{x}=\mathrm{sin}^{\mathrm{2}} \mathrm{x}\left(\mathrm{since}\:\mathrm{e}^{\mathrm{lnN}} =\mathrm{N},\mathrm{lne}^{\mathrm{m}} =\mathrm{m}\right) \\ $$$$\Leftrightarrow\mathrm{cos}^{\mathrm{2}} \mathrm{x}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}=\mathrm{0}\Leftrightarrow\mathrm{cos2x}=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{2}\boldsymbol{\mathrm{x}}=\frac{\boldsymbol{\pi}}{\mathrm{2}}+\boldsymbol{\mathrm{k}\pi}\Leftrightarrow\boldsymbol{\mathrm{x}}=\frac{\boldsymbol{\pi}}{\mathrm{4}}+\frac{\boldsymbol{\mathrm{k}\pi}}{\mathrm{2}}\: \\ $$$$\left.\mathrm{b}\right)\mathrm{2}^{\mathrm{x}+\mathrm{1}} =\mathrm{3}^{\mathrm{x}+\mathrm{1}} \Leftrightarrow\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{x}+\mathrm{1}} =\mathrm{1}\Leftrightarrow\mathrm{x}+\mathrm{1}=\mathrm{0} \\ $$$$\Leftrightarrow\boldsymbol{\mathrm{x}}=−\mathrm{1}\: \\ $$$$\left.\mathrm{c}\right)\mathrm{4}^{\mathrm{x}} =\mathrm{2}^{\mathrm{x}^{\mathrm{2}} −\mathrm{3}} \Leftrightarrow\mathrm{2}^{\mathrm{2x}} =\mathrm{2}^{\mathrm{x}^{\mathrm{2}} −\mathrm{3}} \Leftrightarrow\mathrm{x}^{\mathrm{2}} −\mathrm{3}=\mathrm{2x} \\ $$$$\Leftrightarrow\mathrm{x}^{\mathrm{2}} −\mathrm{2x}−\mathrm{3}=\mathrm{0}\:\Delta;'=\mathrm{1}+\mathrm{3}=\mathrm{2}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{x}=\mathrm{1}\pm\mathrm{2}\Rightarrow\boldsymbol{\mathrm{x}}\in\left\{−\mathrm{1},\mathrm{3}\right\}\: \\ $$$$\left.\mathrm{d}\right)\mathrm{log}_{\mathrm{4}} \left(\mathrm{x}+\mathrm{1}\right)−\mathrm{2log}_{\mathrm{4}} \left(\mathrm{x}+\mathrm{1}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}\right) \\ $$$$\mathrm{Condition}\:\mathrm{for}\:\mathrm{the}\:\mathrm{eqn}.\mathrm{is}\:\mathrm{deined}\:\mathrm{as}\:\mathrm{x}\geqslant−\mathrm{1} \\ $$$$\left(\mathrm{1}\right)\Leftrightarrow\mathrm{log}_{\mathrm{4}} \left(\mathrm{x}+\mathrm{1}\right)=−\frac{\mathrm{1}}{\mathrm{2}}\Leftrightarrow\mathrm{x}+\mathrm{1}=\mathrm{4}^{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$\Leftrightarrow\mathrm{x}+\mathrm{1}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{4}}}=\frac{\mathrm{1}}{\mathrm{2}}\Leftrightarrow\boldsymbol{\mathrm{x}}=−\frac{\mathrm{1}}{\mathrm{2}}\: \\ $$$$\left.\mathrm{Q3}.\mathrm{a}\right)\mathrm{y}=\sqrt{−\mathrm{3x}−\mathrm{5}}\Rightarrow\mathrm{y}'=\frac{−\mathrm{3}}{\mathrm{2}\sqrt{−\mathrm{3x}−\mathrm{5}}} \\ $$$$\left.\mathrm{b}\right)\mathrm{sin3x}=\mathrm{sin}\left(\mathrm{x}+\mathrm{2x}\right)=\mathrm{sinxcos2x}+\mathrm{cosxsin2x} \\ $$$$=\mathrm{sinx}\left(\mathrm{1}−\mathrm{2sin}^{\mathrm{2}} \mathrm{x}\right)+\mathrm{cosx}.\mathrm{2sinxcosx} \\ $$$$=\mathrm{sinx}−\mathrm{2sin}^{\mathrm{3}} \mathrm{x}+\mathrm{2sinx}\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}\right) \\ $$$$=\mathrm{3sinx}−\mathrm{4sin}^{\mathrm{3}} \mathrm{x}\:\left(\mathrm{q}.\mathrm{e}.\mathrm{d}\right) \\ $$$$\left.\mathrm{Q4}.\mathrm{a}\right)\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{Lim}}\frac{\mid\mathrm{x}+\mathrm{2}\mid−\mathrm{2}}{\mid\mathrm{x}\mid}=\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{x}+\mathrm{2}−\mathrm{2}}{\mid\mathrm{x}\mid} \\ $$$$=\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{x}}{\mid\mathrm{x}\mid}=\begin{cases}{\underset{\mathrm{x}\rightarrow−\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{x}}{−\mathrm{x}}=−\mathrm{1}}\\{\underset{\mathrm{x}\rightarrow+\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{x}}{\mathrm{x}}=\mathrm{1}}\end{cases} \\ $$$$\mathrm{Since}\:\mathrm{left}−\mathrm{limit}\neq\mathrm{right}−\mathrm{limit} \\ $$$$\mathrm{the}\:\mathrm{given}\:\mathrm{limit}\:\mathrm{don}'\mathrm{t}\:\mathrm{exist}\: \\ $$$$\left.\mathrm{b}\right)\underset{\mathrm{x}\rightarrow−\mathrm{2}} {\mathrm{lim}}\frac{\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{1}}{\mathrm{2}}}{\mathrm{x}^{\mathrm{3}} +\mathrm{8}}\underset{\mathrm{x}\rightarrow−\mathrm{2}} {=\mathrm{lim}}\frac{\frac{\mathrm{x}+\mathrm{2}}{\mathrm{2x}}}{\left(\mathrm{x}+\mathrm{2}\right)\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{4}\right)}= \\ $$$$=\underset{\mathrm{x}\rightarrow−\mathrm{2}} {\mathrm{lim}}\:\frac{\mathrm{1}}{\mathrm{2x}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{4}\right)}=\frac{\mathrm{1}}{−\mathrm{4}\left(\mathrm{4}+\mathrm{2}+\mathrm{4}\right)}=\frac{−\mathrm{1}}{\mathrm{40}} \\ $$$$\left.\mathrm{c}\right)\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{\mathrm{x}^{−\mathrm{1}} −\mathrm{2}}{\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}}=\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{\frac{\mathrm{1}}{\mathrm{x}}−\mathrm{2}}{\frac{\mathrm{2x}−\mathrm{1}}{\mathrm{2}}}=\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{\frac{\mathrm{1}−\mathrm{2x}}{\mathrm{x}}}{\frac{\mathrm{2x}−\mathrm{1}}{\mathrm{2}}} \\ $$$$=\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{−\mathrm{2}}{\mathrm{x}}=−\mathrm{4} \\ $$$$\left.\mathrm{d}\right)\underset{\mathrm{x}\rightarrow\mathrm{1}} {\mathrm{li}\:\mathrm{m}}\frac{\mathrm{sin}\left(\mathrm{x}−\mathrm{1}\right)}{\mathrm{x}^{\mathrm{2}} +\mathrm{2x}−\mathrm{3}}\:\underset{\mathrm{x}−\mathrm{1}=\mathrm{t}} {\:\:=\:}\:\:\underset{\mathrm{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\mathrm{sin}\left(\mathrm{x}−\mathrm{1}\right)}{\left(\mathrm{x}−\mathrm{1}\right)\left(\mathrm{x}+\mathrm{3}\right)} \\ $$$$=\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sint}}{\mathrm{t}\left(\mathrm{t}+\mathrm{4}\right)} \\ $$$$=\underset{\mathrm{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sint}}{\mathrm{t}}×\frac{\mathrm{1}}{\mathrm{t}+\mathrm{4}}=\mathrm{1}×\frac{\mathrm{1}}{\mathrm{4}}=\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\left.\mathrm{e}\right)\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{\mathrm{6}\sqrt{\mathrm{x}}−\mathrm{6}\sqrt{\mathrm{2x}−\mathrm{1}}}{\mathrm{x}−\mathrm{1}}=\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{\mathrm{6}\left[\mathrm{x}−\left(\mathrm{2x}−\mathrm{1}\right)\right.}{\left(\mathrm{x}−\mathrm{1}\right)\left(\sqrt{\mathrm{x}}+\sqrt{\mathrm{2x}−\mathrm{1}}\right)} \\ $$$$=\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{−\mathrm{6}\left(\mathrm{x}−\mathrm{1}\right)}{\left(\mathrm{x}−\mathrm{1}\right)\left(\sqrt{\mathrm{x}}+\sqrt{\mathrm{2x}−\mathrm{1}}\right)}=\underset{\mathrm{x}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}} {\mathrm{lim}}\frac{−\mathrm{6}}{\:\sqrt{\mathrm{x}}+\sqrt{\mathrm{2x}−\mathrm{1}}} \\ $$$$=\frac{−\mathrm{6}}{\:\sqrt{\frac{\mathrm{1}}{\mathrm{2}}}}=−\mathrm{6}\sqrt{\mathrm{2}} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply