Question-106727

Question Number 106727 by Algoritm last updated on 06/Aug/20

Answered by Her_Majesty last updated on 06/Aug/20

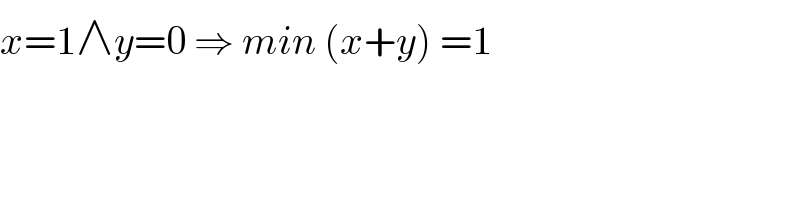
$${x}=\mathrm{1}\wedge{y}=\mathrm{0}\:\Rightarrow\:{min}\:\left({x}+{y}\right)\:=\mathrm{1} \\ $$

Commented by Her_Majesty last updated on 06/Aug/20

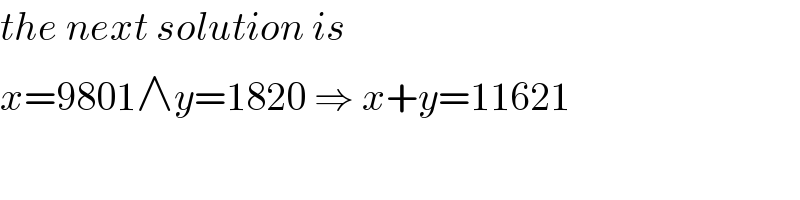
the next solution is x=9801∧y=1820 ⇒ x+y=11621

$${the}\:{next}\:{solution}\:{is} \\ $$$${x}=\mathrm{9801}\wedge{y}=\mathrm{1820}\:\Rightarrow\:{x}+{y}=\mathrm{11621} \\ $$

Commented by mathmax by abdo last updated on 07/Aug/20

$$\mathrm{can}\:\mathrm{you}\:\mathrm{show}\:\mathrm{the}\:\mathrm{work}\:… \\ $$

Commented by Her_Majesty last updated on 07/Aug/20

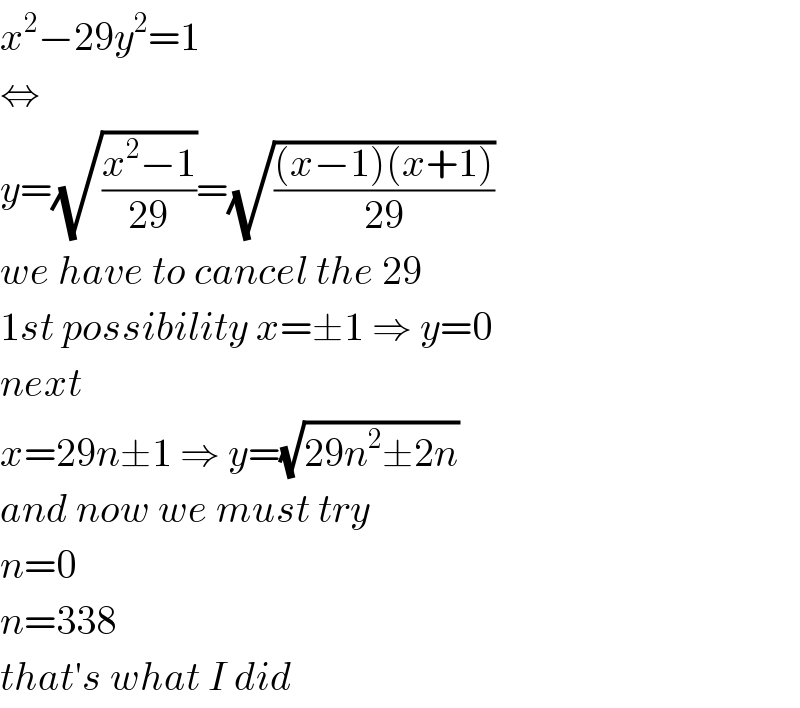
x^2 −29y^2 =1 ⇔ y=(√((x^2 −1)/(29)))=(√(((x−1)(x+1))/(29))) we have to cancel the 29 1st possibility x=±1 ⇒ y=0 next x=29n±1 ⇒ y=(√(29n^2 ±2n)) and now we must try n=0 n=338 that′s what I did

$${x}^{\mathrm{2}} −\mathrm{29}{y}^{\mathrm{2}} =\mathrm{1} \\ $$$$\Leftrightarrow \\ $$$${y}=\sqrt{\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\mathrm{29}}}=\sqrt{\frac{\left({x}−\mathrm{1}\right)\left({x}+\mathrm{1}\right)}{\mathrm{29}}} \\ $$$${we}\:{have}\:{to}\:{cancel}\:{the}\:\mathrm{29} \\ $$$$\mathrm{1}{st}\:{possibility}\:{x}=\pm\mathrm{1}\:\Rightarrow\:{y}=\mathrm{0} \\ $$$${next} \\ $$$${x}=\mathrm{29}{n}\pm\mathrm{1}\:\Rightarrow\:{y}=\sqrt{\mathrm{29}{n}^{\mathrm{2}} \pm\mathrm{2}{n}} \\ $$$${and}\:{now}\:{we}\:{must}\:{try} \\ $$$${n}=\mathrm{0} \\ $$$${n}=\mathrm{338} \\ $$$${that}'{s}\:{what}\:{I}\:{did} \\ $$

Answered by mathmax by abdo last updated on 06/Aug/20

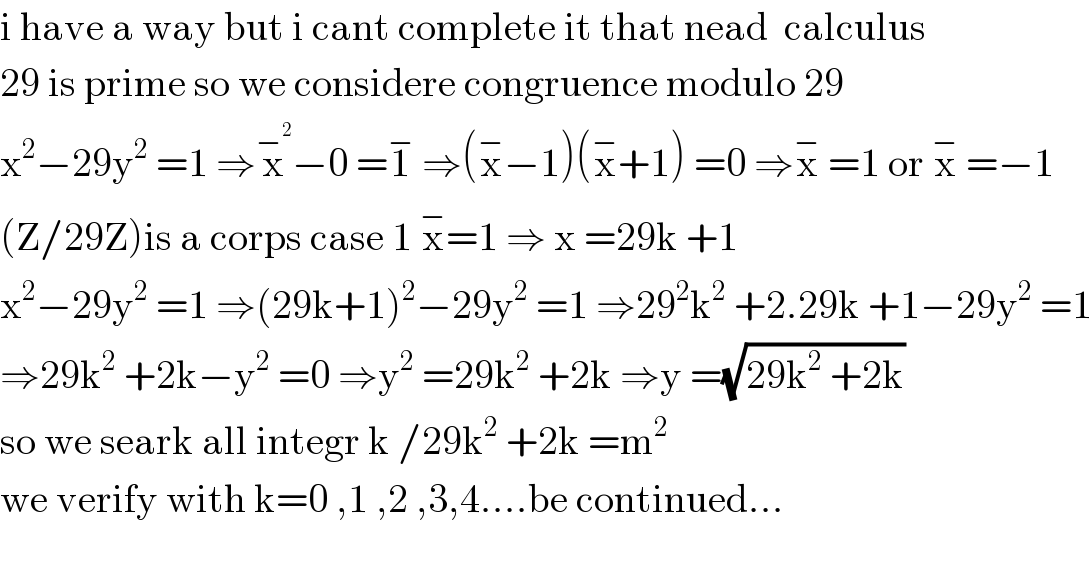
i have a way but i cant complete it that nead calculus 29 is prime so we considere congruence modulo 29 x^2 −29y^2 =1 ⇒x^−^2 −0 =1^− ⇒(x^− −1)(x^− +1) =0 ⇒x^− =1 or x^− =−1 (Z/29Z)is a corps case 1 x^− =1 ⇒ x =29k +1 x^2 −29y^2 =1 ⇒(29k+1)^2 −29y^2 =1 ⇒29^2 k^2 +2.29k +1−29y^2 =1 ⇒29k^2 +2k−y^2 =0 ⇒y^2 =29k^2 +2k ⇒y =(√(29k^2 +2k)) so we seark all integr k /29k^2 +2k =m^(2 ) we verify with k=0 ,1 ,2 ,3,4....be continued...

$$\mathrm{i}\:\mathrm{have}\:\mathrm{a}\:\mathrm{way}\:\mathrm{but}\:\mathrm{i}\:\mathrm{cant}\:\mathrm{complete}\:\mathrm{it}\:\mathrm{that}\:\mathrm{nead}\:\:\mathrm{calculus} \\ $$$$\mathrm{29}\:\mathrm{is}\:\mathrm{prime}\:\mathrm{so}\:\mathrm{we}\:\mathrm{considere}\:\mathrm{congruence}\:\mathrm{modulo}\:\mathrm{29} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{29y}^{\mathrm{2}} \:=\mathrm{1}\:\Rightarrow\overset{−^{\mathrm{2}} } {\mathrm{x}}−\mathrm{0}\:=\overset{−} {\mathrm{1}}\:\Rightarrow\left(\overset{−} {\mathrm{x}}−\mathrm{1}\right)\left(\overset{−} {\mathrm{x}}+\mathrm{1}\right)\:=\mathrm{0}\:\Rightarrow\overset{−} {\mathrm{x}}\:=\mathrm{1}\:\mathrm{or}\:\overset{−} {\mathrm{x}}\:=−\mathrm{1} \\ $$$$\left(\mathrm{Z}/\mathrm{29Z}\right)\mathrm{is}\:\mathrm{a}\:\mathrm{corps}\:\mathrm{case}\:\mathrm{1}\:\overset{−} {\mathrm{x}}=\mathrm{1}\:\Rightarrow\:\mathrm{x}\:=\mathrm{29k}\:+\mathrm{1} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{29y}^{\mathrm{2}} \:=\mathrm{1}\:\Rightarrow\left(\mathrm{29k}+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{29y}^{\mathrm{2}} \:=\mathrm{1}\:\Rightarrow\mathrm{29}^{\mathrm{2}} \mathrm{k}^{\mathrm{2}} \:+\mathrm{2}.\mathrm{29k}\:+\mathrm{1}−\mathrm{29y}^{\mathrm{2}} \:=\mathrm{1} \\ $$$$\Rightarrow\mathrm{29k}^{\mathrm{2}} \:+\mathrm{2k}−\mathrm{y}^{\mathrm{2}} \:=\mathrm{0}\:\Rightarrow\mathrm{y}^{\mathrm{2}} \:=\mathrm{29k}^{\mathrm{2}} \:+\mathrm{2k}\:\Rightarrow\mathrm{y}\:=\sqrt{\mathrm{29k}^{\mathrm{2}} \:+\mathrm{2k}} \\ $$$$\mathrm{so}\:\mathrm{we}\:\mathrm{seark}\:\mathrm{all}\:\mathrm{integr}\:\mathrm{k}\:/\mathrm{29k}^{\mathrm{2}} \:+\mathrm{2k}\:=\mathrm{m}^{\mathrm{2}\:} \\ $$$$\mathrm{we}\:\mathrm{verify}\:\mathrm{with}\:\mathrm{k}=\mathrm{0}\:,\mathrm{1}\:,\mathrm{2}\:,\mathrm{3},\mathrm{4}….\mathrm{be}\:\mathrm{continued}… \\ $$$$ \\ $$

Commented by Algoritm last updated on 07/Aug/20