Question Number 107234 by O Predador last updated on 09/Aug/20

Answered by floor(10²Eta[1]) last updated on 09/Aug/20
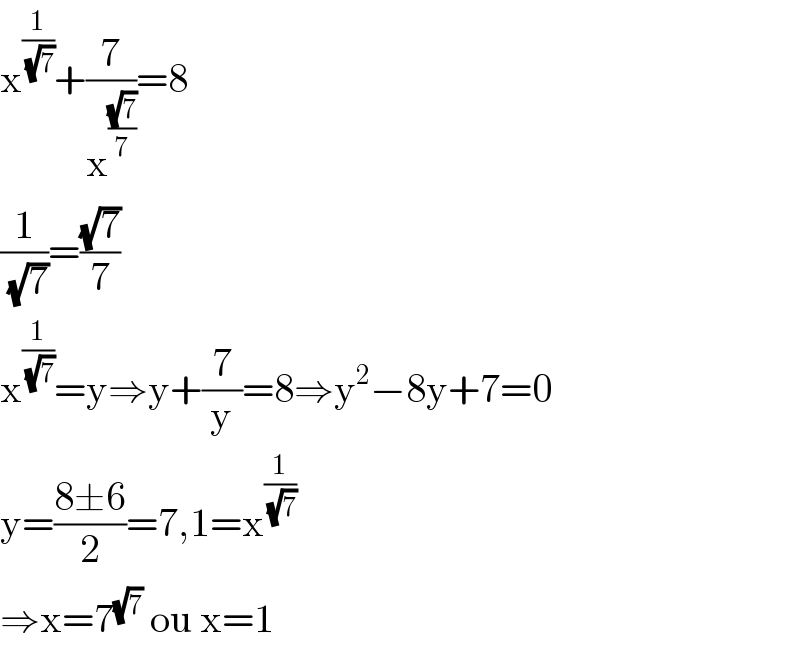
$$\mathrm{x}^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{7}}}} +\frac{\mathrm{7}}{\mathrm{x}^{\frac{\sqrt{\mathrm{7}}}{\mathrm{7}}} }=\mathrm{8} \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\mathrm{7}}}=\frac{\sqrt{\mathrm{7}}}{\mathrm{7}} \\ $$$$\mathrm{x}^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{7}}}} =\mathrm{y}\Rightarrow\mathrm{y}+\frac{\mathrm{7}}{\mathrm{y}}=\mathrm{8}\Rightarrow\mathrm{y}^{\mathrm{2}} −\mathrm{8y}+\mathrm{7}=\mathrm{0} \\ $$$$\mathrm{y}=\frac{\mathrm{8}\pm\mathrm{6}}{\mathrm{2}}=\mathrm{7},\mathrm{1}=\mathrm{x}^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{7}}}} \\ $$$$\Rightarrow\mathrm{x}=\mathrm{7}^{\sqrt{\mathrm{7}}} \:\mathrm{ou}\:\mathrm{x}=\mathrm{1} \\ $$
