Question Number 107794 by udaythool last updated on 12/Aug/20

Answered by arcana last updated on 12/Aug/20

$$\mathrm{Considere} \\ $$$${f}\left({x}\right)={x}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} } \\ $$$$\Rightarrow{f}\:'\left({x}\right)=\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }−\frac{{x}^{\mathrm{2}} }{\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}=\mathrm{0}, \\ $$$$\mathrm{luego}\:\mathrm{1}−\mathrm{2}{x}^{\mathrm{2}} =\mathrm{0}\Rightarrow{x}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}. \\ $$$$\mathrm{Decimos}\:\mathrm{que}\:\left(\mathrm{1}/\sqrt{\mathrm{2}},{f}\left(\mathrm{1}/\sqrt{\mathrm{2}}\right)\right)\:\mathrm{es}\:\mathrm{punto} \\ $$$$\mathrm{maximo}\:\mathrm{de}\:{f}. \\ $$$$\mathrm{Por}\:\mathrm{tanto},\:{f}\left({x}\right)\leqslant{f}\left(\mathrm{1}/\sqrt{\mathrm{2}}\right). \\ $$$$\mathrm{Como}\:\mid{z}\mid={x}^{\mathrm{2}} +{y}^{\mathrm{2}} \leqslant\mathrm{1},\:\mathrm{entonces} \\ $$$${xy}\leqslant{x}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\leqslant\mathrm{1}/\mathrm{2}. \\ $$$$ \\ $$
Commented by udaythool last updated on 13/Aug/20
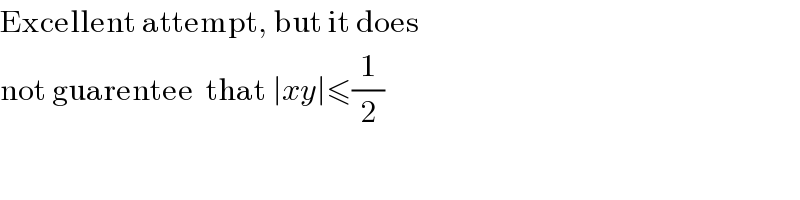
$$\mathrm{Excellent}\:\mathrm{attempt},\:\mathrm{but}\:\mathrm{it}\:\mathrm{does} \\ $$$$\mathrm{not}\:\mathrm{guarentee}\:\:\mathrm{that}\:\mid{xy}\mid\leqslant\frac{\mathrm{1}}{\mathrm{2}} \\ $$
Commented by udaythool last updated on 14/Aug/20

$$\mathrm{I}\:\mathrm{think}\:\mathrm{by}\:\mathrm{contradiction}\:\mathrm{method} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{prove}\:\mathrm{it}\:\mathrm{easily}; \\ $$$$\mathrm{Suppose}\:\forall{z}={x}+{iy}\in{A},\:\mid{xy}\mid\nleqslant\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\exists\:\mathrm{some}\:{z}={x}+{iy}\:\in{A}\:\mathrm{such}\:\mathrm{that} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}<\mid{xy}\mid \\ $$$$\mathrm{But}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} \leqslant\mathrm{1}\:\mathrm{hence} \\ $$$$\mathrm{0}\geqslant{x}^{\mathrm{2}} +{y}^{\mathrm{2}} −\mathrm{1}>\left(\frac{\mathrm{1}}{\mathrm{2}{y}}−{y}\right)^{\mathrm{2}} \geqslant\mathrm{0} \\ $$$$\mathrm{which}\:\mathrm{is}\:\mathrm{absurd}… \\ $$
Answered by mr W last updated on 12/Aug/20
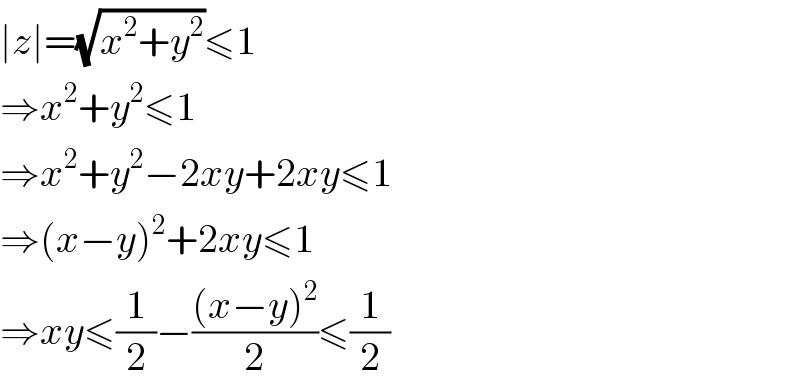
$$\mid{z}\mid=\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }\leqslant\mathrm{1} \\ $$$$\Rightarrow{x}^{\mathrm{2}} +{y}^{\mathrm{2}} \leqslant\mathrm{1} \\ $$$$\Rightarrow{x}^{\mathrm{2}} +{y}^{\mathrm{2}} −\mathrm{2}{xy}+\mathrm{2}{xy}\leqslant\mathrm{1} \\ $$$$\Rightarrow\left({x}−{y}\right)^{\mathrm{2}} +\mathrm{2}{xy}\leqslant\mathrm{1} \\ $$$$\Rightarrow{xy}\leqslant\frac{\mathrm{1}}{\mathrm{2}}−\frac{\left({x}−{y}\right)^{\mathrm{2}} }{\mathrm{2}}\leqslant\frac{\mathrm{1}}{\mathrm{2}} \\ $$
