Question Number 107999 by Don08q last updated on 13/Aug/20

Commented by udaythool last updated on 13/Aug/20

$$\Rightarrow\mathrm{4}{x}^{\mathrm{2}} +\mathrm{3}{xy}−{y}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\left({x}+{y}\right)\left(\mathrm{4}{x}−{y}\right)=\mathrm{0} \\ $$$$\Rightarrow\mathrm{4}{x}={y};\:\:\:\left(\because\:{x}+{y}\neq\mathrm{0}\right) \\ $$$$\Rightarrow\mathrm{4}{x}^{\mathrm{2}} ={xy} \\ $$$$\Rightarrow\mathrm{5}{xy}=\mathrm{5} \\ $$$$\Rightarrow{xy}=\mathrm{1} \\ $$
Commented by Don08q last updated on 13/Aug/20

$${Thanks}\:{Sir} \\ $$
Commented by Rasheed.Sindhi last updated on 13/Aug/20
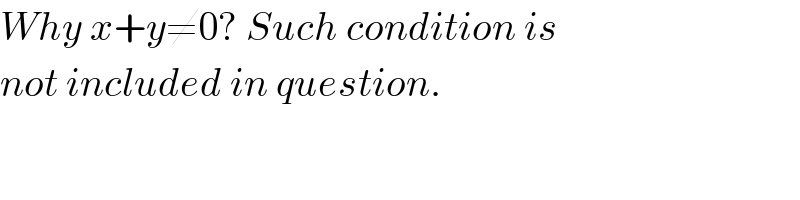
$${Why}\:{x}+{y}\neq\mathrm{0}?\:{Such}\:{condition}\:{is} \\ $$$${not}\:{included}\:{in}\:{question}. \\ $$
Commented by Sarah85 last updated on 13/Aug/20

$$\mathrm{if}\:{x}+{y}=\mathrm{0}\:\Leftrightarrow\:{y}=−{x}\:\Leftrightarrow\:{x}=−{y} \\ $$$$\mathrm{4}{x}^{\mathrm{2}} −\mathrm{4}{x}^{\mathrm{2}} =\mathrm{5} \\ $$$${y}^{\mathrm{2}} −{y}^{\mathrm{2}} =\mathrm{5} \\ $$$$\mathrm{both}\:\mathrm{are}\:\mathrm{impossible} \\ $$
Commented by Rasheed.Sindhi last updated on 14/Aug/20
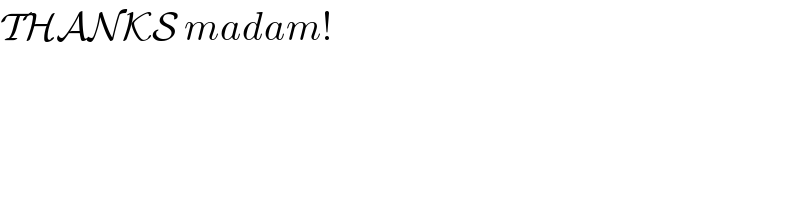
$$\mathcal{THANKS}\:{madam}! \\ $$
Answered by Sarah85 last updated on 13/Aug/20
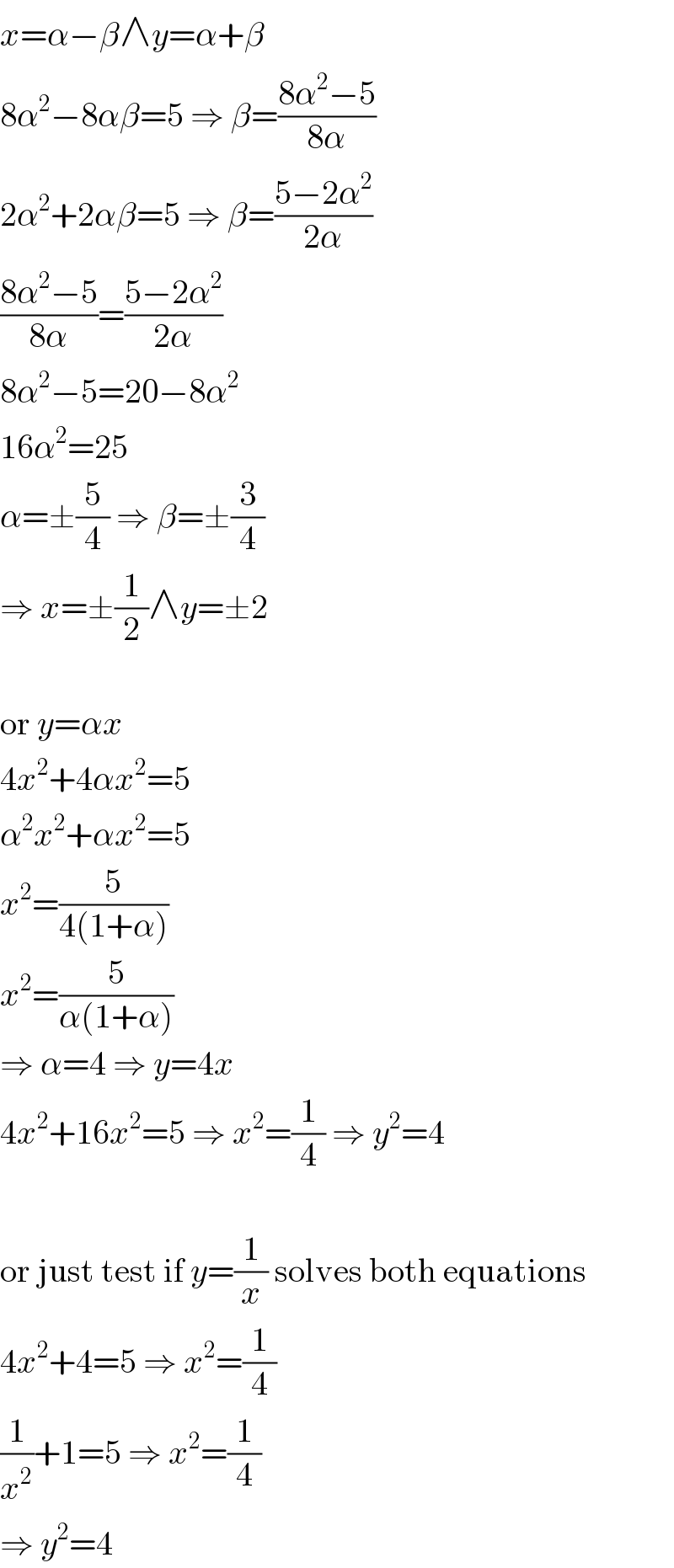
$${x}=\alpha−\beta\wedge{y}=\alpha+\beta \\ $$$$\mathrm{8}\alpha^{\mathrm{2}} −\mathrm{8}\alpha\beta=\mathrm{5}\:\Rightarrow\:\beta=\frac{\mathrm{8}\alpha^{\mathrm{2}} −\mathrm{5}}{\mathrm{8}\alpha} \\ $$$$\mathrm{2}\alpha^{\mathrm{2}} +\mathrm{2}\alpha\beta=\mathrm{5}\:\Rightarrow\:\beta=\frac{\mathrm{5}−\mathrm{2}\alpha^{\mathrm{2}} }{\mathrm{2}\alpha} \\ $$$$\frac{\mathrm{8}\alpha^{\mathrm{2}} −\mathrm{5}}{\mathrm{8}\alpha}=\frac{\mathrm{5}−\mathrm{2}\alpha^{\mathrm{2}} }{\mathrm{2}\alpha} \\ $$$$\mathrm{8}\alpha^{\mathrm{2}} −\mathrm{5}=\mathrm{20}−\mathrm{8}\alpha^{\mathrm{2}} \\ $$$$\mathrm{16}\alpha^{\mathrm{2}} =\mathrm{25} \\ $$$$\alpha=\pm\frac{\mathrm{5}}{\mathrm{4}}\:\Rightarrow\:\beta=\pm\frac{\mathrm{3}}{\mathrm{4}} \\ $$$$\Rightarrow\:{x}=\pm\frac{\mathrm{1}}{\mathrm{2}}\wedge{y}=\pm\mathrm{2} \\ $$$$ \\ $$$$\mathrm{or}\:{y}=\alpha{x} \\ $$$$\mathrm{4}{x}^{\mathrm{2}} +\mathrm{4}\alpha{x}^{\mathrm{2}} =\mathrm{5} \\ $$$$\alpha^{\mathrm{2}} {x}^{\mathrm{2}} +\alpha{x}^{\mathrm{2}} =\mathrm{5} \\ $$$${x}^{\mathrm{2}} =\frac{\mathrm{5}}{\mathrm{4}\left(\mathrm{1}+\alpha\right)} \\ $$$${x}^{\mathrm{2}} =\frac{\mathrm{5}}{\alpha\left(\mathrm{1}+\alpha\right)} \\ $$$$\Rightarrow\:\alpha=\mathrm{4}\:\Rightarrow\:{y}=\mathrm{4}{x} \\ $$$$\mathrm{4}{x}^{\mathrm{2}} +\mathrm{16}{x}^{\mathrm{2}} =\mathrm{5}\:\Rightarrow\:{x}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{4}}\:\Rightarrow\:{y}^{\mathrm{2}} =\mathrm{4} \\ $$$$ \\ $$$$\mathrm{or}\:\mathrm{just}\:\mathrm{test}\:\mathrm{if}\:{y}=\frac{\mathrm{1}}{{x}}\:\mathrm{solves}\:\mathrm{both}\:\mathrm{equations} \\ $$$$\mathrm{4}{x}^{\mathrm{2}} +\mathrm{4}=\mathrm{5}\:\Rightarrow\:{x}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\frac{\mathrm{1}}{{x}^{\mathrm{2}} }+\mathrm{1}=\mathrm{5}\:\Rightarrow\:{x}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\Rightarrow\:{y}^{\mathrm{2}} =\mathrm{4} \\ $$
Answered by 1549442205PVT last updated on 14/Aug/20
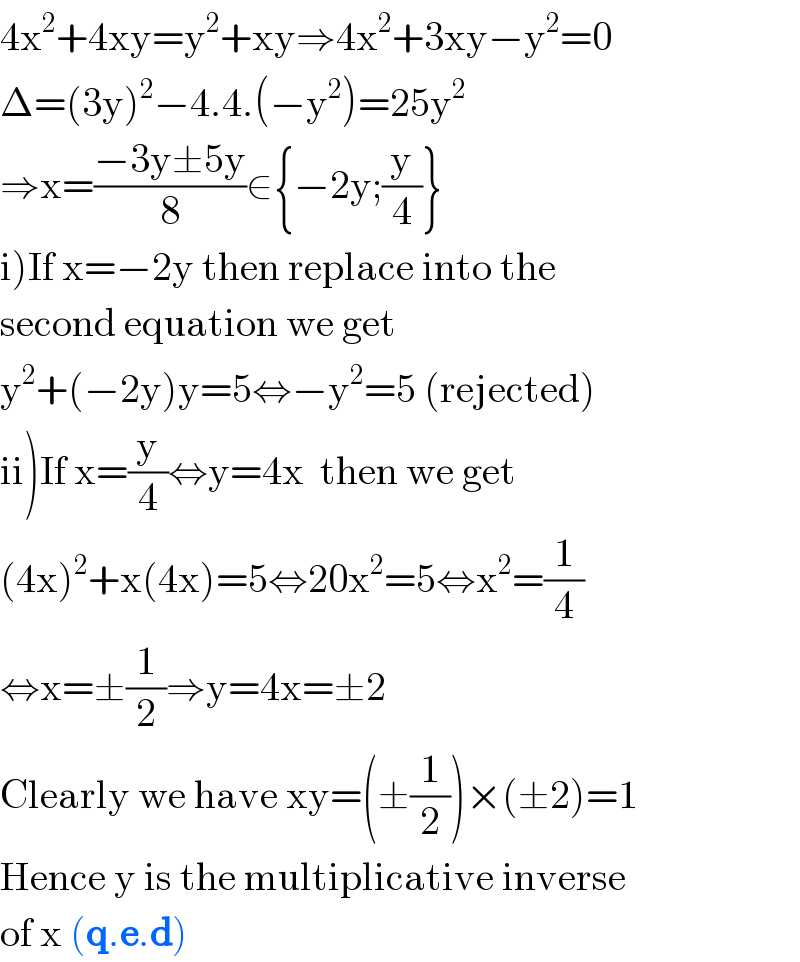
$$\mathrm{4x}^{\mathrm{2}} +\mathrm{4xy}=\mathrm{y}^{\mathrm{2}} +\mathrm{xy}\Rightarrow\mathrm{4x}^{\mathrm{2}} +\mathrm{3xy}−\mathrm{y}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Delta=\left(\mathrm{3y}\right)^{\mathrm{2}} −\mathrm{4}.\mathrm{4}.\left(−\mathrm{y}^{\mathrm{2}} \right)=\mathrm{25y}^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{x}=\frac{−\mathrm{3y}\pm\mathrm{5y}}{\mathrm{8}}\in\left\{−\mathrm{2y};\frac{\mathrm{y}}{\mathrm{4}}\right\} \\ $$$$\left.\mathrm{i}\right)\mathrm{If}\:\mathrm{x}=−\mathrm{2y}\:\mathrm{then}\:\mathrm{replace}\:\mathrm{into}\:\mathrm{the} \\ $$$$\mathrm{second}\:\mathrm{equation}\:\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{y}^{\mathrm{2}} +\left(−\mathrm{2y}\right)\mathrm{y}=\mathrm{5}\Leftrightarrow−\mathrm{y}^{\mathrm{2}} =\mathrm{5}\:\left(\mathrm{rejected}\right) \\ $$$$\left.\mathrm{ii}\right)\mathrm{If}\:\mathrm{x}=\frac{\mathrm{y}}{\mathrm{4}}\Leftrightarrow\mathrm{y}=\mathrm{4x}\:\:\mathrm{then}\:\mathrm{we}\:\mathrm{get} \\ $$$$\left(\mathrm{4x}\right)^{\mathrm{2}} +\mathrm{x}\left(\mathrm{4x}\right)=\mathrm{5}\Leftrightarrow\mathrm{20x}^{\mathrm{2}} =\mathrm{5}\Leftrightarrow\mathrm{x}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\Leftrightarrow\mathrm{x}=\pm\frac{\mathrm{1}}{\mathrm{2}}\Rightarrow\mathrm{y}=\mathrm{4x}=\pm\mathrm{2} \\ $$$$\mathrm{Clearly}\:\mathrm{we}\:\mathrm{have}\:\mathrm{xy}=\left(\pm\frac{\mathrm{1}}{\mathrm{2}}\right)×\left(\pm\mathrm{2}\right)=\mathrm{1} \\ $$$$\mathrm{Hence}\:\mathrm{y}\:\mathrm{is}\:\mathrm{the}\:\mathrm{multiplicative}\:\mathrm{inverse} \\ $$$$\mathrm{of}\:\mathrm{x}\:\left(\boldsymbol{\mathrm{q}}.\boldsymbol{\mathrm{e}}.\boldsymbol{\mathrm{d}}\right) \\ $$
Commented by Don08q last updated on 14/Aug/20

$${Thanks}\:{Sir} \\ $$
Answered by Rasheed.Sindhi last updated on 14/Aug/20

$${y}\:{is}\:{inverse}\:{of}\:{x}\Rightarrow{xy}=\mathrm{1}.\mathrm{So}\:\mathrm{now} \\ $$$$\mathrm{the}\:\mathrm{question}\:\mathrm{in}\:\mathrm{other}\:\mathrm{words}\:\mathrm{is}: \\ $$$$“{To}\:{show}\:{the}\:{following}\:{system} \\ $$$$\:\:\:\boldsymbol{{consistent}}\:'': \\ $$$$\:\begin{cases}{\mathrm{4}{x}^{\mathrm{2}} +\mathrm{4}{xy}=\mathrm{5}}\\{{y}^{\mathrm{2}} +{xy}=\mathrm{5}}\\{{xy}=\mathrm{1}}\end{cases} \\ $$$${I}-{E}\:\:{the}\:{system}\:{has}\:{common}\: \\ $$$${solution}. \\ $$$$\:\Rightarrow\begin{cases}{\mathrm{4}{x}^{\mathrm{2}} +\mathrm{4}\left(\mathrm{1}\right)=\mathrm{5}\Rightarrow{x}=\pm\frac{\mathrm{1}}{\mathrm{2}}}\\{{y}^{\mathrm{2}} +\left(\mathrm{1}\right)=\mathrm{5}\Rightarrow{y}=\pm\mathrm{2}}\end{cases} \\ $$$${Since}\:\left(\frac{\mathrm{1}}{\mathrm{2}},\mathrm{2}\right)\:\&\:\left(−\frac{\mathrm{1}}{\mathrm{2}},−\mathrm{2}\right)\:{are} \\ $$$${solutions}\:{satisfying}\:{the}\:{system} \\ $$$${so}\:{the}\:{system}\:{is}\:\boldsymbol{{consistent}}. \\ $$$$\left({Evident}\:{from}\:{solutions}\:{that}\right. \\ $$$$\left.{y}\:{is}\:{multiplicative}\:{inverse}\:{of}\:{x}.\right) \\ $$
Commented by Don08q last updated on 14/Aug/20

$${Thanks}\:{Sir} \\ $$
