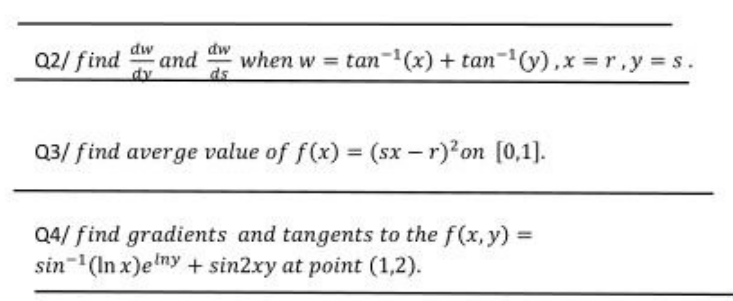
Question Number 108652 by mohammad17 last updated on 18/Aug/20
Commented by mohammad17 last updated on 18/Aug/20
$${sir}\:{are}\:{you}\:{can}\:{help}\:{me}\:{pleas}\:? \\ $$
Answered by Aziztisffola last updated on 18/Aug/20
![Q2) (dw/dy)=(1/(1+y^2 )) (dw/ds)=(1/(1+s^2 )) Q3) (1/(1−0))∫_0 ^( 1) f(x)dx=∫_0 ^( 1) (sx−r)^2 dx =[(1/(3s))(sx−r)^3 ]_0 ^1 =(1/(3s))((s−r)^3 −(−r)^3 ) =(((s−r)^3 +r^3 )/(3s))](https://www.tinkutara.com/question/Q108662.png)
$$\left.\mathrm{Q2}\right)\:\frac{\mathrm{dw}}{\mathrm{dy}}=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{y}^{\mathrm{2}} }\:\: \\ $$$$\:\frac{\mathrm{dw}}{\mathrm{ds}}=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{s}^{\mathrm{2}} } \\ $$$$\left.\mathrm{Q3}\right)\:\frac{\mathrm{1}}{\mathrm{1}−\mathrm{0}}\int_{\mathrm{0}} ^{\:\mathrm{1}} \mathrm{f}\left(\mathrm{x}\right)\mathrm{dx}=\int_{\mathrm{0}} ^{\:\mathrm{1}} \left(\mathrm{sx}−\mathrm{r}\right)^{\mathrm{2}} \mathrm{dx} \\ $$$$=\left[\frac{\mathrm{1}}{\mathrm{3s}}\left(\mathrm{sx}−\mathrm{r}\right)^{\mathrm{3}} \right]_{\mathrm{0}} ^{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{3s}}\left(\left(\mathrm{s}−\mathrm{r}\right)^{\mathrm{3}} −\left(−\mathrm{r}\right)^{\mathrm{3}} \right) \\ $$$$=\frac{\left(\mathrm{s}−\mathrm{r}\right)^{\mathrm{3}} +\mathrm{r}^{\mathrm{3}} }{\mathrm{3s}} \\ $$
