Menu Close

Question-109369

Tinku Tara 0 Comments
Pin




Question Number 109369 by I want to learn more last updated on 23/Aug/20
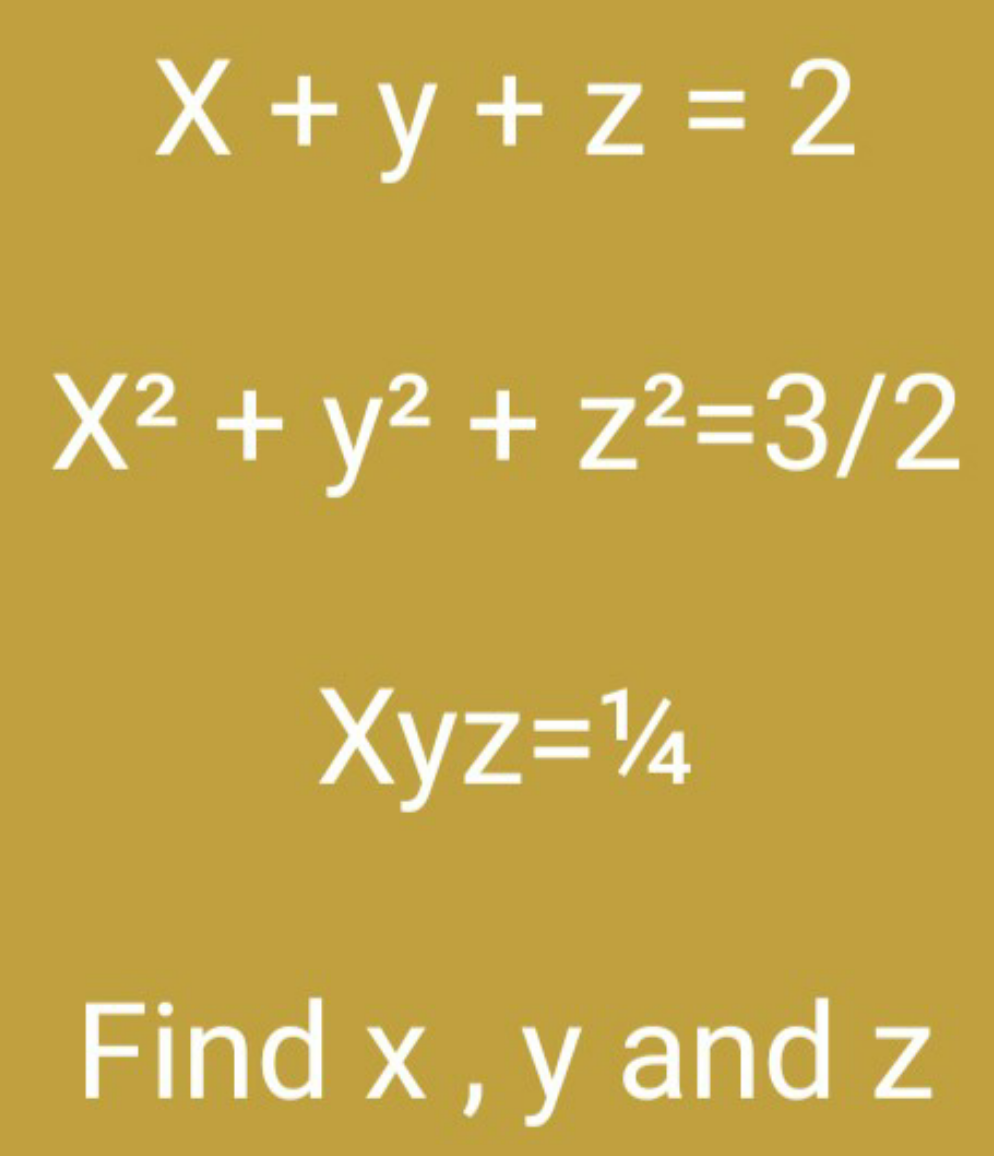
Answered by floor(10²Eta[1]) last updated on 23/Aug/20
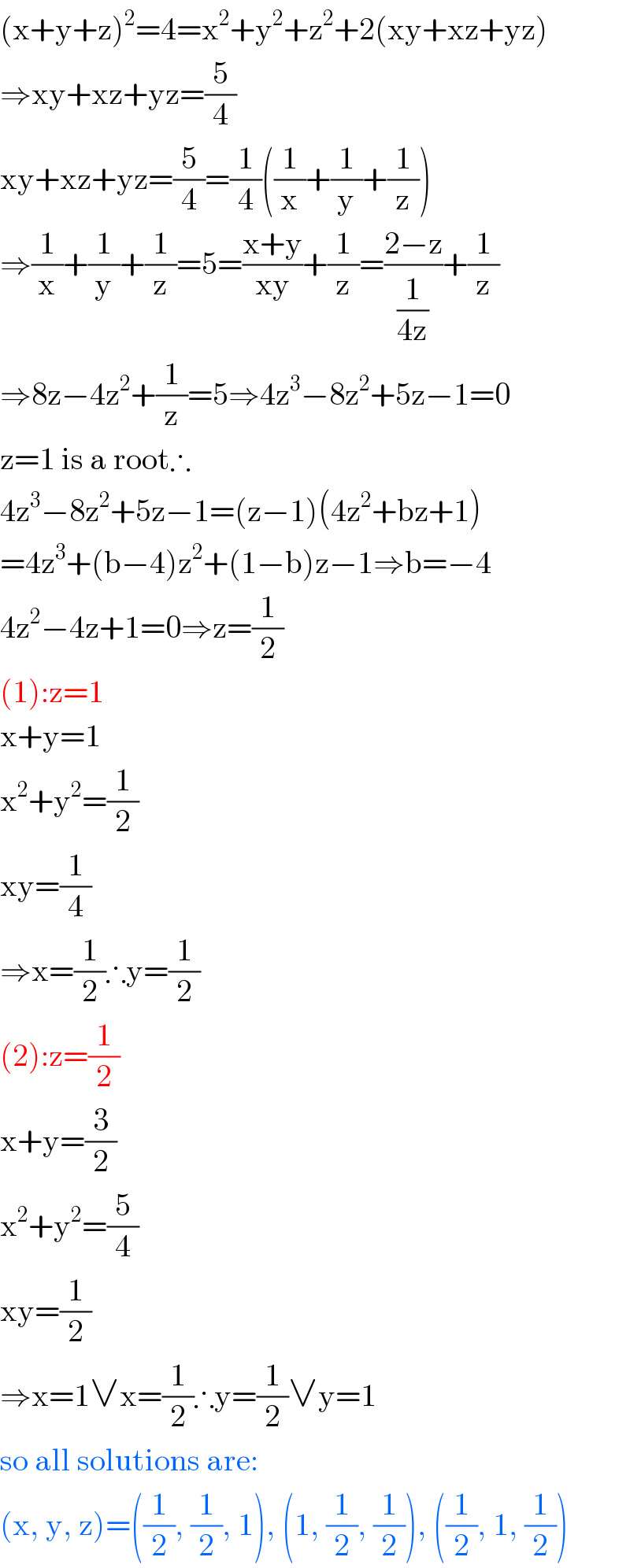
(x+y+z)^2 =4=x^2 +y^2 +z^2 +2(xy+xz+yz) ⇒xy+xz+yz=(5/4) xy+xz+yz=(5/4)=(1/4)((1/x)+(1/y)+(1/z)) ⇒(1/x)+(1/y)+(1/z)=5=((x+y)/(xy))+(1/z)=((2−z)/(1/(4z)))+(1/z) ⇒8z−4z^2 +(1/z)=5⇒4z^3 −8z^2 +5z−1=0 z=1 is a root∴ 4z^3 −8z^2 +5z−1=(z−1)(4z^2 +bz+1) =4z^3 +(b−4)z^2 +(1−b)z−1⇒b=−4 4z^2 −4z+1=0⇒z=(1/2) (1):z=1 x+y=1 x^2 +y^2 =(1/2) xy=(1/4) ⇒x=(1/2)∴y=(1/2) (2):z=(1/2) x+y=(3/2) x^2 +y^2 =(5/4) xy=(1/2) ⇒x=1∨x=(1/2)∴y=(1/2)∨y=1 so all solutions are: (x, y, z)=((1/2), (1/2), 1), (1, (1/2), (1/2)), ((1/2), 1, (1/2))
$$\left(\mathrm{x}+\mathrm{y}+\mathrm{z}\right)^{\mathrm{2}} =\mathrm{4}=\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} +\mathrm{2}\left(\mathrm{xy}+\mathrm{xz}+\mathrm{yz}\right) \\ $$$$\Rightarrow\mathrm{xy}+\mathrm{xz}+\mathrm{yz}=\frac{\mathrm{5}}{\mathrm{4}} \\ $$$$\mathrm{xy}+\mathrm{xz}+\mathrm{yz}=\frac{\mathrm{5}}{\mathrm{4}}=\frac{\mathrm{1}}{\mathrm{4}}\left(\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{1}}{\mathrm{y}}+\frac{\mathrm{1}}{\mathrm{z}}\right) \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{x}}+\frac{\mathrm{1}}{\mathrm{y}}+\frac{\mathrm{1}}{\mathrm{z}}=\mathrm{5}=\frac{\mathrm{x}+\mathrm{y}}{\mathrm{xy}}+\frac{\mathrm{1}}{\mathrm{z}}=\frac{\mathrm{2}−\mathrm{z}}{\frac{\mathrm{1}}{\mathrm{4z}}}+\frac{\mathrm{1}}{\mathrm{z}} \\ $$$$\Rightarrow\mathrm{8z}−\mathrm{4z}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{z}}=\mathrm{5}\Rightarrow\mathrm{4z}^{\mathrm{3}} −\mathrm{8z}^{\mathrm{2}} +\mathrm{5z}−\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{z}=\mathrm{1}\:\mathrm{is}\:\mathrm{a}\:\mathrm{root}\therefore \\ $$$$\mathrm{4z}^{\mathrm{3}} −\mathrm{8z}^{\mathrm{2}} +\mathrm{5z}−\mathrm{1}=\left(\mathrm{z}−\mathrm{1}\right)\left(\mathrm{4z}^{\mathrm{2}} +\mathrm{bz}+\mathrm{1}\right) \\ $$$$=\mathrm{4z}^{\mathrm{3}} +\left(\mathrm{b}−\mathrm{4}\right)\mathrm{z}^{\mathrm{2}} +\left(\mathrm{1}−\mathrm{b}\right)\mathrm{z}−\mathrm{1}\Rightarrow\mathrm{b}=−\mathrm{4} \\ $$$$\mathrm{4z}^{\mathrm{2}} −\mathrm{4z}+\mathrm{1}=\mathrm{0}\Rightarrow\mathrm{z}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\left(\mathrm{1}\right):\mathrm{z}=\mathrm{1} \\ $$$$\mathrm{x}+\mathrm{y}=\mathrm{1} \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{xy}=\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\Rightarrow\mathrm{x}=\frac{\mathrm{1}}{\mathrm{2}}\therefore\mathrm{y}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\left(\mathrm{2}\right):\mathrm{z}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{x}+\mathrm{y}=\frac{\mathrm{3}}{\mathrm{2}} \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} =\frac{\mathrm{5}}{\mathrm{4}} \\ $$$$\mathrm{xy}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{x}=\mathrm{1}\vee\mathrm{x}=\frac{\mathrm{1}}{\mathrm{2}}\therefore\mathrm{y}=\frac{\mathrm{1}}{\mathrm{2}}\vee\mathrm{y}=\mathrm{1} \\ $$$$\mathrm{so}\:\mathrm{all}\:\mathrm{solutions}\:\mathrm{are}: \\ $$$$\left(\mathrm{x},\:\mathrm{y},\:\mathrm{z}\right)=\left(\frac{\mathrm{1}}{\mathrm{2}},\:\frac{\mathrm{1}}{\mathrm{2}},\:\mathrm{1}\right),\:\left(\mathrm{1},\:\frac{\mathrm{1}}{\mathrm{2}},\:\frac{\mathrm{1}}{\mathrm{2}}\right),\:\left(\frac{\mathrm{1}}{\mathrm{2}},\:\mathrm{1},\:\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$
Commented by I want to learn more last updated on 23/Aug/20
Thanks sir
$$\mathrm{Thanks}\:\mathrm{sir} \\ $$
Answered by 1549442205PVT last updated on 23/Aug/20
{ ((x^2 +y^2 +z^2 =3/2)),((x+y+z=2)) :} ⇒xy+yz+zx=(((x+y+z)^2 −(x^2 +y^2 +z^2 ))/2) =((2^2 −3/2)/2)=(5/4) { ((x+y+z=2)),((xy+yz+zx=5/4)),((xyz=1/4)) :} From Vieta′s theorem we infer that x,y,z are the roots of the equation of degree 3: t^3 −2t^2 +(5/4)t−(1/4)=0 ⇔4t^3 −8t^2 +5t−1=0⇔(t−1)(4t^2 −4t+1)=0 ⇔(t−1)(2t−1)=0⇒t_(1,2,3) ∈{1,(1/2),(1/2)} ⇒(x,y,z)∈{(1,(1/2),(1/2)),((1/2),1,(1/2)),((1/2),(1/2),1)}
$$\begin{cases}{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} =\mathrm{3}/\mathrm{2}}\\{\mathrm{x}+\mathrm{y}+\mathrm{z}=\mathrm{2}}\end{cases} \\ $$$$\Rightarrow\mathrm{xy}+\mathrm{yz}+\mathrm{zx}=\frac{\left(\mathrm{x}+\mathrm{y}+\mathrm{z}\right)^{\mathrm{2}} −\left(\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} \right)}{\mathrm{2}} \\ $$$$=\frac{\mathrm{2}^{\mathrm{2}} −\mathrm{3}/\mathrm{2}}{\mathrm{2}}=\frac{\mathrm{5}}{\mathrm{4}} \\ $$$$\begin{cases}{\mathrm{x}+\mathrm{y}+\mathrm{z}=\mathrm{2}}\\{\mathrm{xy}+\mathrm{yz}+\mathrm{zx}=\mathrm{5}/\mathrm{4}}\\{\mathrm{xyz}=\mathrm{1}/\mathrm{4}}\end{cases} \\ $$$$\mathrm{From}\:\mathrm{Vieta}'\mathrm{s}\:\mathrm{theorem}\:\mathrm{we}\:\mathrm{infer}\:\mathrm{that} \\ $$$$\mathrm{x},\mathrm{y},\mathrm{z}\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\mathrm{of}\:\mathrm{degree}\:\mathrm{3}:\:\:\:\:\:\mathrm{t}^{\mathrm{3}} −\mathrm{2t}^{\mathrm{2}} +\frac{\mathrm{5}}{\mathrm{4}}\mathrm{t}−\frac{\mathrm{1}}{\mathrm{4}}=\mathrm{0} \\ $$$$\Leftrightarrow\mathrm{4t}^{\mathrm{3}} −\mathrm{8t}^{\mathrm{2}} +\mathrm{5t}−\mathrm{1}=\mathrm{0}\Leftrightarrow\left(\mathrm{t}−\mathrm{1}\right)\left(\mathrm{4t}^{\mathrm{2}} −\mathrm{4t}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\Leftrightarrow\left(\mathrm{t}−\mathrm{1}\right)\left(\mathrm{2t}−\mathrm{1}\right)=\mathrm{0}\Rightarrow\mathrm{t}_{\mathrm{1},\mathrm{2},\mathrm{3}} \in\left\{\mathrm{1},\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}}\right\} \\ $$$$\Rightarrow\left(\boldsymbol{\mathrm{x}},\boldsymbol{\mathrm{y}},\boldsymbol{\mathrm{z}}\right)\in\left\{\left(\mathrm{1},\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}}\right),\left(\frac{\mathrm{1}}{\mathrm{2}},\mathrm{1},\frac{\mathrm{1}}{\mathrm{2}}\right),\left(\frac{\mathrm{1}}{\mathrm{2}},\frac{\mathrm{1}}{\mathrm{2}},\mathrm{1}\right)\right\} \\ $$
Commented by I want to learn more last updated on 23/Aug/20
Thanks sir
$$\mathrm{Thanks}\:\mathrm{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *