Question-109377

Question Number 109377 by bemath last updated on 23/Aug/20

Answered by john santu last updated on 23/Aug/20

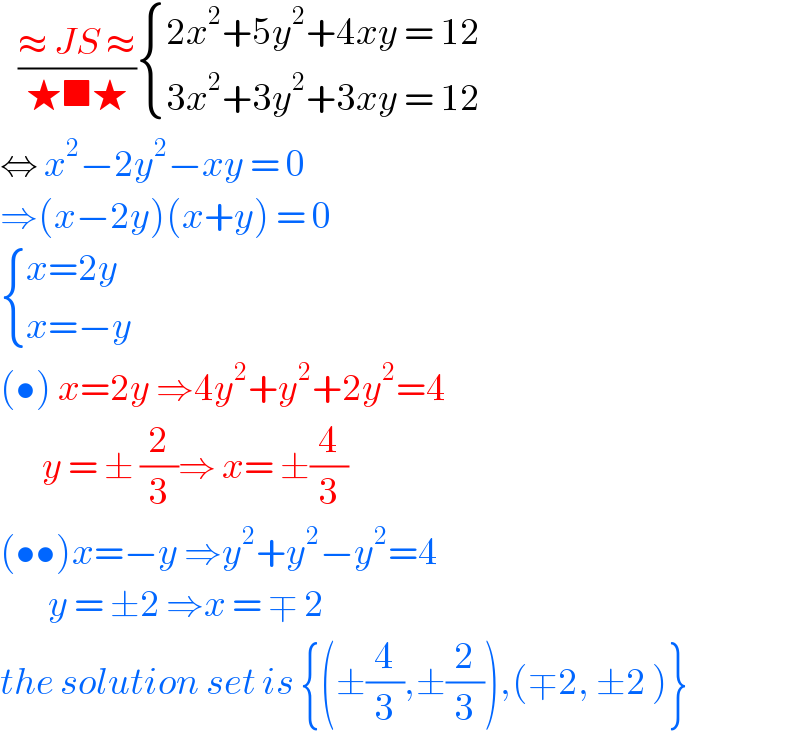
((≈ JS ≈)/(★■★)) { ((2x^2 +5y^2 +4xy = 12)),((3x^2 +3y^2 +3xy = 12)) :} ⇔ x^2 −2y^2 −xy = 0 ⇒(x−2y)(x+y) = 0 { ((x=2y)),((x=−y)) :} (•) x=2y ⇒4y^2 +y^2 +2y^2 =4 y = ± (2/3)⇒ x= ±(4/3) (••)x=−y ⇒y^2 +y^2 −y^2 =4 y = ±2 ⇒x = ∓ 2 the solution set is {(±(4/3),±(2/3)),(∓2, ±2 )}

$$\:\:\:\frac{\approx\:{JS}\:\approx}{\bigstar\blacksquare\bigstar}\begin{cases}{\mathrm{2}{x}^{\mathrm{2}} +\mathrm{5}{y}^{\mathrm{2}} +\mathrm{4}{xy}\:=\:\mathrm{12}}\\{\mathrm{3}{x}^{\mathrm{2}} +\mathrm{3}{y}^{\mathrm{2}} +\mathrm{3}{xy}\:=\:\mathrm{12}}\end{cases} \\ $$$$\Leftrightarrow\:{x}^{\mathrm{2}} −\mathrm{2}{y}^{\mathrm{2}} −{xy}\:=\:\mathrm{0} \\ $$$$\Rightarrow\left({x}−\mathrm{2}{y}\right)\left({x}+{y}\right)\:=\:\mathrm{0}\: \\ $$$$\begin{cases}{{x}=\mathrm{2}{y}}\\{{x}=−{y}}\end{cases} \\ $$$$\left(\bullet\right)\:{x}=\mathrm{2}{y}\:\Rightarrow\mathrm{4}{y}^{\mathrm{2}} +{y}^{\mathrm{2}} +\mathrm{2}{y}^{\mathrm{2}} =\mathrm{4}\: \\ $$$$\:\:\:\:\:\:\:{y}\:=\:\pm\:\frac{\mathrm{2}}{\mathrm{3}}\Rightarrow\:{x}=\:\pm\frac{\mathrm{4}}{\mathrm{3}} \\ $$$$\left(\bullet\bullet\right){x}=−{y}\:\Rightarrow{y}^{\mathrm{2}} +{y}^{\mathrm{2}} −{y}^{\mathrm{2}} =\mathrm{4} \\ $$$$\:\:\:\:\:\:\:\:{y}\:=\:\pm\mathrm{2}\:\Rightarrow{x}\:=\:\mp\:\mathrm{2}\: \\ $$$${the}\:{solution}\:{set}\:{is}\:\left\{\left(\pm\frac{\mathrm{4}}{\mathrm{3}},\pm\frac{\mathrm{2}}{\mathrm{3}}\right),\left(\mp\mathrm{2},\:\pm\mathrm{2}\:\right)\right\} \\ $$

Commented by bobhans last updated on 23/Aug/20