Question Number 112162 by 175mohamed last updated on 06/Sep/20

Commented by JDamian last updated on 06/Sep/20
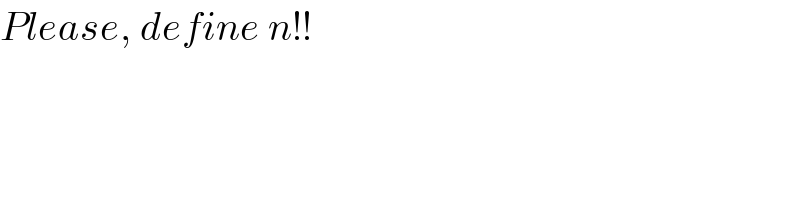
$${Please},\:{define}\:{n}!! \\ $$
Commented by mnjuly1970 last updated on 06/Sep/20

$${n}!!\:\left({double}\:{factorial}\right) \\ $$$${if}\:{n}=\mathrm{2}{k}\:\Rightarrow \\ $$$$\left(\mathrm{2}{k}\right)!!=\left(\mathrm{2}{k}−\mathrm{2}\right)\ast\left(\mathrm{2}{k}−\mathrm{4}\right)\ast\left(\mathrm{2}{k}−\mathrm{6}\right)….\ast\mathrm{2} \\ $$$${if}\:{n}=\mathrm{2}{k}−\mathrm{1}\:\Rightarrow \\ $$$$\left(\mathrm{2}{k}−\mathrm{1}\right)\ast\left(\mathrm{2}{k}−\mathrm{3}\right)\ast\left(\mathrm{2}{k}−\mathrm{5}\right)…..\ast\mathrm{3}\ast\mathrm{1} \\ $$$${for}\:\:{example} \\ $$$$\mathrm{8}!!\:=\mathrm{8}\ast\mathrm{6}\ast\mathrm{4}\ast\mathrm{2}\:=?? \\ $$$$\mathrm{9}!!\:=\mathrm{9}\ast\mathrm{7}\ast\mathrm{5}\ast\mathrm{3}\ast\mathrm{1} \\ $$
Commented by MJS_new last updated on 06/Sep/20
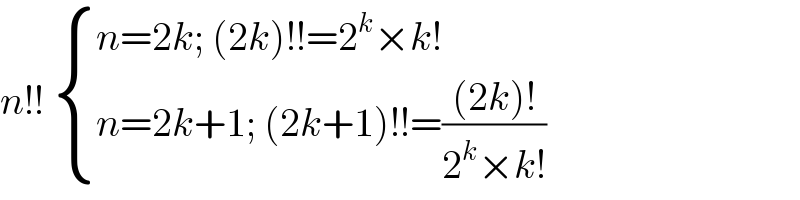
$${n}!!\:\begin{cases}{{n}=\mathrm{2}{k};\:\left(\mathrm{2}{k}\right)!!=\mathrm{2}^{{k}} ×{k}!}\\{{n}=\mathrm{2}{k}+\mathrm{1};\:\left(\mathrm{2}{k}+\mathrm{1}\right)!!=\frac{\left(\mathrm{2}{k}\right)!}{\mathrm{2}^{{k}} ×{k}!}}\end{cases} \\ $$
Answered by mnjuly1970 last updated on 06/Sep/20
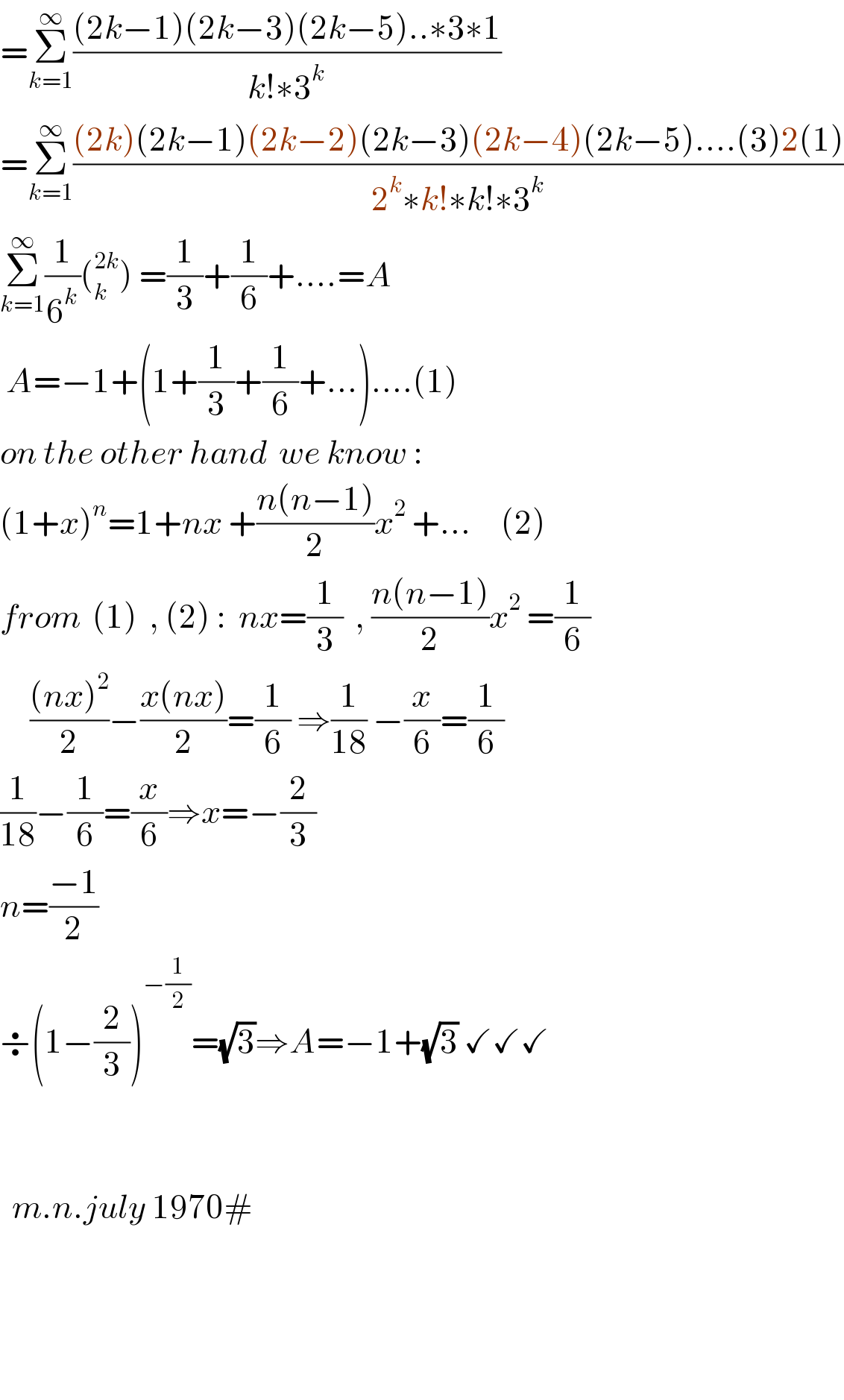
$$=\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{k}−\mathrm{1}\right)\left(\mathrm{2}{k}−\mathrm{3}\right)\left(\mathrm{2}{k}−\mathrm{5}\right)..\ast\mathrm{3}\ast\mathrm{1}}{{k}!\ast\mathrm{3}^{{k}} } \\ $$$$=\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}{k}\right)\left(\mathrm{2}{k}−\mathrm{1}\right)\left(\mathrm{2}{k}−\mathrm{2}\right)\left(\mathrm{2}{k}−\mathrm{3}\right)\left(\mathrm{2}{k}−\mathrm{4}\right)\left(\mathrm{2}{k}−\mathrm{5}\right)….\left(\mathrm{3}\right)\mathrm{2}\left(\mathrm{1}\right)}{\mathrm{2}^{{k}} \ast{k}!\ast{k}!\ast\mathrm{3}^{{k}} } \\ $$$$\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{6}^{{k}} }\left(_{{k}} ^{\mathrm{2}{k}} \right)\:=\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{6}}+….={A} \\ $$$$\:{A}=−\mathrm{1}+\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{6}}+…\right)….\left(\mathrm{1}\right) \\ $$$${on}\:{the}\:{other}\:{hand}\:\:{we}\:{know}\:: \\ $$$$\left(\mathrm{1}+{x}\right)^{{n}} =\mathrm{1}+{nx}\:+\frac{{n}\left({n}−\mathrm{1}\right)}{\mathrm{2}}{x}^{\mathrm{2}} \:+…\:\:\:\:\:\left(\mathrm{2}\right) \\ $$$${from}\:\:\left(\mathrm{1}\right)\:\:,\:\left(\mathrm{2}\right)\::\:\:{nx}=\frac{\mathrm{1}}{\mathrm{3}}\:\:,\:\frac{{n}\left({n}−\mathrm{1}\right)}{\mathrm{2}}{x}^{\mathrm{2}} \:=\frac{\mathrm{1}}{\mathrm{6}} \\ $$$$\:\:\:\:\:\frac{\left({nx}\right)^{\mathrm{2}} }{\mathrm{2}}−\frac{{x}\left({nx}\right)}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{6}}\:\Rightarrow\frac{\mathrm{1}}{\mathrm{18}}\:−\frac{{x}}{\mathrm{6}}=\frac{\mathrm{1}}{\mathrm{6}} \\ $$$$\frac{\mathrm{1}}{\mathrm{18}}−\frac{\mathrm{1}}{\mathrm{6}}=\frac{{x}}{\mathrm{6}}\Rightarrow{x}=−\frac{\mathrm{2}}{\mathrm{3}} \\ $$$${n}=\frac{−\mathrm{1}}{\mathrm{2}} \\ $$$$\boldsymbol{\div}\left(\mathrm{1}−\frac{\mathrm{2}}{\mathrm{3}}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} =\sqrt{\mathrm{3}}\Rightarrow{A}=−\mathrm{1}+\sqrt{\mathrm{3}}\:\checkmark\checkmark\checkmark \\ $$$$ \\ $$$$ \\ $$$$\:\:{m}.{n}.{july}\:\mathrm{1970}# \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by mohammad17 last updated on 07/Sep/20
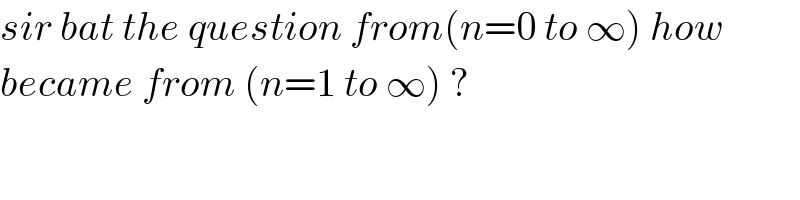
$${sir}\:{bat}\:{the}\:{question}\:{from}\left({n}=\mathrm{0}\:{to}\:\infty\right)\:{how} \\ $$$${became}\:{from}\:\left({n}=\mathrm{1}\:{to}\:\infty\right)\:? \\ $$
Commented by mnjuly1970 last updated on 07/Sep/20

$$\left(−\mathrm{1}\right)!!\:=!!! \\ $$$$ \\ $$
Commented by MJS_new last updated on 07/Sep/20
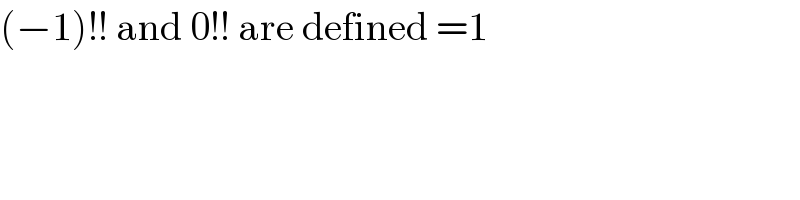
$$\left(−\mathrm{1}\right)!!\:\mathrm{and}\:\mathrm{0}!!\:\mathrm{are}\:\mathrm{defined}\:=\mathrm{1} \\ $$
Commented by mohammad17 last updated on 07/Sep/20

$${thank}\:{you}\:{sir} \\ $$
Commented by mohammad17 last updated on 07/Sep/20

$${thank}\:{you}\:{sir} \\ $$
