Question Number 112881 by bemath last updated on 10/Sep/20
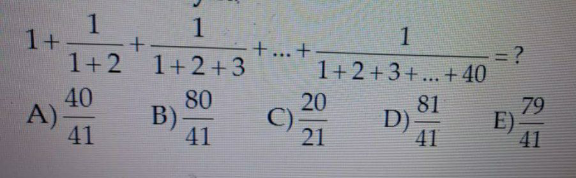
Answered by Dwaipayan Shikari last updated on 10/Sep/20
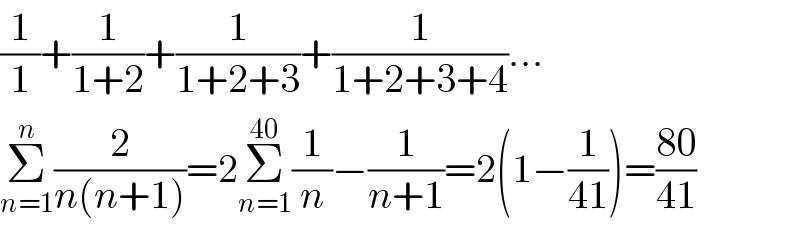
$$\frac{\mathrm{1}}{\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}+\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}+\mathrm{3}+\mathrm{4}}… \\ $$$$\underset{{n}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{2}}{{n}\left({n}+\mathrm{1}\right)}=\mathrm{2}\underset{{n}=\mathrm{1}} {\overset{\mathrm{40}} {\sum}}\frac{\mathrm{1}}{{n}}−\frac{\mathrm{1}}{{n}+\mathrm{1}}=\mathrm{2}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{41}}\right)=\frac{\mathrm{80}}{\mathrm{41}} \\ $$
Commented by bemath last updated on 10/Sep/20

$$\mathrm{sir}\:\mathrm{how}\:\mathrm{you}\:\mathrm{to}\:\mathrm{get}\:\mathrm{the}\:\mathrm{formula}\:\frac{\mathrm{2}}{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)} \\ $$
Commented by Dwaipayan Shikari last updated on 10/Sep/20
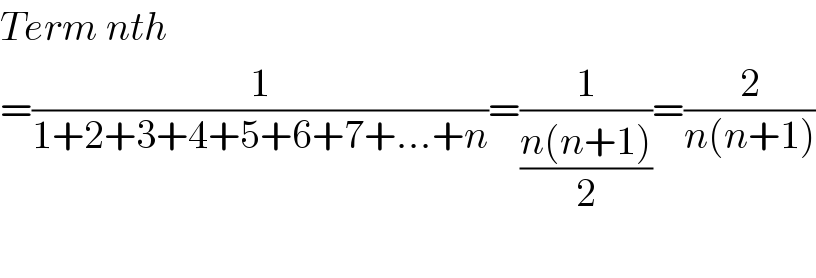
$${Term}\:{nth} \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2}+\mathrm{3}+\mathrm{4}+\mathrm{5}+\mathrm{6}+\mathrm{7}+…+{n}}=\frac{\mathrm{1}}{\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}}=\frac{\mathrm{2}}{{n}\left({n}+\mathrm{1}\right)} \\ $$$$ \\ $$
Commented by bemath last updated on 10/Sep/20

$$\mathrm{oo}\:\mathrm{thank}\:\mathrm{you} \\ $$
