Question Number 112882 by bemath last updated on 10/Sep/20

Commented by bemath last updated on 10/Sep/20
$$\mathrm{prof}\:\mathrm{MJs},\mathrm{prof}\:\mathrm{Mr}\:\mathrm{Abdo}\:\mathrm{and}\:\mathrm{other} \\ $$$$\mathrm{can}\:\mathrm{you}\:\mathrm{help}\:\mathrm{me}? \\ $$
Commented by mathmax by abdo last updated on 10/Sep/20

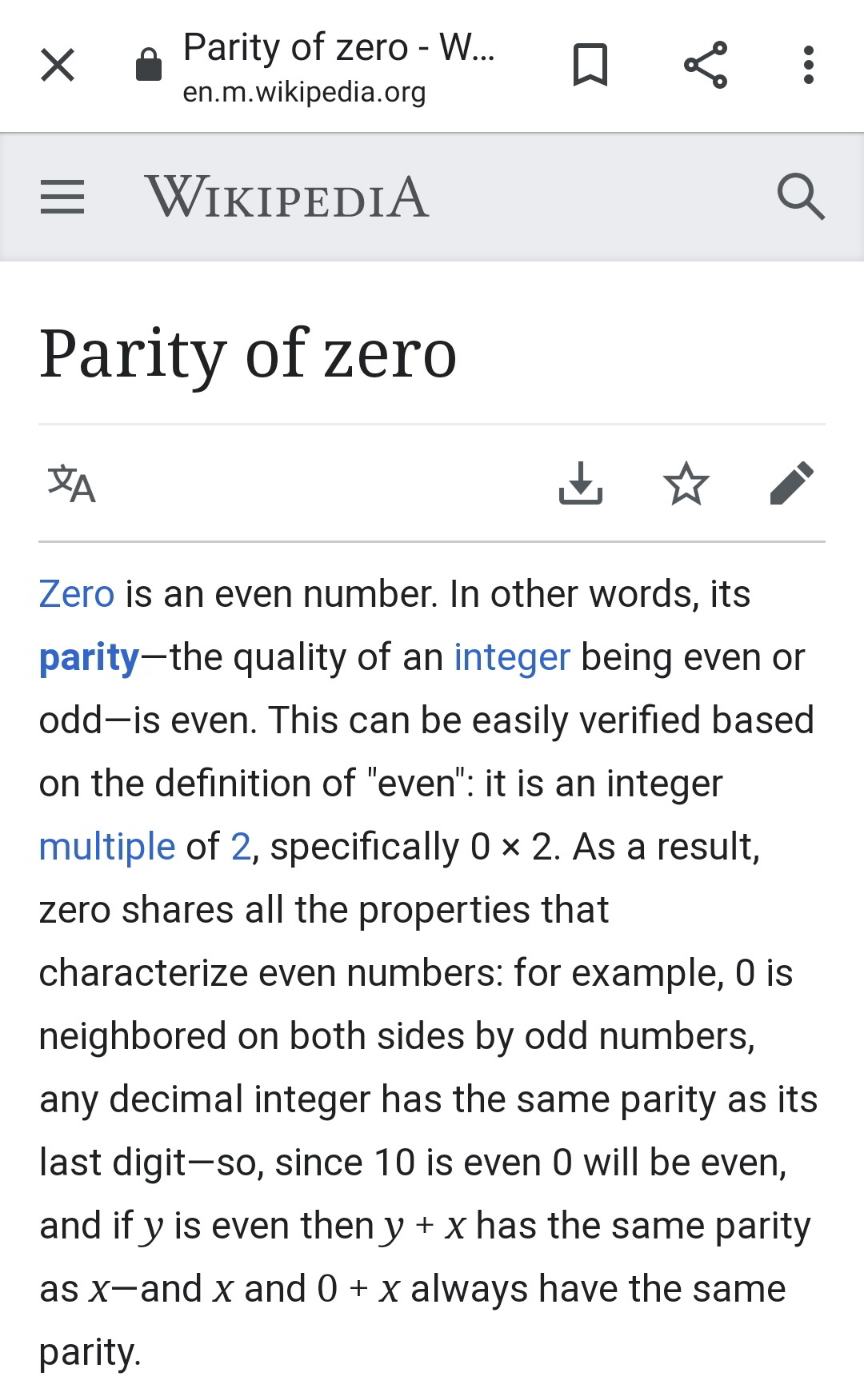
$$\mathrm{all}\:\mathrm{i}\:\mathrm{know}\:\:\mathrm{and}\:\:\mathrm{all}\:\mathrm{i}\:\mathrm{have}\:\mathrm{in}\:\mathrm{my}\:\mathrm{storeis}\:\mathrm{that}\:\mathrm{0}\:\mathrm{is}\:\mathrm{a}\:\mathrm{even}\:\mathrm{number}. \\ $$$$\left(\mathrm{at}\:\mathrm{form}\:\mathrm{2K}\right) \\ $$
Answered by mr W last updated on 10/Sep/20
Commented by bemath last updated on 10/Sep/20
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{mr}\:\mathrm{W}.\:\mathrm{maybe}\:\mathrm{you}\:\mathrm{have} \\ $$$$\mathrm{pdf}\:\mathrm{file}\:\mathrm{or}\:\mathrm{book}? \\ $$
Commented by bemath last updated on 10/Sep/20
i agree with all sir that zero is an even number, but my colleagues ask if there is any book explaining that. they say zero is also divisible by 3. they say zero is not a number and not an odd number. it is an independent number.
Commented by MJS_new last updated on 10/Sep/20

$$\mathrm{6}\:\mathrm{is}\:\mathrm{also}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{2}\:\mathrm{and}\:\mathrm{3} \\ $$$$\mathrm{if}\:\mathrm{0}\:\mathrm{is}\:“\mathrm{independent}'',\:\mathrm{what}\:\mathrm{makes}\:\mathrm{it}\:\mathrm{independent}, \\ $$$$\mathrm{as}\:\mathrm{it}\:\mathrm{behaves}\:\mathrm{exactly}\:\mathrm{like}\:\mathrm{all}\:\mathrm{the}\:\mathrm{even}\:\mathrm{numbers}? \\ $$$$ \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{2}\:\mathrm{even}\:\mathrm{numbers}\:\mathrm{is}\:\mathrm{even} \\ $$$$\mathrm{0}+\mathrm{24}=\mathrm{24} \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{2}\:\mathrm{odd}\:\mathrm{numbers}\:\mathrm{is}\:\mathrm{even} \\ $$$$−\mathrm{5}+\mathrm{5}=\mathrm{0} \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{1}\:\mathrm{odd}\:\mathrm{and}\:\mathrm{1}\:\mathrm{even}\:\mathrm{number}\:\mathrm{is}\:\mathrm{odd} \\ $$$$\mathrm{0}+\mathrm{3}=\mathrm{3} \\ $$$$ \\ $$$$\mathrm{you}\:\mathrm{would}\:\mathrm{have}\:\mathrm{to}\:\mathrm{say}\:“\mathrm{0}\:\mathrm{is}\:\mathrm{independent}\:\mathrm{as} \\ $$$$\mathrm{long}\:\mathrm{it}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{interact}''. \\ $$
Answered by MJS_new last updated on 10/Sep/20

$$\mathrm{a}\:\mathrm{number}\:{z}\in\mathbb{Z}\:\mathrm{is}\:\mathrm{called}\:“\mathrm{even}''\:\Leftrightarrow\:\mathrm{the} \\ $$$$\mathrm{remainder}\:\mathrm{of}\:\mathrm{its}\:\mathrm{division}\:\mathrm{by}\:\mathrm{2}\:\mathrm{is}\:\mathrm{0} \\ $$$$\mathrm{this}\:\mathrm{is}\:\mathrm{a}\:\boldsymbol{{definition}}.\:\mathrm{a}\:\mathrm{definition}\:\mathrm{cannot}\:\mathrm{be} \\ $$$$\mathrm{right}\:\mathrm{or}\:\mathrm{wrong}. \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{only}\:\mathrm{test}\:\mathrm{a}\:\mathrm{hypothesis}: \\ $$$$ \\ $$$$\mathrm{assume}\:\mathrm{17}\:\mathrm{is}\:\mathrm{even} \\ $$$$\mathrm{17}:\mathrm{2}=\mathrm{8}\:\mathrm{remainder}\:\mathrm{1} \\ $$$$\mathrm{but}\:\mathrm{1}\neq\mathrm{0}\:\Rightarrow\:\mathrm{17}\:\mathrm{is}\:\mathrm{not}\:\mathrm{even} \\ $$$$ \\ $$$$\mathrm{assume}\:\mathrm{0}\:\mathrm{is}\:\mathrm{even} \\ $$$$\mathrm{0}:\mathrm{2}=\mathrm{0}\:\mathrm{remainder}\:\mathrm{0}\:\Rightarrow\:\mathrm{0}\:\mathrm{is}\:\mathrm{even} \\ $$
Commented by bemath last updated on 10/Sep/20
![sir. So this a matter that is our debate. : A child will make a password in the form of [letters_1 ],[ number_1 ], [letters_2 ] , [ number_2 ] with condition (1) the first letter is a vowel except I (2) the first number is even (3) the second letter is O (4) the second number are selected from the set { 1,2,4,6,8 }. How many passwords can be generated? my answer is = 4×5×1×5 = 100](https://www.tinkutara.com/question/Q112951.png)
$$\mathrm{sir}.\:\mathrm{So}\:\mathrm{this}\:\mathrm{a}\:\mathrm{matter}\:\mathrm{that}\:\mathrm{is}\:\mathrm{our}\:\mathrm{debate}. \\ $$$$:\:\mathrm{A}\:\mathrm{child}\:\mathrm{will}\:\mathrm{make}\:\mathrm{a}\:\mathrm{password}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{form}\:\mathrm{of}\:\left[\mathrm{letters}_{\mathrm{1}} \:\right],\left[\:\mathrm{number}_{\mathrm{1}} \right], \\ $$$$\left[\mathrm{letters}_{\mathrm{2}} \right]\:,\:\left[\:\mathrm{number}_{\mathrm{2}} \right]\:\mathrm{with}\:\mathrm{condition} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{the}\:\mathrm{first}\:\mathrm{letter}\:\mathrm{is}\:\mathrm{a}\:\mathrm{vowel}\:\mathrm{except}\:\mathrm{I} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{the}\:\mathrm{first}\:\mathrm{number}\:\mathrm{is}\:\mathrm{even} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{the}\:\mathrm{second}\:\mathrm{letter}\:\mathrm{is}\:\mathrm{O} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{the}\:\mathrm{second}\:\mathrm{number}\:\mathrm{are}\:\mathrm{selected}\:\mathrm{from}\:\mathrm{the}\: \\ $$$$\mathrm{set}\:\left\{\:\mathrm{1},\mathrm{2},\mathrm{4},\mathrm{6},\mathrm{8}\:\right\}. \\ $$$$\mathrm{How}\:\mathrm{many}\:\mathrm{passwords}\:\mathrm{can}\:\mathrm{be}\: \\ $$$$\mathrm{generated}? \\ $$$$\mathrm{my}\:\mathrm{answer}\:\mathrm{is}\:=\:\mathrm{4}×\mathrm{5}×\mathrm{1}×\mathrm{5}\:=\:\mathrm{100} \\ $$
Commented by bemath last updated on 10/Sep/20
$$\mathrm{but}\:\mathrm{my}\:\mathrm{friend}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{80} \\ $$
Commented by MJS_new last updated on 10/Sep/20

$$\mathrm{your}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{right}\:\mathrm{because}\:\mathrm{0}\:\mathrm{is}\:\mathrm{even}. \\ $$$$\mathrm{try}\:\mathrm{to}\:\mathrm{prove}\:\mathrm{it}\:\mathrm{is}\:\mathrm{not}\:\mathrm{even}…\:\mathrm{if}\:\mathrm{somebody}\:\mathrm{has} \\ $$$$\mathrm{a}\:\mathrm{proof},\:\mathrm{let}\:\mathrm{me}\:\mathrm{know},\:\mathrm{I}'\mathrm{ll}\:\mathrm{review}\:\mathrm{it} \\ $$
Commented by bemath last updated on 10/Sep/20
that's how my colleagues insist that zero is not an even number. my emotions were bought. they argue which book explains that it is an even number
Answered by $@y@m last updated on 10/Sep/20
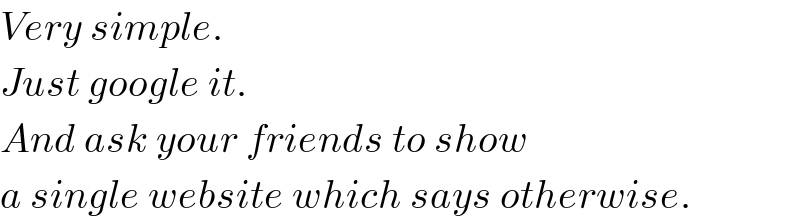
$${Very}\:{simple}. \\ $$$${Just}\:{google}\:{it}. \\ $$$${And}\:{ask}\:{your}\:{friends}\:{to}\:{show} \\ $$$${a}\:{single}\:{website}\:{which}\:{says}\:{otherwise}. \\ $$
Commented by mathmax by abdo last updated on 11/Sep/20

$$\mathrm{its}\:\mathrm{seems}\:\mathrm{that}\:\mathrm{you}\:\mathrm{are}\:\mathrm{open}\:\mathrm{a}\:\mathrm{door}\:\mathrm{of}\:\mathrm{philosofy}…. \\ $$
Commented by $@y@m last updated on 11/Sep/20
������
The question itself is philosophy.
Mr. Bemath knows 0 is an even number.
The problem is how to convince his friend.
Commented by MJS_new last updated on 11/Sep/20

$$\mathrm{The}\:\mathrm{problem}\:\mathrm{is},\:\mathrm{you}\:\mathrm{cannot}\:\mathrm{convince}\:\mathrm{anybody} \\ $$$$\mathrm{who}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{want}\:\mathrm{to}\:\mathrm{follow}\:\mathrm{your}\:\mathrm{arguments}. \\ $$$$\mathrm{I}\:\mathrm{remember}\:\mathrm{a}\:\mathrm{person}\:\mathrm{here}\:\mathrm{who}\:“\mathrm{proved}''\:\mathrm{that} \\ $$$$\mathrm{i}=\sqrt{−\mathrm{1}}=\pm\mathrm{1},\:\mathrm{other}\:\mathrm{people}\:\mathrm{believe}\:\pi\:\mathrm{is}\:\mathrm{wrong} \\ $$$$\mathrm{because}\:\mathrm{its}\:“\mathrm{true}''\:\mathrm{value}\:\mathrm{is}\:\mathrm{6}.\mathrm{283185}…\:\mathrm{not} \\ $$$$\mathrm{3}.\mathrm{141592}…\:\mathrm{I}\:\mathrm{also}\:\mathrm{remember}\:\mathrm{some}\:\mathrm{fights}\:\mathrm{with} \\ $$$$\mathrm{someone}\:\mathrm{who}\:\mathrm{believes}\:\mathrm{5}−\mathrm{2}×\mathrm{3}=\mathrm{9}. \\ $$
Commented by MJS_new last updated on 11/Sep/20

$$\boldsymbol{\mathrm{definition}}:\:\forall\boldsymbol{{n}}\in\mathbb{Z}:\:\boldsymbol{\mathrm{if}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{division}}\:\boldsymbol{{n}}:\mathrm{2}\:\boldsymbol{\mathrm{leaves}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{reminder}}\:\mathrm{0}\:\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{call}}\:\boldsymbol{{n}}\:\boldsymbol{\mathrm{even}} \\ $$$$\mathrm{this}\:\mathrm{is}\:\mathrm{all}\:\mathrm{we}\:\mathrm{need}. \\ $$$${n}\:\mathrm{can}\:\mathrm{be}\:\begin{cases}{\mathrm{even}}\\{\mathrm{not}\:\mathrm{even}\:=\:\mathrm{uneven}\:=\mathrm{odd}}\\{\mathrm{undecideable}}\end{cases} \\ $$$$\mathrm{examples}: \\ $$$$\mathrm{even}:\:−\mathrm{16};\:\mathrm{542};\:\mathrm{6}{u}+\mathrm{2}\forall{u}\in\mathbb{Z};\:\mathrm{2}{p}^{\mathrm{2}} −\mathrm{18}\forall{p}\in\mathbb{Z} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{generally}\:\mathrm{2}{k}\forall{k}\in\mathbb{Z} \\ $$$$\mathrm{odd}:\:\:\:−\mathrm{371};\:\mathrm{3};\:\mathrm{8}{h}−\mathrm{1}\forall{h}\in\mathbb{Z};\:\mathrm{2}{s}^{\mathrm{2}} −\mathrm{4}{t}^{\mathrm{4}} +\mathrm{1}\forall{s},\:{t}\:\in\mathbb{Z} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{generally}\:\mathrm{2}{k}+\mathrm{1}\forall{k}\in\mathbb{Z} \\ $$$$\mathrm{undecideable}:\:{e}\in\mathbb{Z};\:{u}×{v}\wedge{u},\:{v}\:\in\mathbb{Z},\:{h}^{\mathrm{3}} +\mathrm{5}\wedge{h}\in\mathbb{Z} \\ $$$$ \\ $$$$\mathrm{thesis}:\:\mathrm{0}\:\mathrm{is}\:\mathrm{odd} \\ $$$$\mathrm{prove}:\:\mathrm{0}\in\mathbb{Z}\:\Rightarrow\:\mathrm{0}\:\mathrm{is}\:\mathrm{odd}\:\Leftrightarrow\:\exists{k}\in\mathbb{Z}:\:\mathrm{2}{k}+\mathrm{1}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}{k}+\mathrm{1}=\mathrm{0}\:\:\:\:\:\mid−\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}{k}=−\mathrm{1}\:\:\:\:\:\:\:\mid\boldsymbol{\div}\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{k}=−\frac{\mathrm{1}}{\mathrm{2}}\:\notin\mathbb{Z}\:\Rightarrow\:\mathrm{false}\:\Rightarrow\:\mathrm{0}\:\mathrm{is}\:\mathrm{not}\:\mathrm{odd} \\ $$$$\mathrm{thesis}:\:\mathrm{0}\:\mathrm{is}\:\mathrm{even} \\ $$$$\mathrm{prove}:\:\mathrm{0}\in\mathbb{Z}\:\Rightarrow\:\mathrm{0}\:\mathrm{is}\:\mathrm{even}\:\Leftrightarrow\:\exists{k}\in\mathbb{Z}:\mathrm{2}{k}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}{k}=\mathrm{0}\:\:\:\:\:\:\mid\boldsymbol{\div}\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{k}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{testing}:\:\mathrm{2}{k}=\mathrm{2}×\mathrm{0}=\mathrm{0}\:\Rightarrow\:\mathrm{0}\:\mathrm{is}\:\mathrm{even} \\ $$$$ \\ $$$$\boldsymbol{\mathrm{note}}:\:\boldsymbol{\mathrm{if}}\:\boldsymbol{{n}}\notin\mathbb{Z}\:\boldsymbol{\mathrm{we}}\:\boldsymbol{\mathrm{might}}\:\boldsymbol{\mathrm{need}}\:\boldsymbol{\mathrm{additional}}\:\boldsymbol{\mathrm{definitions}} \\ $$$$\left(\frac{{p}}{{q}}\:\mathrm{divided}\:\mathrm{by}\:\mathrm{2}\:=\:\frac{{p}}{\mathrm{2}{q}}\:\mathrm{remainder}\:\mathrm{0}\:\overset{???} {\Rightarrow}\:\frac{{p}}{{q}}\in\mathbb{Q}\:\mathrm{even}\right) \\ $$
Commented by bemath last updated on 11/Sep/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{prof} \\ $$
Commented by abdomsup last updated on 11/Sep/20

$${simple}\:{he}\:{must}\:{give}\:{them}\:{a}\:{pizza} \\ $$$${if}\:\:{they}\:{are}\:{two}\:{he}\:{divide}\:{the}\: \\ $$$${pizza}\:{by}\:\mathrm{2}\:\:{and}\:{him}\:{dont}\:{eat} \\ $$$${of}\:{they}\:{are}\:{tre}\:\:{he}\:{divide}\:{the} \\ $$$${pizza}\:{by}\:\mathrm{4}\:{and}\:{eat}\:{with}\:{them}…. \\ $$
