Question Number 113598 by bobhans last updated on 14/Sep/20
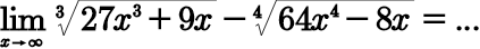
Answered by bemath last updated on 14/Sep/20
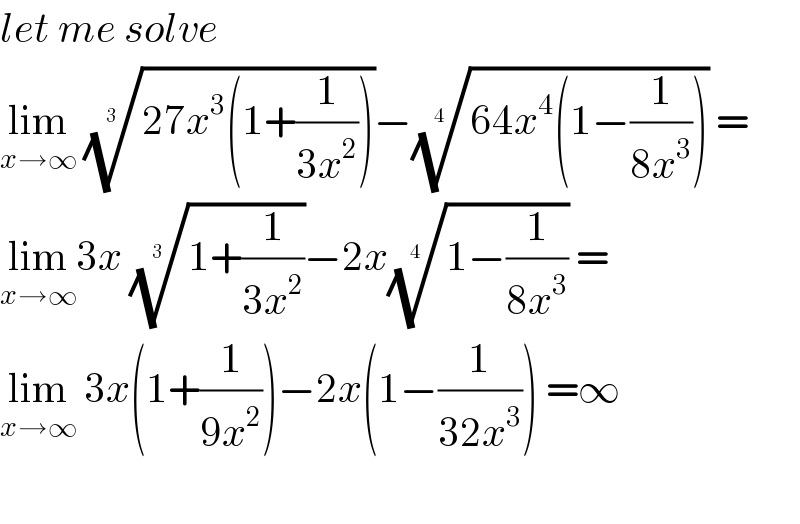
$${let}\:{me}\:{solve} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\sqrt[{\mathrm{3}\:}]{\mathrm{27}{x}^{\mathrm{3}} \left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}{x}^{\mathrm{2}} }\right)}−\sqrt[{\mathrm{4}\:}]{\mathrm{64}{x}^{\mathrm{4}} \left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{8}{x}^{\mathrm{3}} }\right)}\:= \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}3}{x}\:\sqrt[{\mathrm{3}\:}]{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}{x}^{\mathrm{2}} }}−\mathrm{2}{x}\sqrt[{\mathrm{4}\:}]{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{8}{x}^{\mathrm{3}} }}\:= \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\mathrm{3}{x}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{9}{x}^{\mathrm{2}} }\right)−\mathrm{2}{x}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{32}{x}^{\mathrm{3}} }\right)\:=\infty \\ $$$$ \\ $$
