Question Number 114796 by Algoritm last updated on 21/Sep/20

Answered by MJS_new last updated on 21/Sep/20
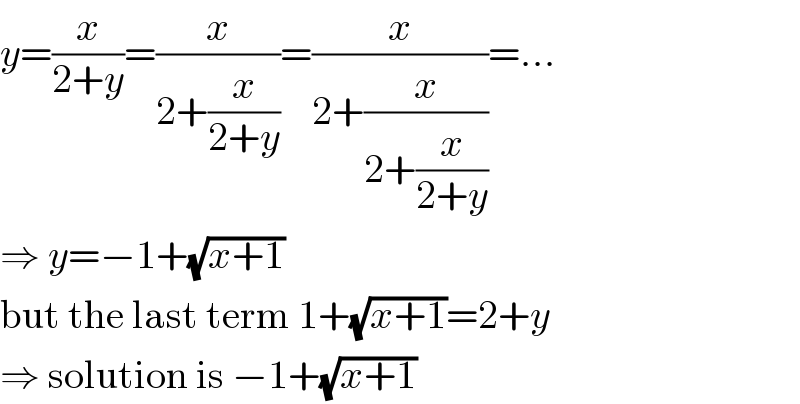
$${y}=\frac{{x}}{\mathrm{2}+{y}}=\frac{{x}}{\mathrm{2}+\frac{{x}}{\mathrm{2}+{y}}}=\frac{{x}}{\mathrm{2}+\frac{{x}}{\mathrm{2}+\frac{{x}}{\mathrm{2}+{y}}}}=… \\ $$$$\Rightarrow\:{y}=−\mathrm{1}+\sqrt{{x}+\mathrm{1}} \\ $$$$\mathrm{but}\:\mathrm{the}\:\mathrm{last}\:\mathrm{term}\:\mathrm{1}+\sqrt{{x}+\mathrm{1}}=\mathrm{2}+{y} \\ $$$$\Rightarrow\:\mathrm{solution}\:\mathrm{is}\:−\mathrm{1}+\sqrt{{x}+\mathrm{1}} \\ $$
Answered by PRITHWISH SEN 2 last updated on 21/Sep/20
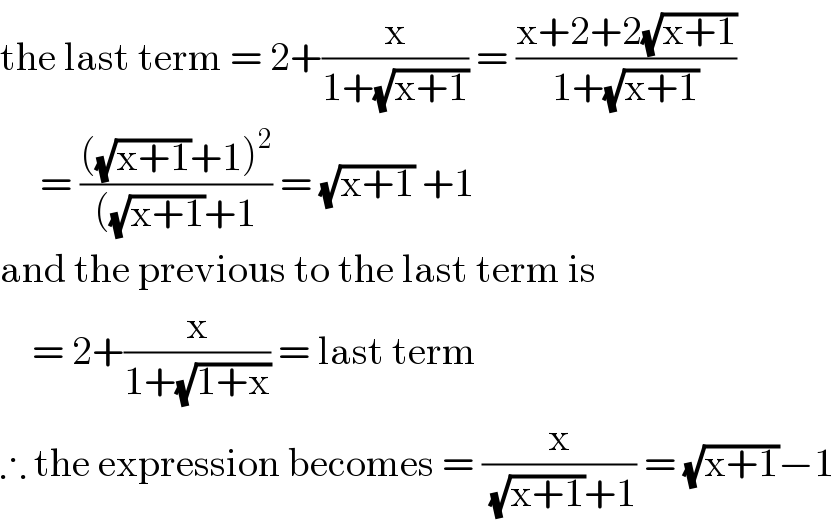
$$\mathrm{the}\:\mathrm{last}\:\mathrm{term}\:=\:\mathrm{2}+\frac{\mathrm{x}}{\mathrm{1}+\sqrt{\mathrm{x}+\mathrm{1}}}\:=\:\frac{\mathrm{x}+\mathrm{2}+\mathrm{2}\sqrt{\mathrm{x}+\mathrm{1}}}{\mathrm{1}+\sqrt{\mathrm{x}+\mathrm{1}}} \\ $$$$\:\:\:\:\:=\:\frac{\left(\sqrt{\mathrm{x}+\mathrm{1}}+\mathrm{1}\right)^{\mathrm{2}} }{\left(\sqrt{\mathrm{x}+\mathrm{1}}+\mathrm{1}\right.}\:=\:\sqrt{\mathrm{x}+\mathrm{1}}\:+\mathrm{1} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{previous}\:\mathrm{to}\:\mathrm{the}\:\mathrm{last}\:\mathrm{term}\:\mathrm{is} \\ $$$$\:\:\:\:=\:\mathrm{2}+\frac{\mathrm{x}}{\mathrm{1}+\sqrt{\mathrm{1}+\mathrm{x}}}\:=\:\mathrm{last}\:\mathrm{term} \\ $$$$\therefore\:\mathrm{the}\:\mathrm{expression}\:\mathrm{becomes}\:=\:\frac{\mathrm{x}}{\:\sqrt{\mathrm{x}+\mathrm{1}}+\mathrm{1}}\:=\:\sqrt{\mathrm{x}+\mathrm{1}}−\mathrm{1} \\ $$
