Question Number 115736 by gab last updated on 28/Sep/20

Answered by Dwaipayan Shikari last updated on 28/Sep/20
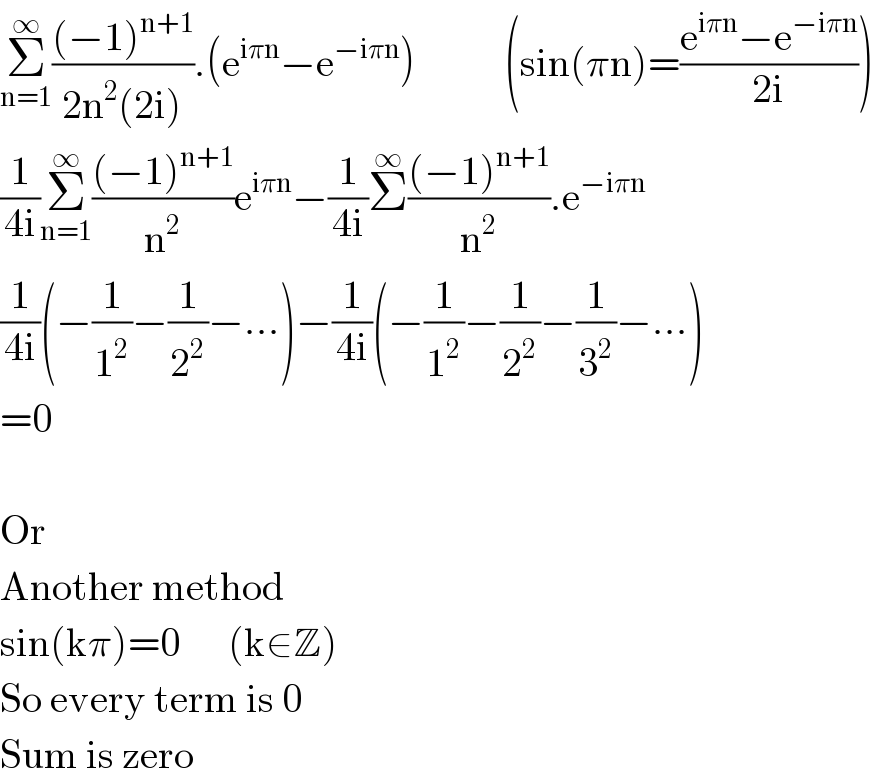
$$\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }{\mathrm{2n}^{\mathrm{2}} \left(\mathrm{2i}\right)}.\left(\mathrm{e}^{\mathrm{i}\pi\mathrm{n}} −\mathrm{e}^{−\mathrm{i}\pi\mathrm{n}} \right)\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{sin}\left(\pi\mathrm{n}\right)=\frac{\mathrm{e}^{\mathrm{i}\pi\mathrm{n}} −\mathrm{e}^{−\mathrm{i}\pi\mathrm{n}} }{\mathrm{2i}}\right) \\ $$$$\frac{\mathrm{1}}{\mathrm{4i}}\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}^{\mathrm{2}} }\mathrm{e}^{\mathrm{i}\pi\mathrm{n}} −\frac{\mathrm{1}}{\mathrm{4i}}\overset{\infty} {\sum}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}^{\mathrm{2}} }.\mathrm{e}^{−\mathrm{i}\pi\mathrm{n}} \\ $$$$\frac{\mathrm{1}}{\mathrm{4i}}\left(−\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }−…\right)−\frac{\mathrm{1}}{\mathrm{4i}}\left(−\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }−…\right) \\ $$$$=\mathrm{0} \\ $$$$ \\ $$$$\mathrm{Or} \\ $$$$\mathrm{Another}\:\mathrm{method}\: \\ $$$$\mathrm{sin}\left(\mathrm{k}\pi\right)=\mathrm{0}\:\:\:\:\:\:\left(\mathrm{k}\in\mathbb{Z}\right) \\ $$$$\mathrm{So}\:\mathrm{every}\:\mathrm{term}\:\mathrm{is}\:\mathrm{0} \\ $$$$\mathrm{Sum}\:\mathrm{is}\:\mathrm{zero} \\ $$
Commented by gab last updated on 28/Sep/20

$$ \\ $$$${thank}\:{you}\:{sir}. \\ $$
Answered by mathmax by abdo last updated on 28/Sep/20

$$\mathrm{sin}\left(\mathrm{n}\pi\right)=\mathrm{0}\:\Rightarrow\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}+\mathrm{1}} \mathrm{sin}\left(\mathrm{n}\pi\right)}{\mathrm{2n}^{\mathrm{2}} }=\mathrm{0} \\ $$
