Question Number 115798 by aye48 last updated on 28/Sep/20

Commented by bemath last updated on 29/Sep/20
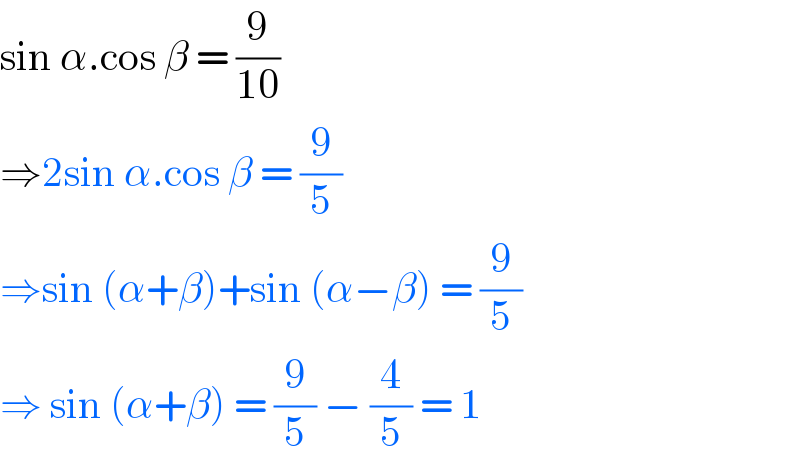
$$\mathrm{sin}\:\alpha.\mathrm{cos}\:\beta\:=\:\frac{\mathrm{9}}{\mathrm{10}} \\ $$$$\Rightarrow\mathrm{2sin}\:\alpha.\mathrm{cos}\:\beta\:=\:\frac{\mathrm{9}}{\mathrm{5}} \\ $$$$\Rightarrow\mathrm{sin}\:\left(\alpha+\beta\right)+\mathrm{sin}\:\left(\alpha−\beta\right)\:=\:\frac{\mathrm{9}}{\mathrm{5}} \\ $$$$\Rightarrow\:\mathrm{sin}\:\left(\alpha+\beta\right)\:=\:\frac{\mathrm{9}}{\mathrm{5}}\:−\:\frac{\mathrm{4}}{\mathrm{5}}\:=\:\mathrm{1} \\ $$
Answered by TANMAY PANACEA last updated on 29/Sep/20

$${sin}\alpha{cos}\beta−{cos}\alpha{sin}\beta=\frac{\mathrm{4}}{\mathrm{5}} \\ $$$${cos}\alpha{sin}\beta=\frac{\mathrm{9}}{\mathrm{10}}−\frac{\mathrm{4}}{\mathrm{5}}=\frac{\mathrm{1}}{\mathrm{10}} \\ $$$${sin}\left(\alpha−\beta\right)=\frac{\mathrm{4}}{\mathrm{5}}={sin}\mathrm{53}^{{o}} \\ $$$${sin}\left(\alpha+\beta\right)={sin}\alpha{cos}\beta+{cos}\alpha{sin}\beta=\frac{\mathrm{9}}{\mathrm{10}}+\frac{\mathrm{1}}{\mathrm{10}}={sin}\mathrm{90}^{{o}} =\mathrm{1} \\ $$$$\alpha+\beta=\mathrm{90} \\ $$$$\alpha−\beta=\mathrm{53} \\ $$$$\alpha=\frac{\mathrm{90}+\mathrm{53}}{\mathrm{2}}=\frac{\mathrm{143}}{\mathrm{2}} \\ $$$$\beta=\frac{\mathrm{90}−\mathrm{53}}{\mathrm{2}}=\frac{\mathrm{37}}{\mathrm{2}} \\ $$$$\frac{{tan}\alpha}{{tan}\beta}=\frac{{sin}\alpha{cos}\beta}{{sin}\beta{cos}\alpha}=\frac{\mathrm{9}}{\mathrm{10}}×\frac{\mathrm{10}}{\mathrm{1}}=\mathrm{9} \\ $$
