Question Number 115916 by I want to learn more last updated on 29/Sep/20

Answered by mr W last updated on 30/Sep/20

$${say}\:{the}\:{first}\:{shelf}\:{has}\:{k}\:{books}, \\ $$$${then}\:{the}\:{second}\:{shelf}\:{has}\:\mathrm{15}−{k}\:{books}. \\ $$$$\mathrm{1}\leqslant{k}\leqslant\mathrm{14} \\ $$$${to}\:{select}\:{k}\:{books}\:{there}\:{are}\:{C}_{{k}} ^{\mathrm{15}} \:{ways}. \\ $$$${to}\:{place}\:{k}\:{books}\:{there}\:{are}\:{k}!\:{ways}. \\ $$$${to}\:{place}\:\mathrm{15}−{k}\:{books}\:{there}\:{are}\:\left(\mathrm{15}−{k}\right)!\:{ways}. \\ $$$$ \\ $$$${totally}: \\ $$$$\underset{{k}=\mathrm{1}} {\overset{\mathrm{14}} {\sum}}{C}_{{k}} ^{\mathrm{15}} {k}!\left(\mathrm{15}−{k}\right)! \\ $$$$=\underset{{k}=\mathrm{1}} {\overset{\mathrm{14}} {\sum}}\mathrm{15}! \\ $$$$=\mathrm{14}×\mathrm{15}! \\ $$
Commented by I want to learn more last updated on 30/Sep/20

$$\mathrm{Thanks}\:\mathrm{sir},\:\mathrm{but}\:\mathrm{the}\:\mathrm{text}\:\mathrm{answer}\:\mathrm{says}\:\:\:\mathrm{14}\:×\:\mathrm{15}! \\ $$
Commented by mr W last updated on 30/Sep/20
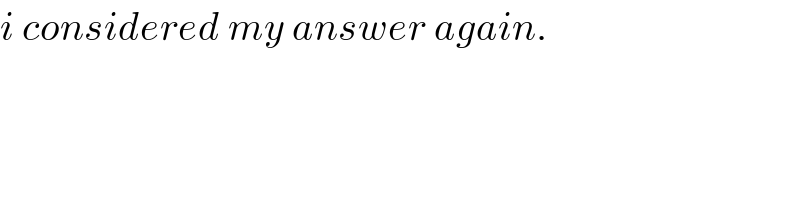
$${i}\:{considered}\:{my}\:{answer}\:{again}.\: \\ $$
Commented by I want to learn more last updated on 01/Oct/20

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir}\:\mathrm{for}\:\mathrm{your}\:\mathrm{time} \\ $$
