Question-115988

Question Number 115988 by bemath last updated on 30/Sep/20

Commented by mr W last updated on 01/Oct/20

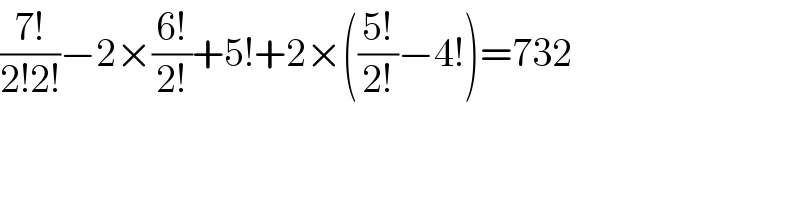
((7!)/(2!2!))−2×((6!)/(2!))+5!+2×(((5!)/(2!))−4!)=732

$$\frac{\mathrm{7}!}{\mathrm{2}!\mathrm{2}!}−\mathrm{2}×\frac{\mathrm{6}!}{\mathrm{2}!}+\mathrm{5}!+\mathrm{2}×\left(\frac{\mathrm{5}!}{\mathrm{2}!}−\mathrm{4}!\right)=\mathrm{732} \\ $$

Commented by bemath last updated on 01/Oct/20

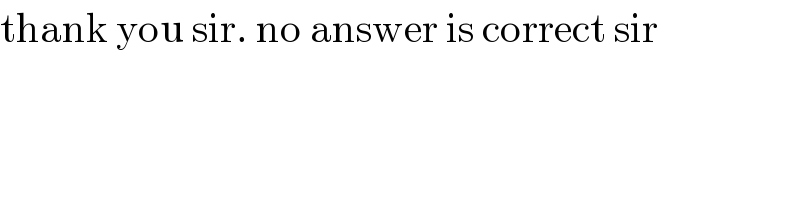
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{no}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{correct}\:\mathrm{sir} \\ $$

Answered by mr W last updated on 01/Oct/20

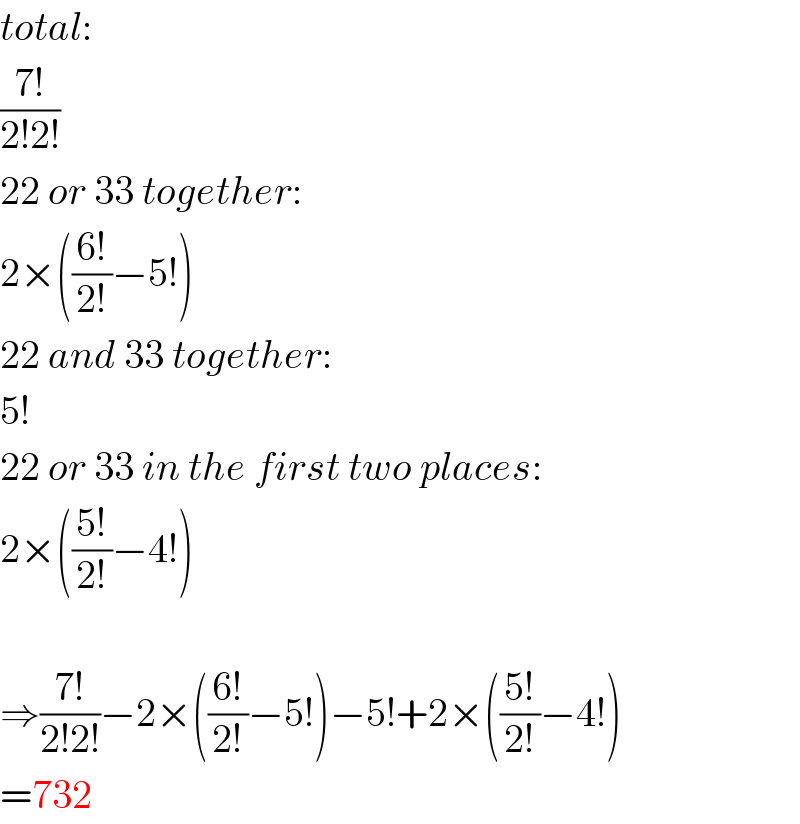
total: ((7!)/(2!2!)) 22 or 33 together: 2×(((6!)/(2!))−5!) 22 and 33 together: 5! 22 or 33 in the first two places: 2×(((5!)/(2!))−4!) ⇒((7!)/(2!2!))−2×(((6!)/(2!))−5!)−5!+2×(((5!)/(2!))−4!) =732

$${total}: \\ $$$$\frac{\mathrm{7}!}{\mathrm{2}!\mathrm{2}!} \\ $$$$\mathrm{22}\:{or}\:\mathrm{33}\:{together}: \\ $$$$\mathrm{2}×\left(\frac{\mathrm{6}!}{\mathrm{2}!}−\mathrm{5}!\right) \\ $$$$\mathrm{22}\:{and}\:\mathrm{33}\:{together}: \\ $$$$\mathrm{5}! \\ $$$$\mathrm{22}\:{or}\:\mathrm{33}\:{in}\:{the}\:{first}\:{two}\:{places}: \\ $$$$\mathrm{2}×\left(\frac{\mathrm{5}!}{\mathrm{2}!}−\mathrm{4}!\right) \\ $$$$ \\ $$$$\Rightarrow\frac{\mathrm{7}!}{\mathrm{2}!\mathrm{2}!}−\mathrm{2}×\left(\frac{\mathrm{6}!}{\mathrm{2}!}−\mathrm{5}!\right)−\mathrm{5}!+\mathrm{2}×\left(\frac{\mathrm{5}!}{\mathrm{2}!}−\mathrm{4}!\right) \\ $$$$=\mathrm{732} \\ $$

Commented by bemath last updated on 02/Oct/20

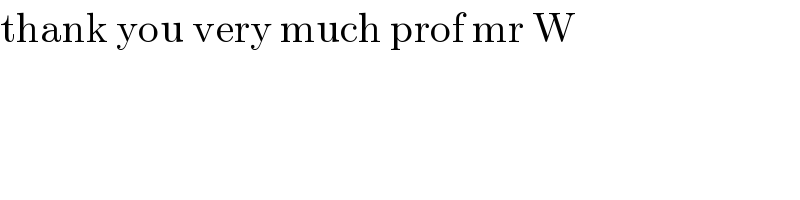
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{prof}\:\mathrm{mr}\:\mathrm{W} \\ $$

Facebook Tweet Pin

Question-115988

Leave a Reply Cancel reply