Question-118555

Question Number 118555 by bramlexs22 last updated on 18/Oct/20

Answered by benjo_mathlover last updated on 18/Oct/20

Start with the 4 non−red slots, then we have ((5),(2) ) ways in which we can assigned of inserting the red slots to get a sequence of 8 categorised slots where the red slots are all apart . So the number of ways is ((5),(2) )×4!×4! = 5760 ways

$${Start}\:{with}\:{the}\:\mathrm{4}\:{non}−{red}\:{slots},\:{then}\:{we} \\ $$$${have}\:\begin{pmatrix}{\mathrm{5}}\\{\mathrm{2}}\end{pmatrix}\:{ways}\:{in}\:{which}\:{we}\:{can}\:{assigned}\:{of} \\ $$$${inserting}\:{the}\:{red}\:{slots}\:{to}\:{get}\:{a}\:{sequence}\:{of} \\ $$$$\mathrm{8}\:{categorised}\:{slots}\:{where}\:{the}\:{red}\:{slots}\: \\ $$$${are}\:{all}\:{apart}\:.\:{So}\:{the}\:{number}\:{of}\:{ways}\:{is}\: \\ $$$$\begin{pmatrix}{\mathrm{5}}\\{\mathrm{2}}\end{pmatrix}×\mathrm{4}!×\mathrm{4}!\:=\:\mathrm{5760}\:{ways} \\ $$

Answered by mr W last updated on 18/Oct/20

⊠■■■■■■■⊠ ■ place for one red book ■ place for one or more other books ⊠ place for zero or more other books (1+x+x^2 +...)^2 (x+x^2 +...)^3 =(x^3 /((1−x)^5 ))=x^3 Σ_(k=0) ^∞ C_4 ^(k+4) x^k coef. of x^4 is C_4 ^5 =C_1 ^5 =5 ⇒number of ways =5×4!×4!=2880

$$\boxtimes\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\boxtimes \\ $$$$\blacksquare\:{place}\:{for}\:{one}\:{red}\:{book} \\ $$$$\blacksquare\:{place}\:{for}\:{one}\:{or}\:{more}\:{other}\:{books} \\ $$$$\boxtimes\:{place}\:{for}\:{zero}\:{or}\:{more}\:{other}\:{books} \\ $$$$ \\ $$$$\left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +…\right)^{\mathrm{2}} \left({x}+{x}^{\mathrm{2}} +…\right)^{\mathrm{3}} \\ $$$$=\frac{{x}^{\mathrm{3}} }{\left(\mathrm{1}−{x}\right)^{\mathrm{5}} }={x}^{\mathrm{3}} \underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}{C}_{\mathrm{4}} ^{{k}+\mathrm{4}} {x}^{{k}} \\ $$$${coef}.\:{of}\:{x}^{\mathrm{4}} \:{is}\:{C}_{\mathrm{4}} ^{\mathrm{5}} ={C}_{\mathrm{1}} ^{\mathrm{5}} =\mathrm{5} \\ $$$$\Rightarrow{number}\:{of}\:{ways}\:=\mathrm{5}×\mathrm{4}!×\mathrm{4}!=\mathrm{2880} \\ $$

Commented by prakash jain last updated on 18/Oct/20

This can result in a blank space. ^5 C_4 is choosing 4 out of 5 blanks □■□■□■□■□ and it can result in choosing Xs below X■X■□■X■X Generating Function Approach □■□■■■■■■ The arrangement above will also result in same function. □■■■■■■■□ Since 3 books slot cannot be chosen we only need to find coefficient on x^1 from 2 white spaces. (1−x)^(−2) =2

$$\mathrm{This}\:\mathrm{can}\:\mathrm{result}\:\mathrm{in}\:\mathrm{a}\:\mathrm{blank}\:\mathrm{space}.\: \\ $$$$\:^{\mathrm{5}} {C}_{\mathrm{4}} \\ $$$$\mathrm{is}\:\mathrm{choosing}\:\mathrm{4}\:\mathrm{out}\:\mathrm{of}\:\mathrm{5}\:\mathrm{blanks} \\ $$$$\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box\blacksquare\Box \\ $$$$\mathrm{and}\:\mathrm{it}\:\mathrm{can}\:\mathrm{result}\:\mathrm{in}\:\mathrm{choosing}\:\mathrm{X}{s}\:\mathrm{below} \\ $$$$\mathrm{X}\blacksquare\mathrm{X}\blacksquare\Box\blacksquare\mathrm{X}\blacksquare\mathrm{X} \\ $$$$\boldsymbol{\mathrm{Generating}}\:\boldsymbol{\mathrm{Function}}\:\boldsymbol{\mathrm{Approach}} \\ $$$$\Box\blacksquare\Box\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare \\ $$$$\mathrm{The}\:\mathrm{arrangement}\:\mathrm{above}\:\mathrm{will}\:\mathrm{also} \\ $$$$\mathrm{result}\:\mathrm{in}\:\mathrm{same}\:\mathrm{function}. \\ $$$$\Box\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\Box \\ $$$$\mathrm{Since}\:\mathrm{3}\:\mathrm{books}\:\mathrm{slot}\:\mathrm{cannot}\:\mathrm{be}\:\mathrm{chosen} \\ $$$$\mathrm{we}\:\mathrm{only}\:\mathrm{need}\:\mathrm{to}\:\mathrm{find}\:\mathrm{coefficient}\:\mathrm{on}\:{x}^{\mathrm{1}} \\ $$$$\mathrm{from}\:\mathrm{2}\:\mathrm{white}\:\mathrm{spaces}. \\ $$$$\left(\mathrm{1}−{x}\right)^{−\mathrm{2}} \:\:=\mathrm{2} \\ $$

Commented by mr W last updated on 18/Oct/20

if the question were: 4 red books and 8 other books, then the answer is C_4 ^9 ×4!×8!. in such a case the generating function method is the best approach i think.

$${if}\:{the}\:{question}\:{were}:\:\mathrm{4}\:{red}\:{books}\:{and} \\ $$$$\mathrm{8}\:{other}\:{books},\:{then}\:{the}\:{answer}\:{is} \\ $$$${C}_{\mathrm{4}} ^{\mathrm{9}} ×\mathrm{4}!×\mathrm{8}!. \\ $$$${in}\:{such}\:{a}\:{case}\:{the}\:{generating}\:{function} \\ $$$${method}\:{is}\:{the}\:{best}\:{approach}\:{i}\:{think}. \\ $$

Commented by prakash jain last updated on 18/Oct/20

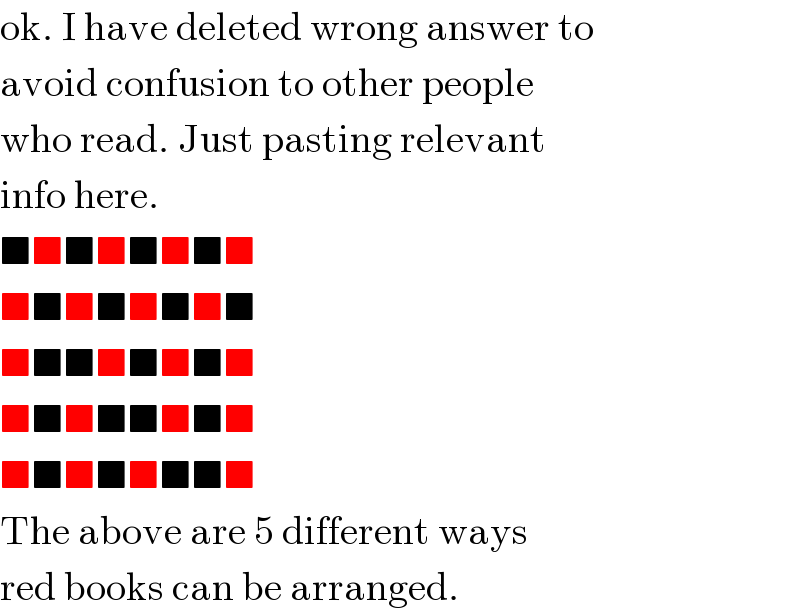
ok. I have deleted wrong answer to avoid confusion to other people who read. Just pasting relevant info here. ■■■■■■■■ ■■■■■■■■ ■■■■■■■■ ■■■■■■■■ ■■■■■■■■ The above are 5 different ways red books can be arranged.

$$\mathrm{ok}.\:\mathrm{I}\:\mathrm{have}\:\mathrm{deleted}\:\mathrm{wrong}\:\mathrm{answer}\:\mathrm{to} \\ $$$$\mathrm{avoid}\:\mathrm{confusion}\:\mathrm{to}\:\mathrm{other}\:\mathrm{people} \\ $$$$\mathrm{who}\:\mathrm{read}.\:\mathrm{Just}\:\mathrm{pasting}\:\mathrm{relevant} \\ $$$$\mathrm{info}\:\mathrm{here}. \\ $$$$\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare \\ $$$$\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare \\ $$$$\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare \\ $$$$\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare \\ $$$$\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare\blacksquare \\ $$$$\mathrm{The}\:\mathrm{above}\:\mathrm{are}\:\mathrm{5}\:\mathrm{different}\:\mathrm{ways} \\ $$$$\mathrm{red}\:\mathrm{books}\:\mathrm{can}\:\mathrm{be}\:\mathrm{arranged}. \\ $$

Commented by mr W last updated on 18/Oct/20

$${absolutely}\:{right}\:{sir}!\:{thanks}\:{alot}! \\ $$

Commented by bramlexs22 last updated on 19/Oct/20

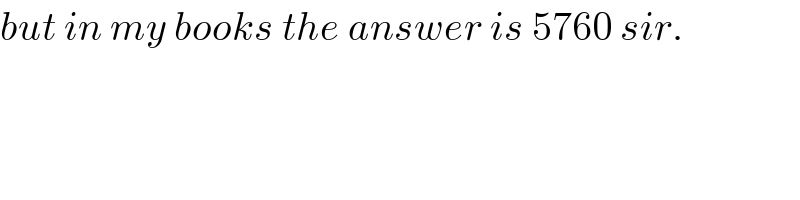
$${but}\:{in}\:{my}\:{books}\:{the}\:{answer}\:{is}\:\mathrm{5760}\:{sir}. \\ $$$$ \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply