Question Number 118944 by mathdave last updated on 20/Oct/20

Answered by Dwaipayan Shikari last updated on 21/Oct/20
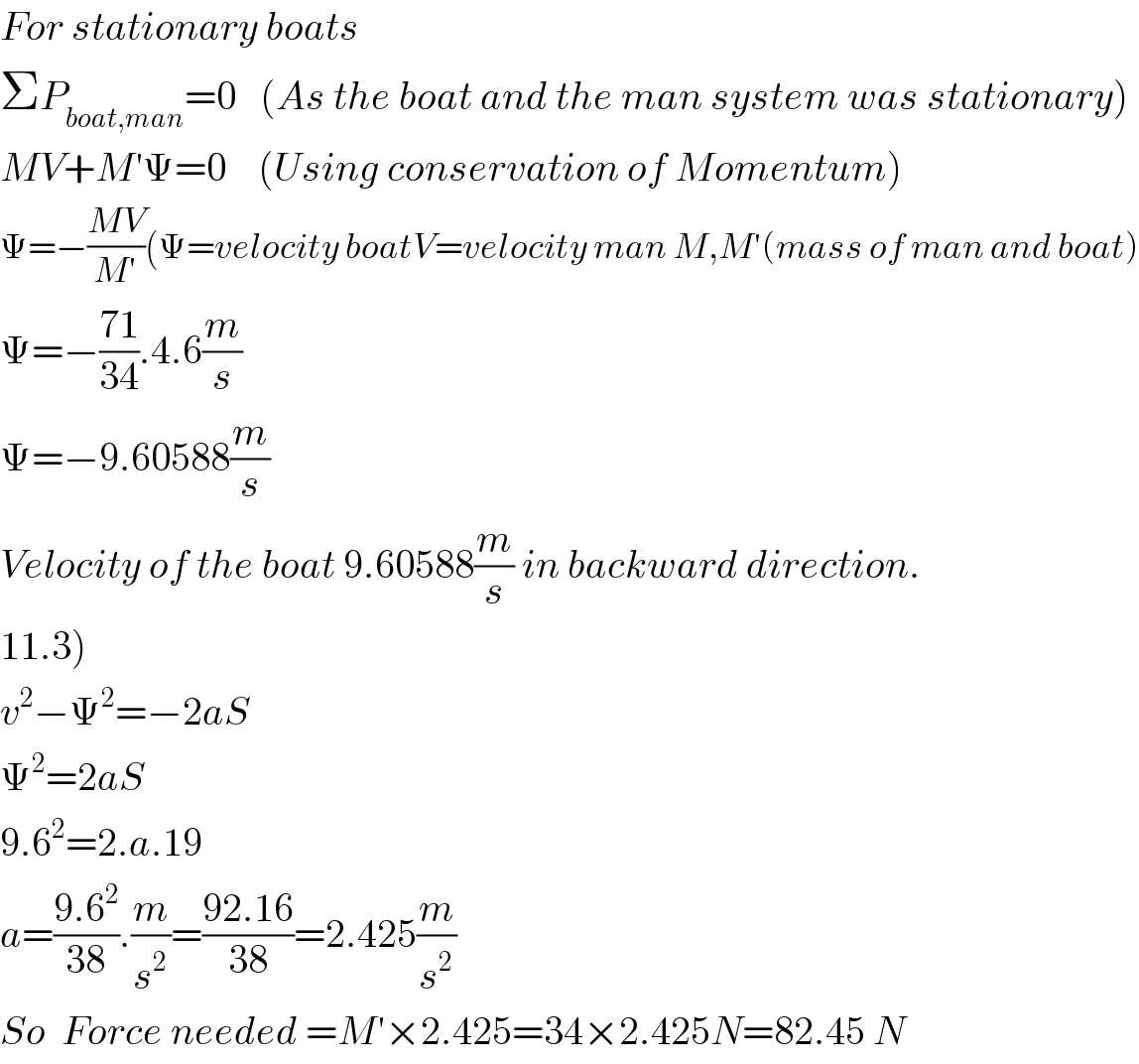
$${For}\:{stationary}\:{boats}\: \\ $$$$\Sigma{P}_{{boat},{man}} =\mathrm{0}\:\:\:\left({As}\:{the}\:{boat}\:{and}\:{the}\:{man}\:{system}\:{was}\:{stationary}\right) \\ $$$${MV}+{M}'\Psi=\mathrm{0}\:\:\:\:\left({Using}\:{conservation}\:{of}\:{Momentum}\right) \\ $$$$\Psi=−\frac{{MV}}{{M}'}\left(\Psi={velocity}\:{boatV}={velocity}\:{man}\:{M},{M}'\left({mass}\:{of}\:{man}\:{and}\:{boat}\right)\right. \\ $$$$\Psi=−\frac{\mathrm{71}}{\mathrm{34}}.\mathrm{4}.\mathrm{6}\frac{{m}}{{s}} \\ $$$$\Psi=−\mathrm{9}.\mathrm{60588}\frac{{m}}{{s}} \\ $$$${Velocity}\:{of}\:{the}\:{boat}\:\mathrm{9}.\mathrm{60588}\frac{{m}}{{s}}\:{in}\:{backward}\:{direction}. \\ $$$$\left.\mathrm{11}.\mathrm{3}\right) \\ $$$${v}^{\mathrm{2}} −\Psi^{\mathrm{2}} =−\mathrm{2}{aS} \\ $$$$\Psi^{\mathrm{2}} =\mathrm{2}{aS} \\ $$$$\mathrm{9}.\mathrm{6}^{\mathrm{2}} =\mathrm{2}.{a}.\mathrm{19} \\ $$$${a}=\frac{\mathrm{9}.\mathrm{6}^{\mathrm{2}} }{\mathrm{38}}.\frac{{m}}{{s}^{\mathrm{2}} }=\frac{\mathrm{92}.\mathrm{16}}{\mathrm{38}}=\mathrm{2}.\mathrm{425}\frac{{m}}{{s}^{\mathrm{2}} } \\ $$$${So}\:\:{Force}\:{needed}\:={M}'×\mathrm{2}.\mathrm{425}=\mathrm{34}×\mathrm{2}.\mathrm{425}{N}=\mathrm{82}.\mathrm{45}\:{N} \\ $$
