Question Number 119314 by peter frank last updated on 23/Oct/20
Commented by mr W last updated on 23/Oct/20

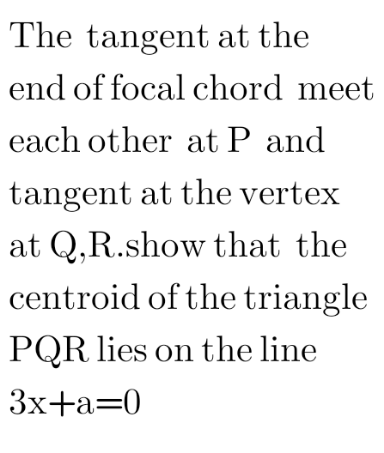
$${what}\:{is}\:{a}? \\ $$
Commented by mr W last updated on 23/Oct/20
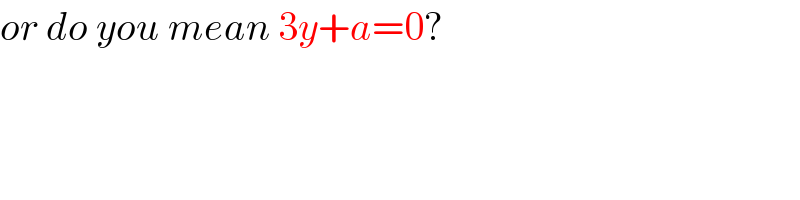
$${or}\:{do}\:{you}\:{mean}\:\mathrm{3}{y}+{a}=\mathrm{0}? \\ $$
Commented by peter frank last updated on 23/Oct/20

$$\mathrm{costant} \\ $$
Commented by mr W last updated on 23/Oct/20
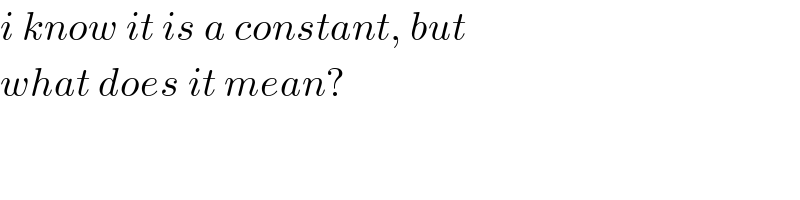
$${i}\:{know}\:{it}\:{is}\:{a}\:{constant},\:{but} \\ $$$${what}\:{does}\:{it}\:{mean}? \\ $$
Commented by peter frank last updated on 23/Oct/20

$$\mathrm{I}\:\:\mathrm{dont}\:\mathrm{know}\:\mathrm{sir}\: \\ $$
Answered by mr W last updated on 23/Oct/20

Commented by mr W last updated on 23/Oct/20
![say vertex is the origin, the facus is F(0,h), then the eqn. of parabola is y=(x^2 /(4h)) say the focal chord is y=h+mx with m=tan θ intersection with parabola: (x^2 /(4h))=h+mx x^2 −4mhx−4h^2 =0 ⇒x=2h(m±(√(m^2 +1))) end point A: x_A =2h(m−(√(m^2 +1))) y_A =h+2hm(m−(√(m^2 +1))) tangent at A: (dy/dx)=(x/(2h))=m−(√(m^2 +1)) y=h+2hm(m−(√(m^2 +1)))+(m−(√(m^2 +1)))[x−2h(m−(√(m^2 +1)))] 0=h+2hm(m−(√(m^2 +1)))+(m−(√(m^2 +1)))[x−2h(m−(√(m^2 +1)))] ⇒x=x_Q =−(h/(m−(√(m^2 +1))))−2h(√(m^2 +1)) end point B: x_B =2h(m+(√(m^2 +1))) y_B =h+2hm(m+(√(m^2 +1))) tangent at B: y=h+2hm(m+(√(m^2 +1)))+(m+(√(m^2 +1)))[x−2h(m+(√(m^2 +1)))] 0=h+2hm(m+(√(m^2 +1)))+(m+(√(m^2 +1)))[x−2h(m+(√(m^2 +1)))] ⇒x=x_R =−(h/(m+(√(m^2 +1))))+2h(√(m^2 +1)) intersection point P: y=h+2hm(m−(√(m^2 +1)))+(m−(√(m^2 +1)))[x−2h(m−(√(m^2 +1)))] y=h+2hm(m+(√(m^2 +1)))+(m+(√(m^2 +1)))[x−2h(m+(√(m^2 +1)))] h[(m+(√(m^2 +1)))^2 −(m−(√(m^2 +1)))^2 ]=(2hm+x)(√(m^2 +1)) ⇒x_P =2mh ⇒y_P =−h centroid G: y_G =(1/3)y_P =−(h/3)=constant ⇒3y_G +h=0 or 3y+h=0 x_G =(1/3)(x_P +x_Q +x_R ) =(1/3)(2mh−(h/(m−(√(m^2 +1))))−2h(√(m^2 +1))−(h/(m+(√(m^2 +1))))+2h(√(m^2 +1))) =(h/3)(2m+(1/( (√(m^2 +1))−m))−(1/( (√(m^2 +1))+m))) =((4mh)/3)≠constant](https://www.tinkutara.com/question/Q119346.png)
$${say}\:{vertex}\:{is}\:{the}\:{origin},\:{the}\:{facus}\:{is} \\ $$$${F}\left(\mathrm{0},{h}\right),\:{then}\:{the}\:{eqn}.\:{of}\:{parabola}\:{is} \\ $$$${y}=\frac{{x}^{\mathrm{2}} }{\mathrm{4}{h}} \\ $$$${say}\:{the}\:{focal}\:{chord}\:{is} \\ $$$${y}={h}+{mx}\:{with}\:{m}=\mathrm{tan}\:\theta \\ $$$$ \\ $$$${intersection}\:{with}\:{parabola}: \\ $$$$\frac{{x}^{\mathrm{2}} }{\mathrm{4}{h}}={h}+{mx} \\ $$$${x}^{\mathrm{2}} −\mathrm{4}{mhx}−\mathrm{4}{h}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow{x}=\mathrm{2}{h}\left({m}\pm\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$$ \\ $$$${end}\:{point}\:{A}: \\ $$$${x}_{{A}} =\mathrm{2}{h}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$${y}_{{A}} ={h}+\mathrm{2}{hm}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$${tangent}\:{at}\:{A}: \\ $$$$\frac{{dy}}{{dx}}=\frac{{x}}{\mathrm{2}{h}}={m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}} \\ $$$${y}={h}+\mathrm{2}{hm}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)+\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\left[{x}−\mathrm{2}{h}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\right] \\ $$$$\mathrm{0}={h}+\mathrm{2}{hm}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)+\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\left[{x}−\mathrm{2}{h}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\right] \\ $$$$\Rightarrow{x}={x}_{{Q}} =−\frac{{h}}{{m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}}−\mathrm{2}{h}\sqrt{{m}^{\mathrm{2}} +\mathrm{1}} \\ $$$$ \\ $$$${end}\:{point}\:{B}: \\ $$$${x}_{{B}} =\mathrm{2}{h}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$${y}_{{B}} ={h}+\mathrm{2}{hm}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$${tangent}\:{at}\:{B}: \\ $$$${y}={h}+\mathrm{2}{hm}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)+\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\left[{x}−\mathrm{2}{h}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\right] \\ $$$$\mathrm{0}={h}+\mathrm{2}{hm}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)+\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\left[{x}−\mathrm{2}{h}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\right] \\ $$$$\Rightarrow{x}={x}_{{R}} =−\frac{{h}}{{m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}}+\mathrm{2}{h}\sqrt{{m}^{\mathrm{2}} +\mathrm{1}} \\ $$$$ \\ $$$${intersection}\:{point}\:{P}: \\ $$$${y}={h}+\mathrm{2}{hm}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)+\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\left[{x}−\mathrm{2}{h}\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\right] \\ $$$${y}={h}+\mathrm{2}{hm}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)+\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\left[{x}−\mathrm{2}{h}\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)\right] \\ $$$${h}\left[\left({m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)^{\mathrm{2}} −\left({m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right)^{\mathrm{2}} \right]=\left(\mathrm{2}{hm}+{x}\right)\sqrt{{m}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\Rightarrow{x}_{{P}} =\mathrm{2}{mh} \\ $$$$\Rightarrow{y}_{{P}} =−{h} \\ $$$$ \\ $$$${centroid}\:{G}: \\ $$$${y}_{{G}} =\frac{\mathrm{1}}{\mathrm{3}}{y}_{{P}} =−\frac{{h}}{\mathrm{3}}={constant} \\ $$$$\Rightarrow\mathrm{3}{y}_{{G}} +{h}=\mathrm{0}\:{or}\:\mathrm{3}{y}+{h}=\mathrm{0} \\ $$$${x}_{{G}} =\frac{\mathrm{1}}{\mathrm{3}}\left({x}_{{P}} +{x}_{{Q}} +{x}_{{R}} \right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{2}{mh}−\frac{{h}}{{m}−\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}}−\mathrm{2}{h}\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}−\frac{{h}}{{m}+\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}}+\mathrm{2}{h}\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$$=\frac{{h}}{\mathrm{3}}\left(\mathrm{2}{m}+\frac{\mathrm{1}}{\:\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}−{m}}−\frac{\mathrm{1}}{\:\sqrt{{m}^{\mathrm{2}} +\mathrm{1}}+{m}}\right) \\ $$$$=\frac{\mathrm{4}{mh}}{\mathrm{3}}\neq{constant} \\ $$
Commented by peter frank last updated on 24/Oct/20

$$\mathrm{thank}\:\mathrm{you}.\mathrm{understood}\:\mathrm{every}\:\mathrm{line} \\ $$$$ \\ $$
